When you have learned to differentiate trigonometric functions, try to prove that in the relativistic case the formula F = ma does not hold, even if m is taken to be the relativistic mass.
Consider the case of a constant force acting on the object. In this was the expression
F=mam0√1−v2/c2dvdt
reduces to the expression
a=dvdt=b√1−v2c2
where dvdt is the time derivative of the velocity, b=Fm0 is a constant.
It may easily be seen that the equation for the derivative becomes an identity, if one puts v=csin(bt)c. Indeed in this case dvdt=bcos(bt),√1−v2/c2=cos(bt)c, consequently dvdt=b√1−v2/c2 But the result obtained for the velocity is meaningless since after a finite time interval
t=πc2b=πem02F
the velocity of the object will be equal to the velocity of light in a vacuum, and this is in contradiction with the theory of relativity.
Similar Questions
Knowledge Check
Two containers of sand S and H are arranged like the blocks figure (a) The container alone have negligible mass, the sand in them has a total mass Mtot , the sand in the hanging container H has mass m. You are to measure the magnitude a of the acceleration of the system in a series of experiments where m varies from experiment to experiment by Mtot does not, that is, you will shift sand between the containers before each trial mMtot is taken on the horizontal axis for all the plots.
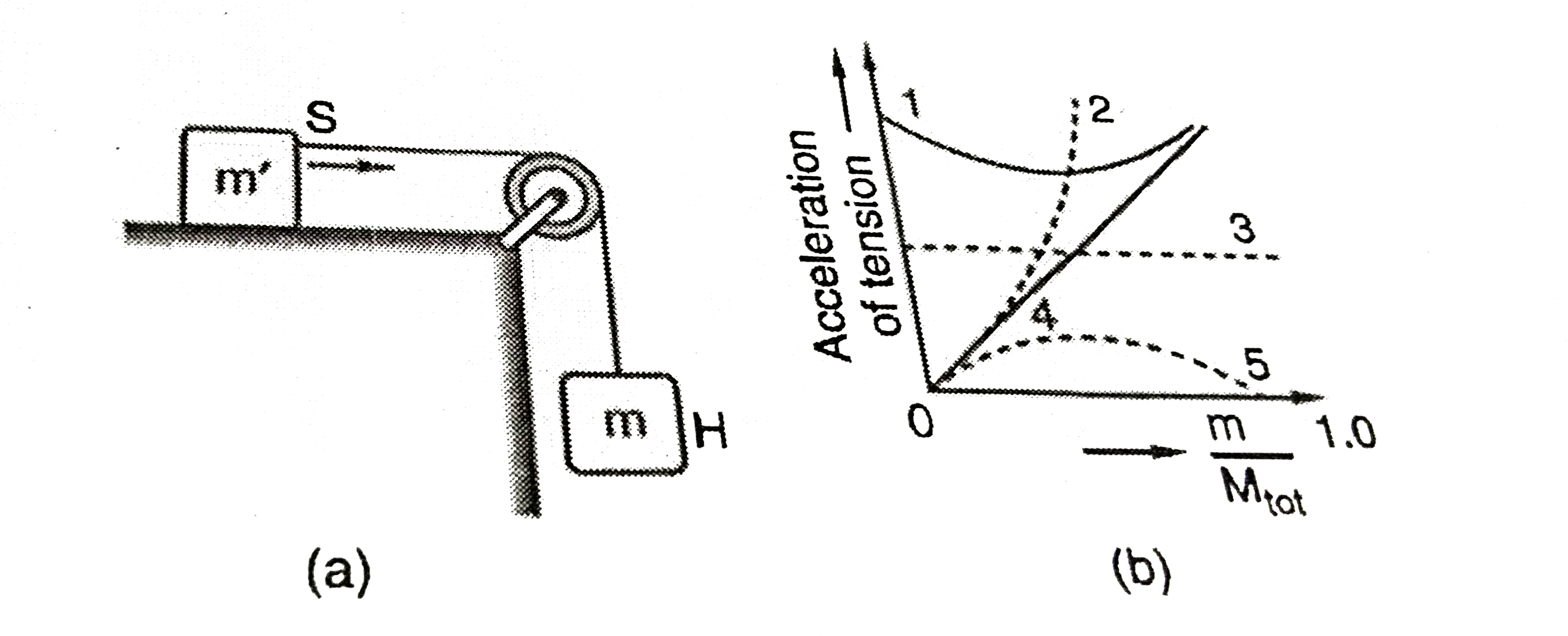
The curve which gives tension in the connecting string (taken on y-axis) as a function of ratio (mMtot) is:A1B2C4D5There are two ways in which, 'nonasserters' manage a conflict. Sometimes they ignore their needs. Faced with a constantly barking dog, for instance, a nonassertive person would try to forget the barking by closing the windows and trying to concentrate even harder. Another form of denial would be to claim that no problem exists-that a little barking never bothered anyone. To the degree that it's possible to make problems disappear by ignoring them, such an approach is probably advisable. In many cases, however, it simply isn't realistic to claim that nothing is wrong. For instance, if your health is beingjeopardized by the cigarette smoke from someone nearby, you are clearly punishing yourself by remaining silent. If you need to learn more information from a supervisor before undertaking a project, you reduce the quality ofyourworkby pretending that you understand it at all. If you claim that an unsatisfactory repair job is acceptable, you are paying good money for nothing. In all these and many more cases simply pretending that nothing is the matter when your needs continue to go unmet is clearly not the answer.
A second nonassertive course of action is to acknowledge your needs are not being met but simply to accept the situation, hoping that it might clear up without any action on your part. You could, for instance, wait for the neighbour who owns the barking dog to move. Y ciu could wait for the dog to be run over by passing car or to die of old age. You could hope that your neighbour will realize how noisy the dog is and do something to keep it quiet. Each of these occurrences is a possibility, of course, but it would be unrealistic to count on one of them to solve your problem. And even if by chance you were lucky enough forthe dog problem to solve without taking action, you couldn't expect to be so fortunate in other parts of your life.
In addition, while waiting for one of these eventualities, you would undoubtedly grow more and more angry at your neighbour, making a friendly relationship between the two of you impossible. You would also lose a degree of selfrespect, since you would see yourself as the kind of person who can't cope with even a common everyday irritation. Clearly, non-ssertion is not a very satisfying course of action either in this case or in other instances.
In case of the presence of a barking dog in the neighbourhood, a nonassertive person is likely to :APray to god to eliminate the dog for good.BSpeak to the neighbour who owns the dog.CClose the window or behave as if the dog is not there.DGet someone to keep the dog quiet.
When you have learned to integrate, try to derive a formula for the instantaneous current from a capacitor discharged through a resistor.
View SolutionIn a nuclear physics experiment, electrons are to be accelerated so as to have de-Broglie wavelength of the order of the diameter of a heavy nucleus (~10–14m). Determine the momentum of the electron needed to make the wavelength of electrons equal to diameter. Use the classical formula for momentum, p=m0v to determine the speed of electrons. Is this speed permissible? Now use the relativistic mass of the electrons in the expression of momentum to find the speed of electrons. Relativistic mass is given by m=m0√1−v2/c2.
Where m0 is rest mass and v is speed of the particle.
View SolutionWhen you have learned to integrate, try to analyze the process of shorting a circuit made up of a coil and a resistor connected to a power supply with constant e.m.f., i.e. the dependence of the current on time. Assume the coil to be without a ferromagnetic core.
View SolutionWhen you have learned to integrate, try to analyze the process of shorting a circuit made up of a coil and a resistor connected to a power supply with constant e.m.f., i.e. the dependence of the current on time. Assume the coil to be without a ferromagnetic core.
View SolutionWe have obtained the expression for the potential of the field of a point charge using numerical methods. When you have learned to difierentiate, prove that formula (18.25) leads to the expression for the field intensity of a point charge known from the Coulomb law.
View SolutionIn 18.3 and 18.7, 18.8 we have obtained the expression for the potential of the field of a point charge using numerical methods. When you have learned to difierentiate, prove that formula (18.25) leads to the expression for the field intensity of a point charge known from the Coulomb law.
View Solution
