What is the angular velocity of rotation of a star at which the matter starts to escape from its equator? In calculating make use of a reference frame fixed to the rotating star. Compare with Problem.
The correct Answer is:ω≥√γM/R3
The forces acting on material particles on the star.s equator in the rotating reference frame are the force of gravity F=γmMR2 and the centrifugal force of inertia Ict=mω2R Matter will start to escape, if Ict≥F, i.e. ω≥√γM/R3
Similar Questions
Knowledge Check
The angular velocity of rotation of star (of mass M and radius R ) at which the matter start to escape from its equator will be
A√2GM2RB√2GMgC√2GMR2D√2GRMThe angular velocity of rotation of a planet of mass M and radius R, at which the matter start to escape from its equator is
A√2GMR3B√2GMRC√2G2MRD√2GM2R
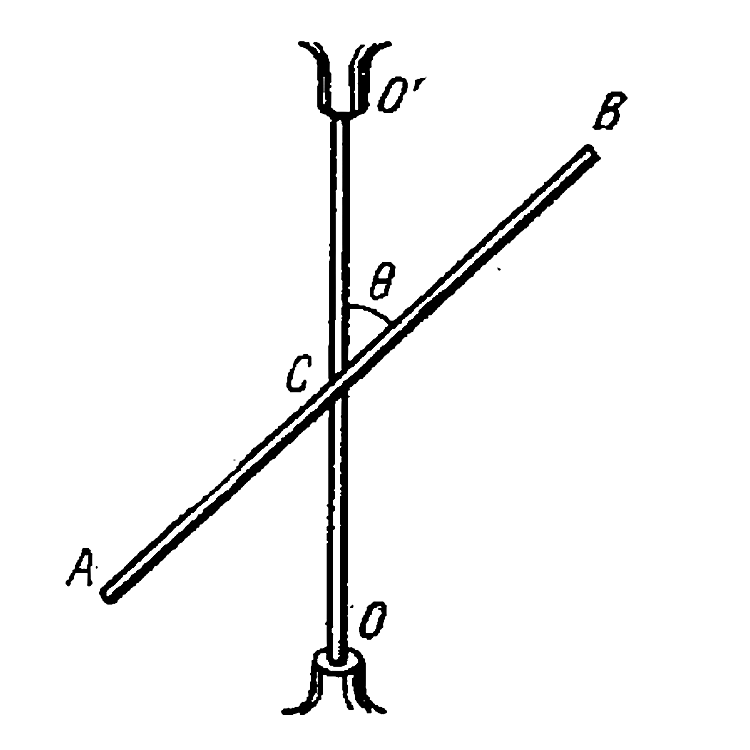
A midpoint of a thin uniform rod AB of mass m and length l is rigidly fixed to a rotation axle OO′ as shown in figure. The rod is set into rotation with a constant angular velocity ω. Find the resultant moment of the centrifugal forces of inertia relative to the point C in the reference frame fixed to the axle OO′ and to the end.
View SolutionCalculate the radius of the circular orbit of a stationary Earth's satellite, which remains motionless with respect to its surface. What are its velocity and acceleration in the inertial reference frame fixed at a given moment to the centre of the Earth?
View SolutionBinary stars rotate under mutual gravitational force at separation 2(Gω2)13, where ω is the angular velocity of each of the star about centre of mass of the system. If difference between the mass of stars is 6 units. Find the ratio of mass of bigger star to smaller star.
View SolutionBinary stars rotate under mutual gravitational force at separation 2(Gω2)13, where ω is the angular velocity of each of the star about centre of mass of the system. If difference between the mass of stars is 6 units. Find the ratio of mass of bigger star to smaller star.
View SolutionA horizontal smooth rod AB rotates with a constant angular velocity ω=2.00rad/s about a vertical axis passing through its end A. A freely sliding sleeve of mass m=0.50g moves along the rod from the point A with the initial velocity v0=1.00m/s. Find the Coriolis force acting on the sleeve (in the reference frame fixed to rotating rod) at the moment when the sleeve is located at the distance r=50cm from the rotation axis.
View SolutionAt what angular speed of rotation is the surface material on the equator of a neutron star on the verge of flying off the star if the star is spherical with a radius of 18.0 km and a mass of 7.72×1024 kg?
View Solution
