Find the value of the constant k so that the function given below is continuous at x=0 ,f(x)={(,1−cos2x2x2(x≠0),kx=0) .
The correct Answer is:k=-4
To find the value of the constant k so that the function
f(x)={1−cos(2x)2x2if x≠0kif x=0
is continuous at x=0, we need to ensure that the limit of f(x) as x approaches 0 is equal to f(0)=k.
Step 1: Find the limit of f(x) as x approaches 0
We need to calculate:
limx→0f(x)=limx→01−cos(2x)2x2
Step 2: Use the trigonometric identity
Using the identity 1−cos(2x)=2sin2(x), we can rewrite the limit:
limx→01−cos(2x)2x2=limx→02sin2(x)2x2=limx→0sin2(x)x2
Step 3: Simplify the limit
The expression simplifies to:
limx→0(sin(x)x)2
Step 4: Evaluate the limit
We know that:
limx→0sin(x)x=1
Thus:
limx→0(sin(x)x)2=12=1
Step 5: Set the limit equal to k
For the function to be continuous at x=0, we need:
k=limx→0f(x)=1
Final Answer
The value of the constant k is:
1
Topper's Solved these Questions
- QUESTION PAPER-2018BOOK - ICSECHAPTER - QUESTION PAPER-2018EXERCISE - Section -B
8 Videos
- QUESTION PAPER-2018BOOK - ICSECHAPTER - QUESTION PAPER-2018EXERCISE - Section -C
8 Videos
- QUESTION PAPER 2022 TERM 1BOOK - ICSECHAPTER - QUESTION PAPER 2022 TERM 1EXERCISE - SECTION C
8 Videos
- RELATIONS AND FUNCTIONS BOOK - ICSECHAPTER - RELATIONS AND FUNCTIONS EXERCISE - MULTIPLE CHOICE QUESTIONS (Competency based questions)
20 Videos
Similar Questions
Find λ if the scalar projection of →a=λˆi+ˆj+4ˆk on →b=2ˆi+6ˆj+3ˆk is 4 units.
View SolutionGiven the total cost function for x units of commodity as C(x)=13x3+3x2−16x+2 . Find Marginal cost function .
View SolutionThe cost function of a firm is given by C(x)=1500+25x+x210 . Then the marginal cost of the firm MC(x) will be :
View SolutionThe price of the oranges in the market is dependent on the amount of oranges (in kgs) which can be represented as y = 3x + 5. Reema went to buy the oranges for a family function in her house. The total number of oranges she wants to buys is 5≤x≤10 according to her assumption of people coming to the party. Answer the following questions on the basis of the given information.
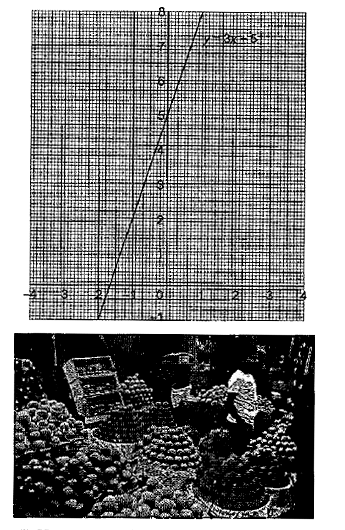
How many ordered pairs can be represented for the equation y=3x+5 for ≤x≤x10 ?
View SolutionDetermine the value of the constant k, so that the function f(x)={(kx)/(|x|), if x =0 is continuous at x=0
View SolutionFind the value of the constant k so that the function given below is continuous at x=0 . f(x)={1−cos2x2x2, x≠0 k, x=0
View SolutionIf the functions f(x) , defined below is continuous at x=0 , find the value of k: f(x)={1−cos2x2x2 , x<0k , x=0,x|x| , x>0
View SolutionFor what value of k, the function
f(x)={x|x|+2x2,x≠0k,x=0 is continuous at x = 0 ?
View Solutionयदि फलन f(x)={1−cos2xx2,x≠0k,x=0,x=0 सतत है, तो k का मान है-
View Solutionयदि निम्नलिखित फलन x=0 पर सतत हो, तो k का मान ज्ञात कीजिए:
f(x)=⎧⎪ ⎪ ⎪⎨⎪ ⎪ ⎪⎩1−cos2x2x2यदिx<0kयदिx=0x|x|यदिx>0
View SolutionFind the values (s) of k so that the following function is continuous at x=0 f(x)=⎧⎨⎩1−coskxxsinx if x≠o12Ifx=0
View SolutionDetermine the value of the constant k, so that the function f(x) ={kx|x|, if x<0 ,3 if x≥0 is continuous at x=0
View SolutionFind the value of the constant k so that the function given below is continuous at x=0 . f(x)={1−cos2x2x2, x≠0 k, x=0
View SolutionFind the value of f(0) so that the function given below is continuous at x=0 f(x)=1−cos2x2x2
View SolutionIf the functions f(x) , defined below is continuous at x=0 , find the value of k: f(x)={1−cos2x2x2 , x<0 k, x=0,x|x| , x>0
View SolutionFind the values of k so that the function f is continuous at the indicated point f(x)=⎧⎪⎨⎪⎩1−cos(kx)x2,if x≠012,if x=0 at x= 0
View Solution
