Evaluate: ∫x3+5x2+4x+1x2dx
The correct Answer is:x22+5x+4logx−1x+c
To evaluate the integral
∫x3+5x2+4x+1x2dx,
we can simplify the integrand first.
Step 1: Simplify the integrand
We can divide each term in the numerator by x2:
x3x2+5x2x2+4xx2+1x2=x+5+4x+1x2.
Thus, the integral becomes:
∫(x+5+4x+1x2)dx.
Step 2: Break down the integral
Now we can separate the integral into simpler parts:
∫xdx+∫5dx+∫4xdx+∫1x2dx.
Step 3: Evaluate each integral
1. Integrate x:
∫xdx=x22.
2. Integrate 5:
∫5dx=5x.
3. Integrate 4x:
∫4xdx=4ln|x|.
4. Integrate 1x2:
∫1x2dx=∫x−2dx=−1x.
Step 4: Combine the results
Now, we combine all the results from the integrals:
∫x3+5x2+4x+1x2dx=x22+5x+4ln|x|−1x+C,
where C is the constant of integration.
Final Answer
Thus, the final answer is:
x22+5x+4ln|x|−1x+C.
We can divide each term in the numerator by x2:
Now we can separate the integral into simpler parts:
1. Integrate x:
∫xdx=x22.
∫5dx=5x.
∫4xdx=4ln|x|.
∫1x2dx=∫x−2dx=−1x.
Now, we combine all the results from the integrals:
Thus, the final answer is:
Topper's Solved these Questions
- BOOK - ICSECHAPTER - QUESTION PAPER-2018EXERCISE - Section -B8videos
QUESTION PAPER-2018
- BOOK - ICSECHAPTER - QUESTION PAPER-2018EXERCISE - Section -C8videos
QUESTION PAPER-2018
- BOOK - ICSECHAPTER - QUESTION PAPER 2022 TERM 1EXERCISE - SECTION C8videos
QUESTION PAPER 2022 TERM 1
- BOOK - ICSECHAPTER - RELATIONS AND FUNCTIONS EXERCISE - MULTIPLE CHOICE QUESTIONS (Competency based questions)20videos
RELATIONS AND FUNCTIONS
Similar Questions
Find λ if the scalar projection of →a=λˆi+ˆj+4ˆk on →b=2ˆi+6ˆj+3ˆk is 4 units.
05:48View SolutionGiven the total cost function for x units of commodity as C(x)=13x3+3x2−16x+2 . Find Marginal cost function .
02:27View SolutionThe cost function of a firm is given by C(x)=1500+25x+x210 . Then the marginal cost of the firm MC(x) will be :
09:57View SolutionThe price of the oranges in the market is dependent on the amount of oranges (in kgs) which can be represented as y = 3x + 5. Reema went to buy the oranges for a family function in her house. The total number of oranges she wants to buys is 5≤x≤10 according to her assumption of people coming to the party. Answer the following questions on the basis of the given information.
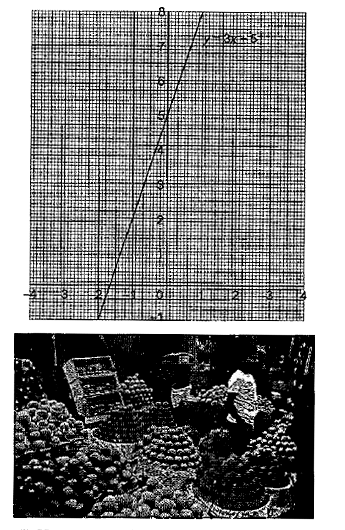
How many ordered pairs can be represented for the equation y=3x+5 for ≤x≤x10 ?03:14View SolutionEvaluate: ∫5x+3√x2+4x+10 dx
05:48View SolutionEvaluate: ∫x3+5x2+4x+1x2 dx
07:02View SolutionEvaluate:
(i) ∫(5x2+2x−5−7x+1√x+5x)dx
(ii) ∫(3sinx−4cosx+5sec2x−2cosec2x)dx
(iii) ∫(1−x)(2+3x)(5−4x)dx
(iv) ∫(3x4−5x3+4x2−x+2x3)dx , (v) ∫(x2+1x2)3dx08:42View SolutionEvaluate : ∫dx√5x+4x−x2
03:51View SolutionEvaluate: ∫5x+3√x2+4x+10 dx
02:00View SolutionEvaluate: ∫5x+3√x2+4x+10 dx
01:53View SolutionEvaluate: ∫x2+5x−8x3−4xdx
06:28View SolutionEVALUATE
∫x3+5x2+4x+1x3dx07:35View SolutionEvaluate: ∫x3+5x2+4x+1x2 dx
03:47View SolutionEvaluate: ∫x−14x2−4x+3 dx
04:07View SolutionEvaluate ∫(5x+3)dxx2+4x+10
06:40View Solution

