Show that the axial chromatic aberration. (fr−fv) for a convex lens is equal to the product of its mean focal length (f) and dispersive power (ω) of its materialie. Prove :
fr−fv=ωf
To prove that the axial chromatic aberration fr−fv for a convex lens is equal to the product of its mean focal length f and the dispersive power ω of its material, we can follow these steps:
1. Lens Maker's Formula: Start with the lens maker's formula, which is given by:
1f=(n−1)(1R1−1R2)
where n is the refractive index of the lens material, and R1 and R2 are the radii of curvature of the lens surfaces.
2. Focal Lengths for Different Colors: For red light, the refractive index is nr and for violet light, it is nv. Using the lens maker's formula, we can write:
1fr=(nr−1)(1R1−1R2)
1fv=(nv−1)(1R1−1R2)
3. Expressing in Terms of Mean Focal Length: The mean focal length f can be expressed as:
1f=(n−1)(1R1−1R2)
where n is the average refractive index.
4. Rearranging the Equations: We can rearrange the equations for fr and fv:
1fr=(nr−1)(n−1)⋅1f
1fv=(nv−1)(n−1)⋅1f
5. Finding the Difference: Now, we subtract the two equations:
1fv−1fr=((nv−1)(n−1)−(nr−1)(n−1))⋅1f
This simplifies to:
1fv−1fr=(nv−nr)(n−1)⋅1f
6. Using the Approximation: Since fr and fv are very close, we can write:
fr−fv≈f2⋅(nv−nr)(n−1)
7. Defining Dispersive Power: The dispersive power ω is defined as:
ω=nv−nrn−1
8. Final Result: Substituting ω into the equation gives:
fr−fv=ω⋅f
Thus, we have proved that:
fr−fv=ωf
1f=(n−1)(1R1−1R2)
where n is the refractive index of the lens material, and R1 and R2 are the radii of curvature of the lens surfaces.
1fr=(nr−1)(1R1−1R2)
1fv=(nv−1)(1R1−1R2)
1f=(n−1)(1R1−1R2)
where n is the average refractive index.
1fr=(nr−1)(n−1)⋅1f
1fv=(nv−1)(n−1)⋅1f
1fv−1fr=((nv−1)(n−1)−(nr−1)(n−1))⋅1f
This simplifies to:
1fv−1fr=(nv−nr)(n−1)⋅1f
fr−fv≈f2⋅(nv−nr)(n−1)
ω=nv−nrn−1
fr−fv=ω⋅f
fr−fv=ωf
Similar Questions
Show that the axial chromatic aberration. (fr−fv) for a convex lens is equal to the product of its mean focal length (f) and dispersive power (ω) of its materialie. Prove :
fr−fv=ωf
View SolutionConsider an equiconvex lens of radius of curvature R and focal length f. If f>R , the refractive index μ of the material of the lens
View SolutionThe power of lens is P=1f' where f is focal length of the lens. The dimensions of power of lens is
View SolutionThe power of lens is P=1f where f is focal length of the lens. The dimensions of power of lens is
View SolutionAn achromatic doublet is formed by combining two lenses.if the focal length of the lenses and their dispersive powers are f,f′and ω,ω′ respectively then
View SolutionThe plane surface of a plano-convex lens of focal length f is silvered. It will behave as
View SolutionThe dispersive powers of two materials are 0.30&0.28. They are used to construct two lenses which are kept in contact to eliminate chromatic aberration (that means the fv=fr the focal ) If the material of disperive power 0.30 is 10cm, then the focal lenght (for av, color) of the lens of other material is :
View SolutionWhen a thin convex lens is put in contact with a thin concave lens of the same focal length (f), then the resultant combination has focal length equal to
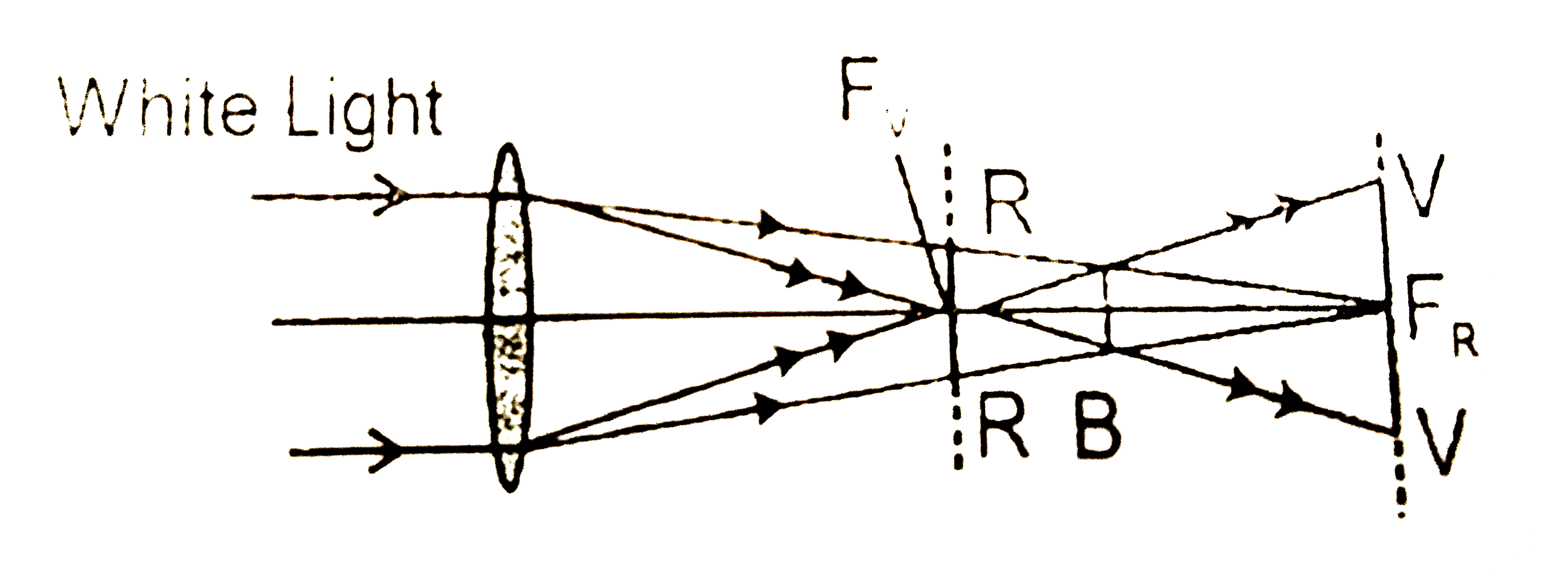
View SolutionThe image of a white object in with light formed by a lens is usually colored and blurred. This defect of image is called chromatic aberration and arises due to the fact that focal length of a lens is different for different colours. As R .I. μ of lens is maximum for violet while minimum for red, violet is focused nearest to the lens while red farthest from it as shown in figure.
As a result of this, in case of convergent lens if a screen is placed at Fv center of the image will be violet and focused while sides are red and blurred. While at FR , reverse is the case, i.e ., center will be red and focused while sides violet and blurred. The differece between fv and fR is a measure of the longitudinal chromatic aberration (L.C.A),i.e.,
L.C.A.=fR−fv=−df with df=fv−fR ...........(1)
However, as for a single lens,
1f=(μ−1)[1R1−1R2] .............(2)
⇒−dff2=dμ[1R1−1R2] ...............(3)
Dividing E1n. (3) by (2) :
−dff=dμ(μ−1)=ω,[ω=dμ(μ−1)]dispersive power,.........(4)
And hence, from Eqns. (1) and (4) ,
L.C.A.=−df=ωf
Now, as for a single lens neither f nor ω zero, we cannot have a single lens free from chromatic aberration.
Condition of Achromatism :
In case of two thin lenses in contact
1F=1F1+1F2i.c,.−dFF2=df1f21−df2f22
The combination will be free from chromatic aberration if dF=0
i.e.,df1f21+df2f22=0 which with the help of Eqn. (4) reduces to
ω1f1f21+ω2f2f22=0,i.e.,ω1f1+ω2f2=0........(5)
This condition is called condition of achromatism (for two thin lenses in contact ) and the lens combination which satisfies this condition is called achromatic lems, from this condition, i.e., form Eqn. (5) it is clear the in case of achromatic doublet :
Since, if ω1=ω2,1f1+1f2=0i.e.,1F=0 or F=∞
i.e., combination will not behave as a lens, but as a plane glass plate.
(2) As ω1 and ω2 are positive quantities, for equation (5) to hold, f1 and f2 must be of opposite nature, i.e., if one of the lenses is converging the other must be diverging.
(3) If the achromatic combination is convergent,
fC<fD and as fCfd=ωCωD,ωC<ωd
i.e., in a convergent achromatic doublet, convex lens has lesses focal legth and dispersive power than the divergent one.
A combination is made of two lenses of focal lengths f and f' in contact , the dispersive powers of the materials of the lenses are ω and ω' . The combination is achromatic when :
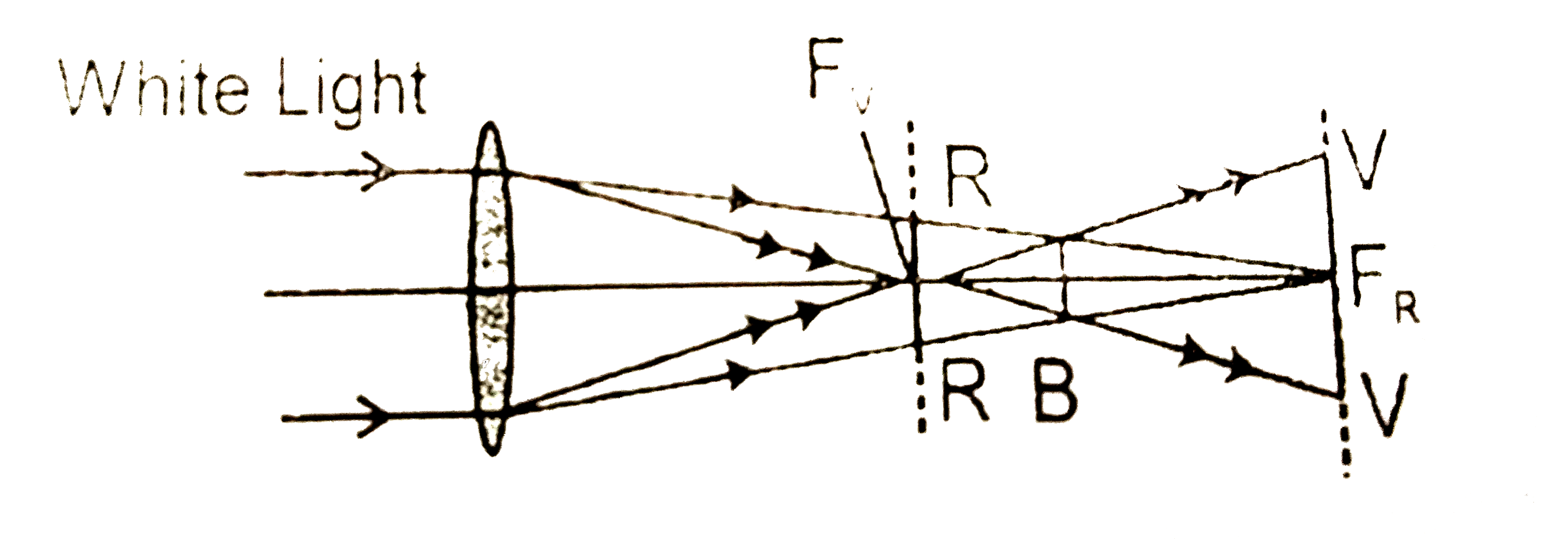
View SolutionThe image of a white object in with light formed by a lens is usually colored and blurred. This defect of image is called chromatic aberration and arises due to the fact that focal length of a lens is different for different colours. As R .I. μ of lens is maximum for violet while minimum for red, violet is focused nearest to the lens while red farthest from it as shown in figure.
As a result of this, in case of convergent lens if a screen is placed at Fv center of the image will be violet and focused while sides are red and blurred. While at FR , reverse is the case, i.e ., center will be red and focused while sides violet and blurred. The differece between fv and fR is a measure of the longitudinal chromatic aberration (L.C.A),i.e.,
L.C.A.=fR−fv=−df with df=fv−fR ...........(1)
However, as for a single lens,
1f=(μ−1)[1R1−1R2] .............(2)
⇒−dff2=dμ[1R1−1R2] ...............(3)
Dividing E1n. (3) by (2) :
−dff=dμ(μ−1)=ω,[ω=dμ(μ−1)]dispersive power,.........(4)
And hence, from Eqns. (1) and (4) ,
L.C.A.=−df=ωf
Now, as for a single lens neither f nor ω zero, we cannot have a single lens free from chromatic aberration.
Condition of Achromatism :
In case of two thin lenses in contact
1F=1F1+1F2i.c,.−dFF2=df1f21−df2f22
The combination will be free from chromatic aberration if dF=0
i.e.,df1f21+df2f22=0 which with the help of Eqn. (4) reduces to
ω1f1f21+ω2f2f22=0,i.e.,ω1f1+ω2f2=0........(5)
This condition is called condition of achromatism (for two thin lenses in contact ) and the lens combination which satisfies this condition is called achromatic lems, from this condition, i.e., form Eqn. (5) it is clear the in case of achromatic doublet :
Since, if ω1=ω2,1f1+1f2=0i.e.,1F=0 or F=∞
i.e., combination will not behave as a lens, but as a plane glass plate.
(2) As ω1 and ω2 are positive quantities, for equation (5) to hold, f1 and f2 must be of opposite nature, i.e., if one of the lenses is converging the other must be diverging.
(3) If the achromatic combination is convergent,
fC<fD and as fCfd=ωCωD,ωC<ωd
i.e., in a convergent achromatic doublet, convex lens has lesses focal legth and dispersive power than the divergent one.
Chromatic aberration in the formation of image by a lens arises because :
View Solution
