If the median of x, 2x, 4x is 12, then find mean of the data.Solution in Telugu
Step by step video & image solution for If the median of x, 2x, 4x is 12, then find mean of the data. by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Topper's Solved these Questions
- BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - FREQUENCY DISTRIBUTION TABLES AND GRAPHSEXERCISE - EXERCISE133videos
FREQUENCY DISTRIBUTION TABLES AND GRAPHS
- BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - FACTORISTIONEXERCISE - EXERCISE182videos
FACTORISTION
- BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - LINEAR EQUATIONS IN ONE VARIABLE EXERCISE - EXERCISE223videos
LINEAR EQUATIONS IN ONE VARIABLE
Similar Questions
Find the arithmetic mean of the sales per day in a fair price shop in a week. ₹10000, ₹10250,₹10790,₹ 9865,₹15350,₹10110.
02:59View SolutionFind the common factors of the give terims in each :8x, 24.
View SolutionWhich of the following are linear equations : 4x+6=8
View SolutionData: 10,15,20,15,25,x; mode = median,find x,mean
00:29View SolutionFind the mean deviation about median for the following data:
02:48View SolutionFind the mean, median and mode of the following data :
01:25View SolutionFind the mode when median is 12 and mean is 16 of a data .
06:13View SolutionIf the mean of 26 , 19 , 15 , 24 , and x is x , then find the median of the data .
01:15View Solutionयदि किन्ही आँकड़ों का माध्य तथा बहुलक क्रमशः 24 और 12 हों, तो माधियका ज्ञात करो ।
01:48View SolutionFind the mean deviation about the median for the data
(i) Find median.
(ii) Hence find mean deviation from the median.02:40View SolutionIf the median of a data is 12 and the polymer is 18, find the mean of the data.
04:51View SolutionThe arithmetic mean and mode of a data are 24 and 12 respectively, then find the median of the data.
04:04View SolutionIf the difference of mode and median of a data is 24, then find the difference of median and mean.
03:31View SolutionFind the mean deviation about the median for the data :
36,72,46,42,60,45,53,46,51,4904:15View SolutionFind the mean deviation about the median for the given data:
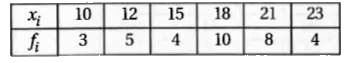
03:26View Solution