A particle is moving in a straight line. Its displacement at any instant t is given by , where x is in meters and t is in seconds. Find
(i) the average acceleration in the intervasl t = 0 to t = 2s and
(ii) instantaneous acceleration at t = 2 s. Solution in Assamese
Step by step video & image solution for A particle is moving in a straight line. Its displacement at any instant t is given by x = 10 t+ 15 t^(3), where x is in meters and t is in seconds. Find (i) the average acceleration in the intervasl t = 0 to t = 2s and (ii) instantaneous acceleration at t = 2 s. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
What is vector product of two vectors ? Give two examples .
View SolutionAt what position, velocity of a particle in SHM is maximum?
View SolutionA particle is moving with uniform acceleration. Its displacement at any instant t is given by s=10t+49t2 .find (i) initial velocity (ii) velocity at t=3second and(iii) the uniform acceleration.
View SolutionA particle moves along a straight line such that its displacement s at any time t is given by s=t3−6t2+3t+4m, t being is seconds. Find the velocity of the particle when the acceleration is zero.
View SolutionA partical is moving in a straight line such that s=t^(3)-3t^(2)+2 , where s is the displacement in meters and t is in seconds. Find the (a) velocity at t=2s , (b) acceleration at t=3s , ( c) velocity when acceleration is zero and (d) acceleration when velocity is zero.
View SolutionThe position of a particle moving on the x-axis is given by x=t^(3)+4t^(2)-2t+5 where x is in meter and t is in seconds (i) the velocity of the particle at t=4 s is 78 m//s (ii) the acceleration of the particle at t=4 s is 32 m//s^(2) (iii) the average velocity during the interval t=0 to t=4 s is 30 m//s (iv) the average acceleration during the interval t=0 to t=4 s is 20 m//s^(2)
View SolutionAcceleration time graph of a particle moving along a straight line is given. Then average acceleration between t=0 & t=4 is :−
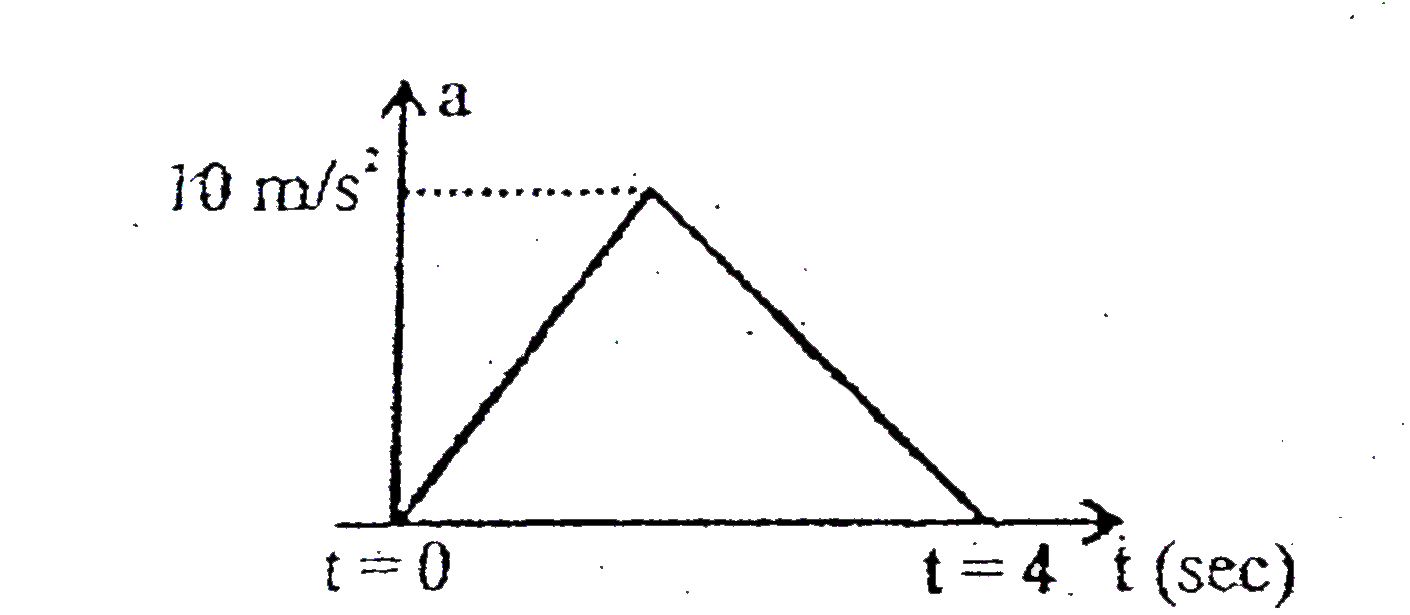
View SolutionLet s(t)=t3−6t2 be the position function of a particle moving along an s-axis, where s is in meters and t is in sec. find the instantaneous acceleration a(t) and show the graph of acceleration versus time.
View SolutionA particle is moving in a straight line. Its displacement at any instant t is given by x = 10 t+ 15 t^(3) , where x is in meters and t is in seconds. Find (i) the average acceleration in the intervasl t = 0 to t = 2s and (ii) instantaneous acceleration at t = 2 s.
View Solutionसरल रेखा में गतिमान एक कण का त्वरण (a) समय (t) ग्राफ संलग्न चित्र में प्रदर्शित है। कण का प्रारम्भिक वेग 2 मीटर/सेकण्ड है। ज्ञात कीजिए (i) t=3 सेकण्ड तथा t=6 सेकण्ड पर कण का वेग (ii) t=0 से t=6 सेकण्ड के बीच कण का औसत त्वरण।
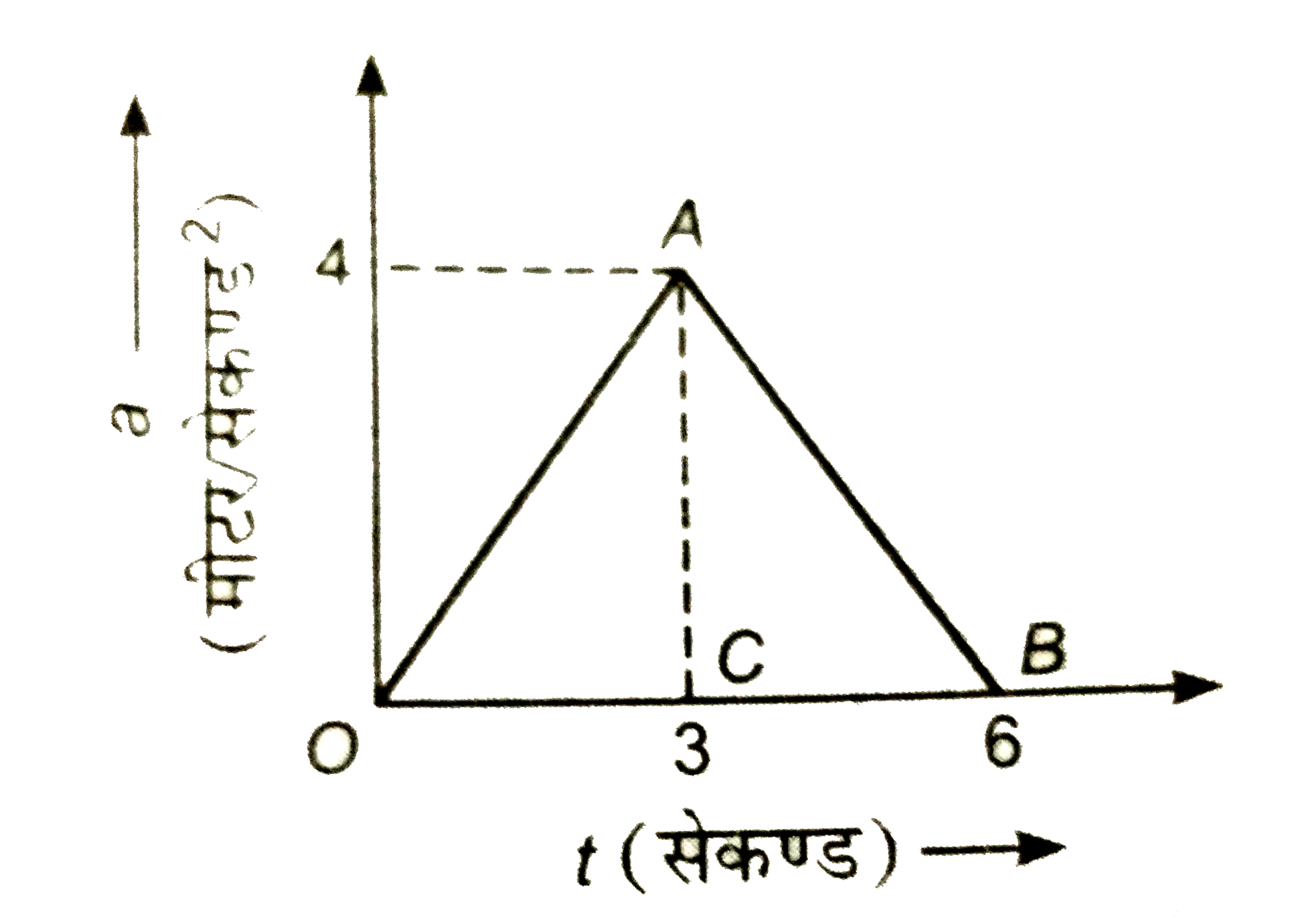
View Solutionएक सरल रेखा में गतिमान कण किसी क्षण पर स्थिति निम्नलिखित सूत्र द्वारा दी जाती है :
x=bt+c
जहाँ b=5⋅0 मी/से तथा c=−3⋅0 मीटर है x मीटर में तथा t सेकण्ड में है ज्ञात कीजिए :
t=−1 सेकण्ड t=0⋅5 सेकण्ड तथा t=10 सेकण्ड पर कर की स्थिति |
View Solutionकिसी क्षण t पर एक कण का विस्थापन निम्नलिखित सूत्र द्वारा दिया है : x=−15t2+20t+30, जहाँ x मीटर में तथा t सेकण्ड में है | t=0 पर कण की त्वरण ज्ञात कीजिए ।
View SolutionA particle moves along x-axis and its acceleration at any time t is a = 2 sin (πt), where t is in seconds and a is in m/s2 . The initial velocity of particle (at time t = 0) is u = 0.
Q. Then the magnitude of displacement (in meters) by the particle from time t = 0 to t = t will be :
View SolutionDisplacement of a particle is given by the expression x = 3 t ^ { 2 } + 7 t - 9 , where x is in meter and t is in seconds. What is acceleration ?
View SolutionA particle is moving in a straight line its displacement at any instant t is given by x=5t2+20t3. Find the average acceleration in the interval t = 0 to t = 3 seconds.
View Solutionकिसी क्षण t पर किसी कण का विस्थापन निम्नलिखित सूत्र द्वारा दिया जाता है -
x=−15t2+20t+30
जहाँ x, मीटर में तथा t, सेकण्ड में है। समय t = 0 पर कण की स्थिति, वेग तथा त्वरण ज्ञात कीजिये।
View Solution
