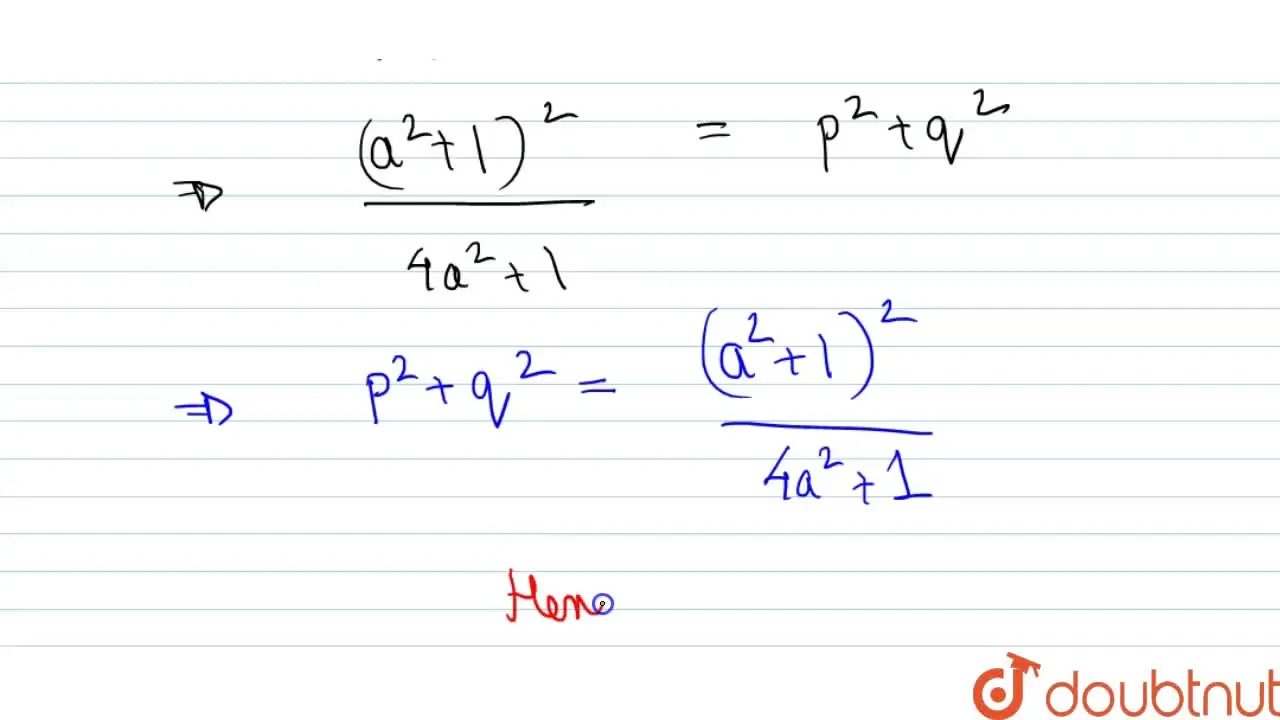
If (a+i)22a−i=p+iq then prove that p2+q2=⎛⎝(a2+1)24a2+1⎞⎠Solution in Assamese
Video Solution
|
Answer
Step by step video & image solution for If (a+i)^2/(2a-i)=p+iq then prove that p^2+q^2=((a^2+1)^2/(4a^2+1)) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023
Similar Questions
If (a+i)22a−i = p + iq, show that p2+q2=(a2+1)24a2+1
03:19View SolutionIf z=x−iy and z13=p+iq, then (xp+yq)/(p2+q2)=
05:37View Solution(a+i)22a−i=p+iq எனில், p2+q2=(a2+1)24a2+1 எனக்காட்டுக.
03:44View Solution(i) If x+iy=a+iba−ib, prove that x2+y2=1.
(ii) If (a+i)22a−i=p+iq, prove that:
p2+q2=(a2+1)24a2+1.05:49View SolutionIf (√3/2+(1/2)i√3/2−(1/2)i)120=p+iq,then
03:37View SolutionIf (a+i)2(2a−i)=p+iq, show that: p2+q2=(a2+1)2(4a2+1) .
04:28View SolutionProve that ∣∣ ∣∣(a+i)22a−i∣∣ ∣∣=a2+1√4a2+1
05:00View SolutionIf (a+i)22a−i=p+iq, prove that p2+q2=(a2+1)24a2+1
04:51View Solution