If f(x)=cosπ(|x|+[x]), then choose the incorrect option.
f is continuous at x =12
f is continuous at x = 0
f is differentiable in (-1,0)
f is differentiable in ( 0,1)
To solve the problem, we need to analyze the function f(x)=cos(π(|x|+(x))), where |x| is the absolute value of x and (x) is the greatest integer function (floor function). We will check the continuity and differentiability of the function in different intervals.
1. Identify the intervals:
The function needs to be analyzed in different intervals based on the behavior of |x| and (x):
- For x<−1
- For −1≤x<0
- For 0≤x<1
- For 1≤x<2
- For x≥2
2. Evaluate f(x) in the intervals:
- For x<−1:
|x|=−xand(x)=x(greatest integer less than x)
Thus, f(x)=cos(π(−x+x))=cos(0)=1.
- For −1≤x<0:
|x|=−xand(x)=−1
Thus, f(x)=cos(π(−x−1))=cos(π(−x−1))=cos(−πx−π)=−cos(πx).
- For 0≤x<1:
|x|=xand(x)=0
Thus, f(x)=cos(π(x+0))=cos(πx).
- For 1≤x<2:
|x|=xand(x)=1
Thus, f(x)=cos(π(x+1))=cos(πx+π)=−cos(πx).
- For x≥2:
|x|=xand(x)=x
Thus, f(x)=cos(π(x+x))=cos(2πx)=1.
3. Check continuity at the boundaries:
- At x=−1:
limx→−1−f(x)=−cos(π(−1))=−(−1)=1
limx→−1+f(x)=1
Since both limits are equal, f(x) is continuous at x=−1.
- At x=0:
limx→0−f(x)=−cos(0)=−1
limx→0+f(x)=cos(0)=1
Since the limits are not equal, f(x) is not continuous at x=0.
- At x=1:
limx→1−f(x)=cos(π)=−1
limx→1+f(x)=1
Since the limits are not equal, f(x) is not continuous at x=1.
4. Check differentiability:
- The function is not differentiable at points where it is not continuous. Thus, it is not differentiable at x=0 and x=1.
Conclusion:
The function f(x) is continuous everywhere except at x=0 and x=1, and it is differentiable in the intervals (−1,0) and (0,1) but not at the endpoints.
The function needs to be analyzed in different intervals based on the behavior of |x| and (x):
- For x<−1
- For −1≤x<0
- For 0≤x<1
- For 1≤x<2
- For x≥2
- For x<−1:
|x|=−xand(x)=x(greatest integer less than x)
Thus, f(x)=cos(π(−x+x))=cos(0)=1.
|x|=−xand(x)=−1
Thus, f(x)=cos(π(−x−1))=cos(π(−x−1))=cos(−πx−π)=−cos(πx).
|x|=xand(x)=0
Thus, f(x)=cos(π(x+0))=cos(πx).
|x|=xand(x)=1
Thus, f(x)=cos(π(x+1))=cos(πx+π)=−cos(πx).
|x|=xand(x)=x
Thus, f(x)=cos(π(x+x))=cos(2πx)=1.
- At x=−1:
limx→−1−f(x)=−cos(π(−1))=−(−1)=1
limx→−1+f(x)=1
Since both limits are equal, f(x) is continuous at x=−1.
limx→0−f(x)=−cos(0)=−1
limx→0+f(x)=cos(0)=1
Since the limits are not equal, f(x) is not continuous at x=0.
limx→1−f(x)=cos(π)=−1
limx→1+f(x)=1
Since the limits are not equal, f(x) is not continuous at x=1.
- The function is not differentiable at points where it is not continuous. Thus, it is not differentiable at x=0 and x=1.
The function f(x) is continuous everywhere except at x=0 and x=1, and it is differentiable in the intervals (−1,0) and (0,1) but not at the endpoints.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Choose the incorrect option (s):
APicric acid & Benzoic acid can be distringuished by NaHCO3B
< rate of SN2 reactionC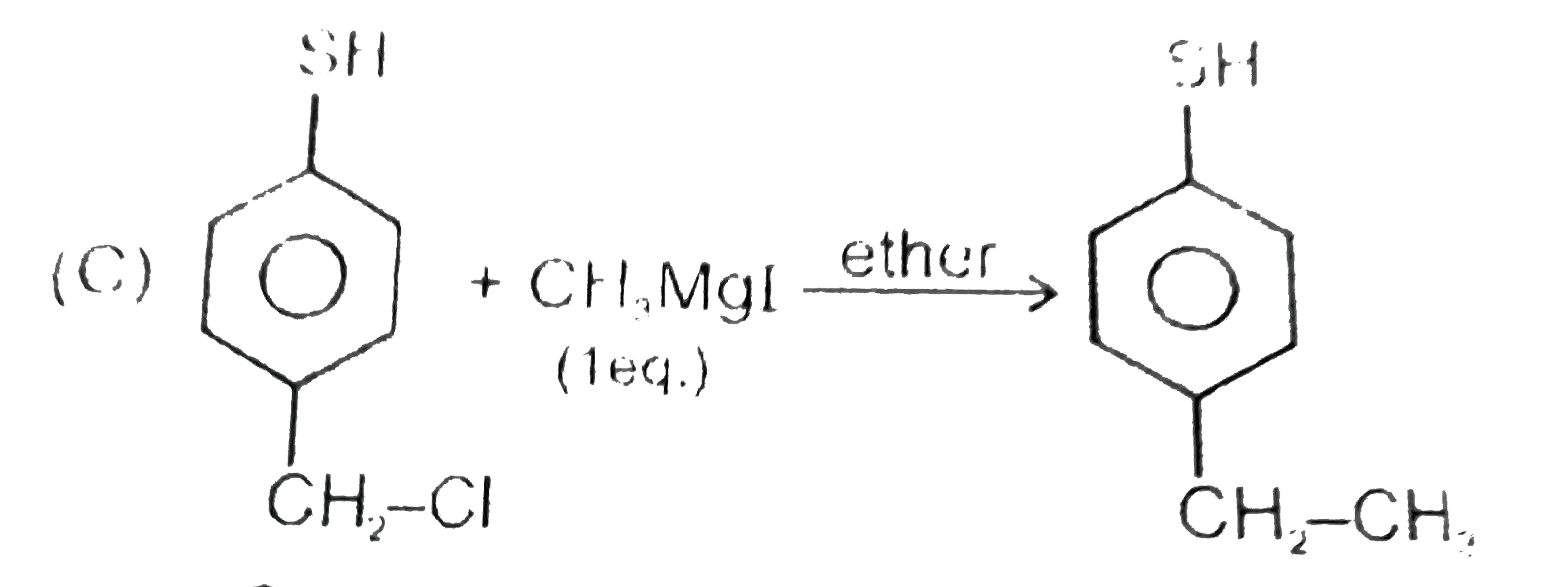
+CH3MgIether−−→(1eq.) D
does't show tautomerism.Choose the incorrect options (s) :
AO2F2 and OF2 both have open book structure.BInorganic benzene and organic benzene both have all sp2 hybridised atoms.CPure hydrogen is prepared action of dill H2SO4 on pure magnesium ribbonDCr2(SO4)3+H2O2+NaOh→Na2Cr2O7+Na2SO4+H2OChoose the incorrect option/options :
AIf acceleration is positive than speed of particle is increasing.BIf acceleration is negative than speed of particle is decreasing.CIn projectile motion speed of particle first decreases than increases.DIn ground to ground projectile motion horizontal range cannot be equal to the maximum height from the ground.