Write the relation between BE,BHandBV along with an appropriate diagram . Solution in Kannada
Figure shows the formation of image I of an object O on the principal axis of a convex surface with centre of curvature C.
As the aperture is small the length of NM will be taken to be nearly equal to the length of the perpendicular from the point N on the principal axis.
For small angles (assuming paraxiat rays) we have,
tan(∣NOM–––––––)≈∣NOM–––––––=MNOM
tan(∣NCM–––––––)≈∣NCM–––––––=MNMC
tan(∣NIM––––––)≈∣NIM––––––=MNMI
 Here i =
Here i =
|NOM–––––––+|NCM–––––––=MNOM+MNMC
and r =
|NCM–––––––−|NIM––––––=MNMC−MNMI
For small angles, Snell.s law, n1sini=n2=sinr can be written as,
n1i=n2=r
n1(MNOM+MNMC)=n2(MNMC−MNMI)
n1MN(1OM+1MC)=n2MN(1MC−1MI)
n1OM+n1MC=n2MC−n2MI
n1OM+n2MI=n2MC−n1MC
n1OM+n2MI=n2−n1MC
Applying the Cartesian sign convention, OM=−u,MI=+v,MC=+R
n1−u+n2v=n2−n1R
n2v−n1u=n2−n1R
Topper's Solved these Questions
- ANNUAL EXAM QUESTION PAPER MARCH - 2018BOOK - SUNSTAR PUBLICATIONCHAPTER - ANNUAL EXAM QUESTION PAPER MARCH - 2018EXERCISE - PART - E
5 Videos
- ANNUAL EXAM QUESTION PAPER MARCH - 2018BOOK - SUNSTAR PUBLICATIONCHAPTER - ANNUAL EXAM QUESTION PAPER MARCH - 2018EXERCISE - PART - C
8 Videos
- ANNUAL EXAM QUESTION PAPER MARCH - 2017BOOK - SUNSTAR PUBLICATIONCHAPTER - ANNUAL EXAM QUESTION PAPER MARCH - 2017EXERCISE - PART - C
40 Videos
- ANNUAL EXAM QUESTION PAPER MARCH -2015BOOK - SUNSTAR PUBLICATIONCHAPTER - ANNUAL EXAM QUESTION PAPER MARCH -2015EXERCISE - PART-A
37 Videos
Similar Questions
Three charges each equal to +4nC are placed at the three comers of a square of side 2 cm. Find the electric field at the fourth corner.
View SolutionMention any three properties of an electric charge.
View SolutionDerive a relation between electric field and potential
View SolutionDefine S.I unit of charge .
View SolutionIf BE represents equatorial magnetic field and BA represents axial magnetic field due to a bar magnet. Which of the following relationships between BE and BA is correct ?
View SolutionIf b1,b2,b3,…..bn are positive then the least value of (b1+b2+b3+…..+bn)(1b1+1b2+…..+1bn) is
View SolutionLet bi>1 for i=1,2,......,101. Suppose logeb1,logeb2,logeb3,........logeb101 are in Arithmetic Progression (AP) with the common difference loge2 . Suppose a1,a2,a3,........a101 are in AP. Such that, a1=b1 and a51=b51. If t=b1+b2+.........+b51 and s=a1+a2+.........+a51, then
View SolutionWrite the relation between B_(E), B_(H) "and" B_(V) along with an appropriate diagram .
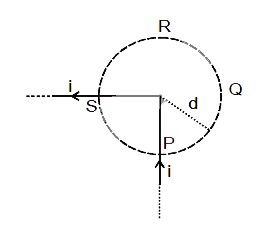
View SolutionFigures shows a long wire bent at the middle to form a right angle. The magnitudes of the magnetic fields at the points P, Q, R and S are B1,B2,B3,B4 respectively. The wire and the circumference of circle are coplanar. Relation between B1,B2,B3,B4 are
View Solution{:("(i) Horizontal component"B_(H),(a) l_(g) prop theta ),("(ii) vertical component"B_(v ),(b) B_(E) cos I ),((iii) "Galvanometer to a ammeter",(c ) l_(g) prop v ),("(iv) Galvanometer to a voltmeter",(d) B_(e) sin l):}
View SolutionWrite a general polynomial q(z) of degree n with coefficients that are b0,b1,b2,....bn,
What are the conditions on b0,b1,b2,....bn ?
View SolutionWrite a general polynomial q(z) of degree n with coefficients that are b0,b1,b2,....bn,
What are the conditions on b0,b1,b2,....bn ?
View SolutionWrite a general polynomial q(z) of degree n with coefficients that are b0,b1,b2,....bn,
What are the conditions on b0,b1,b2,....bn ?
View SolutionWrite a general polynomial q(z) of degree n with coefficients that are b0,b1,b2,....Nn,
What are the conditions on b0,b1,b2,....Bn ?
View SolutionIf b1,b2,b3,…. belongs to an A.P. such that b1+b4+b7+…+b28=220, then the value of b1+b2+b3+…+b28=
View Solutionयदि किसी छड़ चुम्बक के कारण BE विषुवतीय चुम्बकीय क्षेत्र तथा BA अक्षीय चुम्बकीय क्षेत्र को प्रदर्शित करते हैं, तो BE एवं BA के मध्य निम्न में कौन-सा सम्बन्ध सही है?
View Solution
