In the thermal decomposition of C2H5Br to C2H4 and HBr , the pressure changed from an initial value of 200 mmHg to 390 mmHg at the end of the reaction .What fraction remained unchanged when the pssure of the mixture was 300 mmHg?
To solve the problem, we need to analyze the thermal decomposition of C2H5Br into C2H4 and HBr in terms of pressure changes and the fraction of the reactant that has decomposed.
1. Identify Initial and Final Pressures:
- Initial pressure (Pi) = 200 mmHg
- Final pressure when the reaction is complete (Pf) = 390 mmHg
2. Determine the Change in Pressure:
- Change in pressure (ΔP) = Pf−Pi
ΔP=390 mmHg−200 mmHg=190 mmHg
3. Understand the Reaction:
- The decomposition reaction can be represented as:
C2H5Br→C2H4+HBr
- For every mole of C2H5Br that decomposes, the total pressure increases because two moles of products are formed from one mole of reactant.
4. Calculate the Total Pressure Change:
- The increase in pressure due to the complete decomposition of C2H5Br is equivalent to the change in pressure from the initial to the final state, which we calculated as 190 mmHg.
5. Determine the Pressure at 300 mmHg:
- We need to find out how much of C2H5Br has decomposed when the pressure is 300 mmHg.
- The change in pressure from the initial state to 300 mmHg is:
ΔP300=300 mmHg−200 mmHg=100 mmHg
6. Calculate the Fraction Decomposed:
- The fraction of C2H5Br that has decomposed at 300 mmHg can be calculated as:
Fraction decomposed=ΔP300ΔP=100 mmHg190 mmHg=1019
7. Determine the Fraction Unchanged:
- The fraction that remains unchanged is:
Fraction unchanged=1−Fraction decomposed=1−1019=919
Final Answer:
The fraction of C2H5Br that remained unchanged when the pressure of the mixture was 300 mmHg is 919.
- Initial pressure (Pi) = 200 mmHg
- Final pressure when the reaction is complete (Pf) = 390 mmHg
- Change in pressure (ΔP) = Pf−Pi
ΔP=390 mmHg−200 mmHg=190 mmHg
- The decomposition reaction can be represented as:
C2H5Br→C2H4+HBr
- For every mole of C2H5Br that decomposes, the total pressure increases because two moles of products are formed from one mole of reactant.
- The increase in pressure due to the complete decomposition of C2H5Br is equivalent to the change in pressure from the initial to the final state, which we calculated as 190 mmHg.
- We need to find out how much of C2H5Br has decomposed when the pressure is 300 mmHg.
- The change in pressure from the initial state to 300 mmHg is:
ΔP300=300 mmHg−200 mmHg=100 mmHg
- The fraction of C2H5Br that has decomposed at 300 mmHg can be calculated as:
Fraction decomposed=ΔP300ΔP=100 mmHg190 mmHg=1019
- The fraction that remains unchanged is:
Fraction unchanged=1−Fraction decomposed=1−1019=919
The fraction of C2H5Br that remained unchanged when the pressure of the mixture was 300 mmHg is 919.
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - RC MUKHERJEECHAPTER - CHEMICAL KINETICSEXERCISE - OBJECTIVE PROBLEMS
51 Videos
- CHEMICAL KINETICSBOOK - RC MUKHERJEECHAPTER - CHEMICAL KINETICSEXERCISE - OBJECTIVE PROBLEMS
51 Videos
- CHEMICAL EQUIVALENCEBOOK - RC MUKHERJEECHAPTER - CHEMICAL EQUIVALENCEEXERCISE - PROBLEMS
24 Videos
- CHEMICAL THERMODYNAMICSBOOK - RC MUKHERJEECHAPTER - CHEMICAL THERMODYNAMICSEXERCISE - Objective problems
58 Videos
Similar Questions
Complete the following reactions
(i) C2H5OC2H5+HCl→
(ii)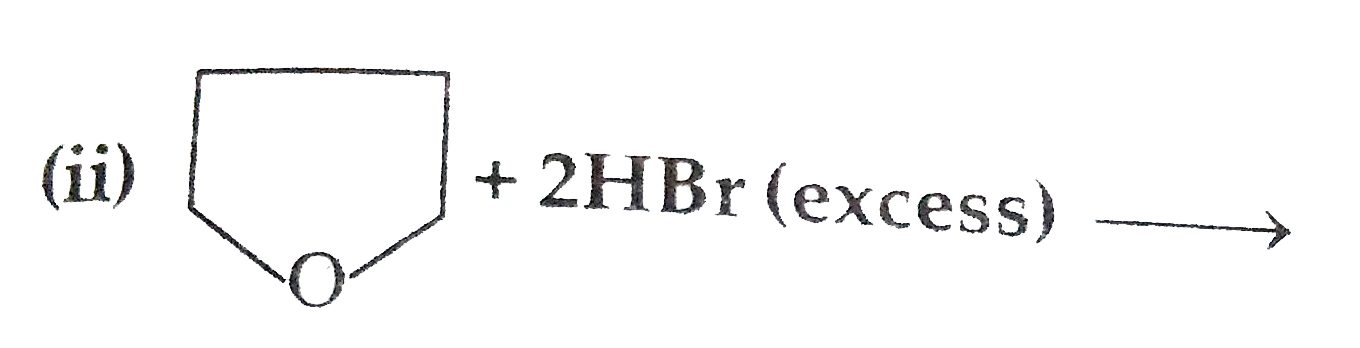
+ 2HBr (excess)→
View SolutionA mixture of CH4andC2H2 occupied a certain volume at a total pressure of 63 mm. The sample was burnt to CO2andH2O and the CO2 alone was collected and its pressure was found to be 69 mm in the same volume and at the same temperature as the original mixture. What fraction of the mixture was methane?
View Solution
Knowledge Check
The given reaction is an example of C2H5Br+KCN(aq.)→C2H5CN+KBr :
AeliminationBnucleophilic substitutionCelectrophilicDredox changeThe reaction,
C6H5Br+2Na+BrCH3→C6H5.CH3+2NaBr is known asAWurtz reactionBWurtz-Fitting reactionCFriedel-Crafts reactionDBerthelot synthesisA mixture of a mol of C3H8 and b mol of C2H4 was kept is a container of VL exerts a pressure of 4.93 atm at temperature T. Mixture was burnt in presence of O2 to convert C3H8 and C2H4 into CO2 in the container at the same temperature. The pressure of gases after the reaction and attaining the thermal equilirium with atomsphere at temperature T was found to be 11.08 atm.
The mole fraction of C2H4 in the mixture isA0.25B0.75C0.45D0.55
The decomposition of N2O5 according to the equation
2N2O5(g)→4NO2(g)+O2(g)
is a first - order reaction . After 30 minutes from the start of the decomposition in a closed vessel , the total pressure developed is found to be 284.5 mmHg and an complete decomposition the total pressure is 584.5 mmHg . Calculate the rate constant of the reaction .
View SolutionA mixture of a mol of C3H8 and b mol of C2H4 was kept is a container of VL exerts a pressure of 4.93 atm at temperature T. Mixture was burnt in presence of O2 to convert C3H8 and C2H4 into CO2 in the container at the same temperature. The pressure of gases after the reaction and attaining the thermal equilirium with atomsphere at temperature T was found to be 11.08 atm.
The moles fraction of C3H8 in the mixture is
View SolutionA mixture of a mol of C3H8 and b mol of C2H4 was kept is a container of VL exerts a pressure of 4.93 atm at temperature T. Mixture was burnt in presence of O2 to convert C3H8 and C2H4 into CO2 in the container at the same temperature. The pressure of gases after the reaction and attaining the thermal equilirium with atomsphere at temperature T was found to be 11.08 atm.
The moles of O2 needed for combustion at temperature T is equal to
View SolutionA sample of C2H6 gas initially at 50∘C and 720 mm Hg is heated to 100∘C in a container of constant volume. What is the new pressure (in mmHg)?
View SolutionThe reaction, C6H5Br+2Na+C2H5Br→C6H5C2H5+2NaBrisknownas:
View Solution