Answer the following questions (Alternatives are to be noted): If x is continuous random variable with p. d. f. {12−ax0≤x≤40otherwise where a is a constant, then find the value of a.Solution in Bengali
Step by step video & image solution for Answer the following questions (Alternatives are to be noted): If x is continuous random variable with p. d. f. {(1/2 - ax, ""0 le x le 4),(0,""otherwise):} where a is a constant, then find the value of a. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Find out the correct answer out of the options given against each question: E(x) = 4,E(y) =3,then E(xy)=
View Solution→a and →b are two vectors such that ∣∣→a+→b∣∣=∣∣→a−→b∣∣ then angle between →a and →b is
View Solutionमाना f(x)=⎧⎪⎨⎪⎩x2+ax+b,if 0≤x<23x+2,if 2≤x≤42ax+5b,if 4<x≤8
यदि फलन f(x), अन्तराल [0,8] में सतत है, तो a व b के मान ज्ञात कीजिये |
View SolutionX is continuous random variable with probability density function f(x)=x28,0≤x≤1. Then, the value of P(0.2≤X≤0.5) is
View SolutionShow that the function f(x) defined by .f(x)=17, for 1≤x≤8 =0, otherwise, is a probability density function for a random variable. Hence find P(3<X<10)
View Solution∫40f(x)dx जहाँ f(x)={2x+81≤x≤26x2≤x≤4 का मान ज्ञात कीजिएः
View SolutionIf the p.d.f of a random variable X is
F(x)={0.5x0≤x≤20otherwise
Then the value of P(0.5≤X≤1.5) is
View SolutionIf X is the random variable with probability density function f(x) is given by
f(x)=⎧⎪⎨⎪⎩x+1−1≤x<0−x+10≤x<10otherwise
then find (i) the distribution function F(x) (ii) P(−0.5≤X≤0.5)
View SolutionIf f(x)={2x0≤x≤a0otherwise is a probability density function of a random variable, then the value of a is
View SolutionIf f(x)=⎧⎨⎩√4+ax−√4−axx−1≤x<03x+2x−80≤x≤1 continuous in [−1,1], then the value of a is
View SolutionIf f(x)=⎧⎪⎨⎪⎩√1+ax−√1−axx,−1≤x<0x2+2x−2,0≤x≤1
continuous on [−1,1], then a =
View SolutionLet f(x)=⎧⎨⎩√1+ax−√1−axx−1≤x<02x+1x−20≤x≤1 is continuous in [-1, 1]. Then a equals :
View SolutionProbability distribution of a discrete random variable X is given in the following table.
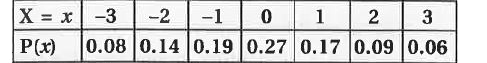
1) Find the probability of random variable X assuming negative values.
2) Find the value of P(0≤x≤3)
View SolutionX is continuous random variable with probability density function f(x)=x28,0≤x≤1. Then, the value of P(0.2≤X≤0.5) is
View SolutionIf the p.d.f of a random variable X is
f(x)={0.5x0≤x≤20otherwise
Then the value of P(0.5≤X≤1.5) is
View Solution
