A,B and C are three collinear points. B lies between A and C. A car stands on point A has reached to B after 3 seconds. If the angle of elevation of a pole of height 10m at C from the point A is 30∘ and from B is 45∘, then velocity of the car is (given that acceleration of car is constant).
Step by step video solution for A,B and C are three collinear points. B lies between A and C. A car stands on point A has reached to B after 3 seconds. If the angle of elevation of a pole of height 10m at C from the point A is 30^(@) and from B is 45^(@), then velocity of the car is (given that acceleration of car is constant). by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
A car moving the constant acceleration cross two points A and B with velocities 10m/s and 20m/s in its path.Find the velocity of the car midway between A and B . (in m/s)
View SolutionIn a car race, car A takes a time of t s less than car B at the finish and passes the finishing point with a velocity v m/s more than the car B. Assuming that the cars start from rest and travel with constant acceleration a_1 and a_2 respectively, velocity v is given by
View SolutionIn a ΔABC whose cicumradius is 2 . A pole standing inside the ΔABC and angle of elevation of top of the pole from points A,B,C is 60∘ then find height of pole
View SolutionA car moving with constant acceleration covered the distance between two points 60.0m apart in 6.00s. Its speed as it passes the second point was 15.0m/s.
(a) What is the speed at the first point?
(b) What is the acceleration?
(c) At what prior distance from the first was the car at rest?
View SolutionTwo cars A and B cross a point P with velocities 10m/s and 15m/s. After that they move with different uniform accelerations and the car A overtakes B with a speed of 25ms−1. What is velocity of B at that instant?
View SolutionIf the angles of elevation of the top of a water from three collinear points, B and C, on a line leading to the foot of the tower, are 30∘, 45∘ and 60∘ respectively, then the ratio, AB:BC,is:
View SolutionA car is moving round a circular track with a constant speed vof20ms−1 (As shown in figure).At different times,the car is at A,B and C,respectively . Find the velocity change (a) from A to C, and (b) from A to B.
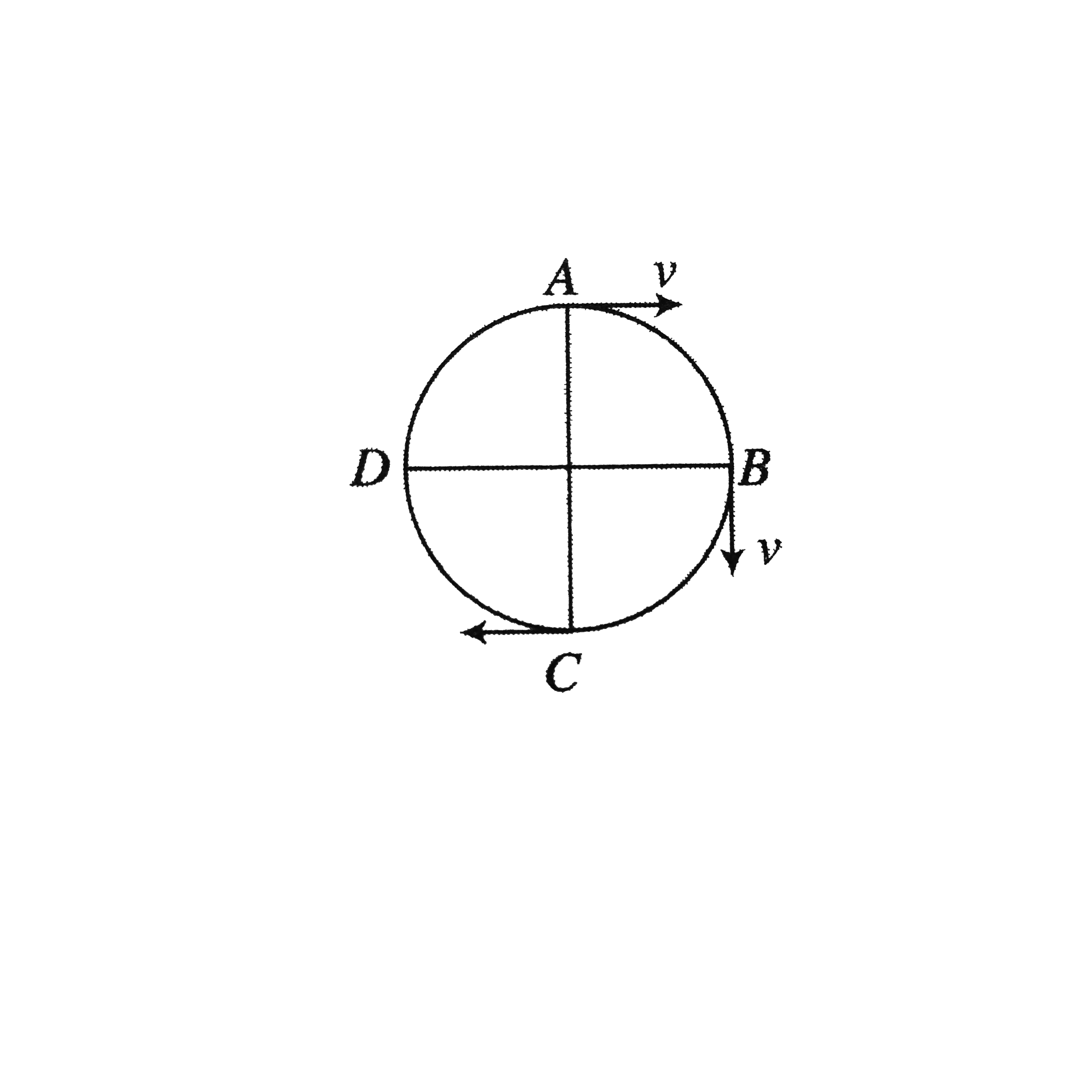 .
.
View Solution
