A tube of length L is filled completely with an incompressible liquid of mass M and closed at both the ends. The tube is then rotated in a horizontal plane about one of its ends with a uniform angular velocity ω. The force exerted by the liquid at the other end is
MLω22
ML2ω2
MLω2
ML2ω22
The correct Answer is:a
To solve the problem, we need to determine the force exerted by the liquid at the other end of the tube when it is rotated with a uniform angular velocity ω. Here’s a step-by-step solution:
Step 1: Understand the setup
We have a tube of length L filled with an incompressible liquid of mass M. The tube is closed at both ends and is rotating about one of its ends with an angular velocity ω.
Step 2: Consider a small segment of the liquid
Let’s consider a small segment of the liquid at a distance x from the end of the tube. The thickness of this segment is dx.
Step 3: Calculate the mass of the segment
The mass of this small segment dm can be expressed as:
dm=MLdx
where ML is the mass per unit length of the liquid in the tube.
Step 4: Determine the centripetal force on the segment
As the tube rotates, this segment experiences a centripetal force due to its circular motion. The centripetal force dF acting on this segment is given by:
dF=dm⋅ω2⋅x
Substituting dm from the previous step:
dF=(MLdx)⋅ω2⋅x
Thus, we can rewrite this as:
dF=Mω2L⋅xdx
Step 5: Integrate to find the total force
To find the total force F exerted by the liquid at the other end of the tube, we need to integrate dF from x=0 to x=L:
F=∫L0dF=∫L0Mω2L⋅xdx
Calculating the integral:
F=Mω2L∫L0xdx=Mω2L(x22)L0=Mω2L⋅L22
This simplifies to:
F=Mω2L2
Final Answer
The force exerted by the liquid at the other end of the tube is:
F=Mω2L2
We have a tube of length L filled with an incompressible liquid of mass M. The tube is closed at both ends and is rotating about one of its ends with an angular velocity ω.
Let’s consider a small segment of the liquid at a distance x from the end of the tube. The thickness of this segment is dx.
The mass of this small segment dm can be expressed as:
dm=MLdx
where ML is the mass per unit length of the liquid in the tube.
As the tube rotates, this segment experiences a centripetal force due to its circular motion. The centripetal force dF acting on this segment is given by:
dF=dm⋅ω2⋅x
Substituting dm from the previous step:
dF=(MLdx)⋅ω2⋅x
Thus, we can rewrite this as:
dF=Mω2L⋅xdx
To find the total force F exerted by the liquid at the other end of the tube, we need to integrate dF from x=0 to x=L:
F=∫L0dF=∫L0Mω2L⋅xdx
Calculating the integral:
F=Mω2L∫L0xdx=Mω2L(x22)L0=Mω2L⋅L22
This simplifies to:
F=Mω2L2
The force exerted by the liquid at the other end of the tube is:
F=Mω2L2
Similar Questions
Knowledge Check
A tube of length L is filled completely with an incompressible liquid of mass M and closed at both the ends. The tube is then rotated in a horizontal plane about one of its ends with a uniform angular velocity ω. Determine the force exerted by the liquid at the other end.
AMω2L2BMω2LCMω2L4DMω2L22
One end of a uniform rod of mas m and length l is clamped. The rod lies on a smooth horizontal surface and rotates on it about the clamped end at a uniform angular velocity ω. The force exerted by the clamp on the rod has a horizontal component
View SolutionA uniform of mass m and length L is tied to a vertical shaft. It rotates in horizontal plane about the vertical axis at angular velocity ω. How much horizontal force does the shaft exert on the rod?
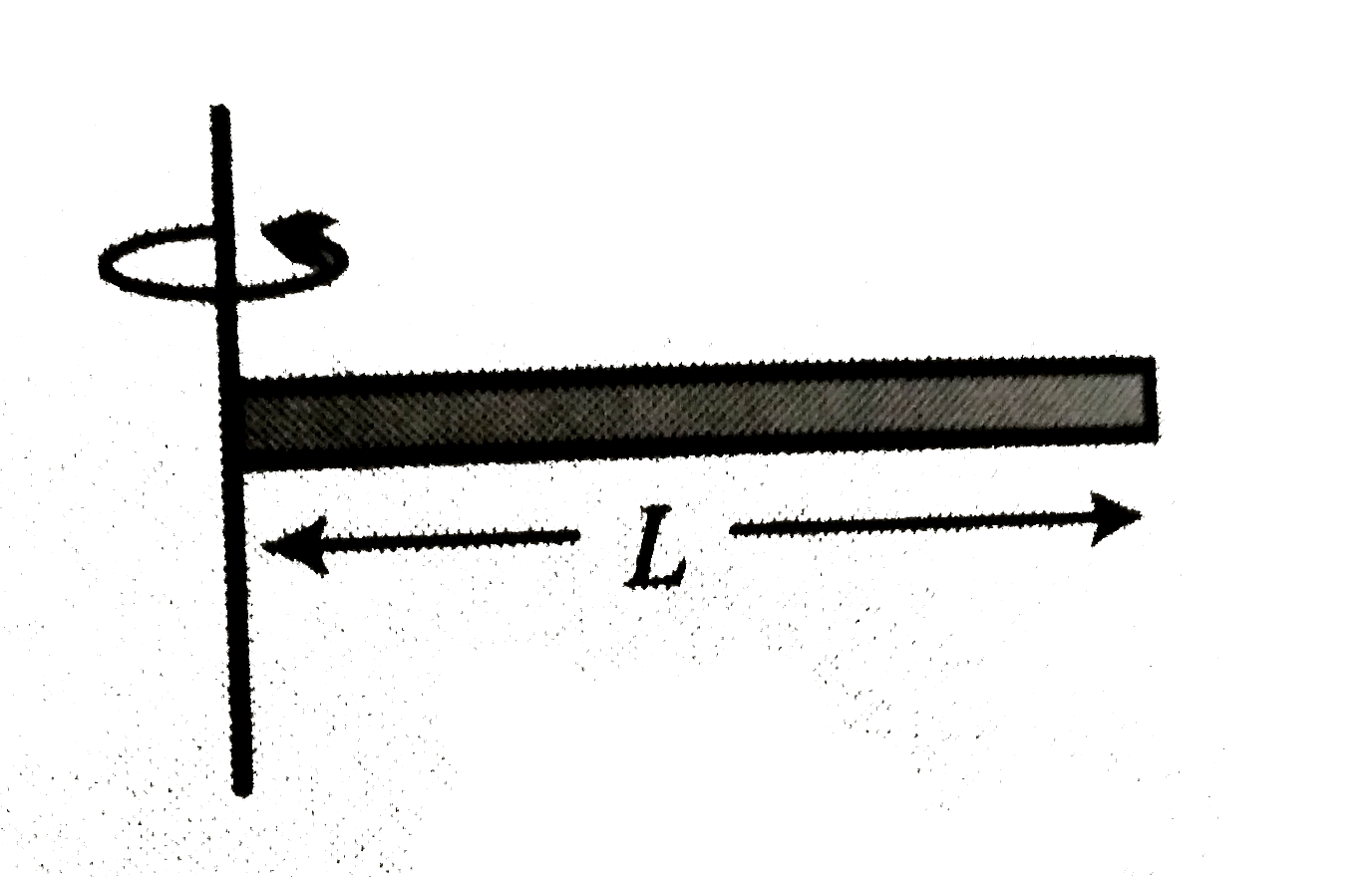
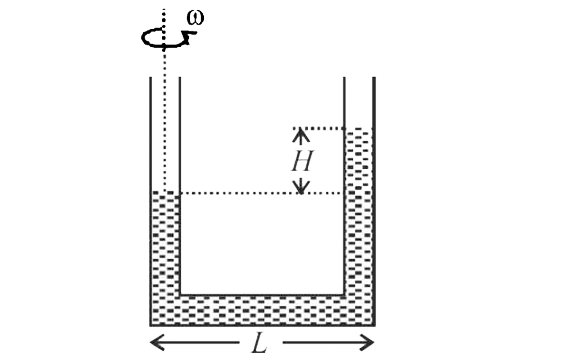
View SolutionA U tube is rotated about one of it's limbs with an angular velocity ω. Find the difference in height H of the liquid (density ρ) level, where diameter of the tube d<<L.
View SolutionA horizontal thin tube of length 2L completely filled with a liquid of density δ rotates about a vertical axis passing through one of its end and along the diameter of its side face with an constant angular velocity ω. Two vertical thin long tubes are fitted with the horizontal tube to measure the pressure difference between points A and B which are at same horizontal level as shown. Neglect viscous forces and surfaces tension of liquid.

The difference in the heights (h) of the liquid column in the two vertical thin tubes is :
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionA horizontal thin tube of length 2L completely filled with a liquid of density δ rotates about a vertical axis passing through one of its end and along the diameter of its side face with an constant angular velocity ω. Two vertical thin long tubes are fitted with the horizontal tube to measure the pressure difference between points A and B which are at same horizontal level as shown. Neglect viscous forces and surfaces tension of liquid.

The graph of the pressure gradient (dPdx) with the distance x meaured from the axis of rotation is :
View SolutionA copper rod of length l is rotated about one end perpendicular to the magnetic field B with constant angular velocity ω . The induced e.m.f between the two ends is
View Solution
