A plenet moving along an elliptical orbit is closest to the sun at a distance and farthest away at a distance of . If and are the linear velocities at these points respectively, then the ratio is
The correct Answer is:A
To solve the problem, we need to find the ratio of the linear velocities v1v2 of a planet moving in an elliptical orbit at its closest distance r1 and farthest distance r2 from the Sun.
1. Understanding Angular Momentum Conservation:
The angular momentum L of a planet moving in an orbit is conserved because the net external torque acting on the system is zero. This is due to the gravitational force acting along the line connecting the planet and the Sun, which does not create any torque.
L=m⋅r⋅v
where m is the mass of the planet, r is the distance from the Sun, and v is the linear velocity.
2. Setting Up the Angular Momentum Equation:
For the two positions of the planet (closest and farthest from the Sun), we can write the angular momentum as follows:
- At the closest point (distance r1):
L1=m⋅r1⋅v1
- At the farthest point (distance r2):
L2=m⋅r2⋅v2
3. Equating Angular Momenta:
Since angular momentum is conserved, we have:
L1=L2
Therefore:
m⋅r1⋅v1=m⋅r2⋅v2
4. Canceling Mass:
The mass m of the planet cancels out from both sides of the equation:
r1⋅v1=r2⋅v2
5. Finding the Ratio:
Rearranging the equation gives us:
v1v2=r2r1
Final Result:
Thus, the ratio of the linear velocities at the closest and farthest points from the Sun is:
v1v2=r2r1
The angular momentum L of a planet moving in an orbit is conserved because the net external torque acting on the system is zero. This is due to the gravitational force acting along the line connecting the planet and the Sun, which does not create any torque.
For the two positions of the planet (closest and farthest from the Sun), we can write the angular momentum as follows:
L1=m⋅r1⋅v1
L2=m⋅r2⋅v2
Since angular momentum is conserved, we have:
L1=L2
Therefore:
m⋅r1⋅v1=m⋅r2⋅v2
The mass m of the planet cancels out from both sides of the equation:
r1⋅v1=r2⋅v2
Rearranging the equation gives us:
v1v2=r2r1
Thus, the ratio of the linear velocities at the closest and farthest points from the Sun is:
v1v2=r2r1
Similar Questions
A planet moves around the sun. It is closest to sun to sun at a distance d1 and have velocity v1 At farthest distance d2 its speed will be
View SolutionA planet is revolving around the Sun in an elliptical orbit. Its closest distance from the sun is rmin. The farthest distance from the sun is rmax if the orbital angular velocity of the planet when it is nearest to the Sun ω then the orbital angular velocity at the point when it is at the farthest distance from the sun is
View Solution
Knowledge Check
A planet is revolving in an elliptical orbit around the sun. Its closest distance from the sun is r and the farthest distance is R. If the velocity of the planet nearest to the sun be v and that farthest away from the sun be V. then v/V is
AR2/r2Br2/R2CR/rDr/RA planet revolves around the sun in an elliptical orbit. If vp and va are the velocities of the planet at the perigee and apogee respectively, then the eccentricity of the elliptical orbit is given by :
AvpvaBva−vpva+vpCvp+vavp−vaDvp−vavp+va
µ_(1) and µ2 are the refractive index of two mediums and v1 and v2 are the velocity of light in these in two mediums respectively. Then, the relation connecting these quantities is
View SolutionA planet is revolving around the Sun in an elliptical orbit. Its closest distance from the Sun is r and farthest distance is R. If the orbital velocity of the planet closest to the Sun is v, then what is the velocity at the farthest point?
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionIf u1,u2 and v1,v2 are the initial and final velocities of two particles before and after collision respectively, then v2−v1u1−u2 is called ___________.
View SolutionIn fig., blocks A and B move with velocities v1 and v2 along horizontal direction. Find the ratio of v1/v2
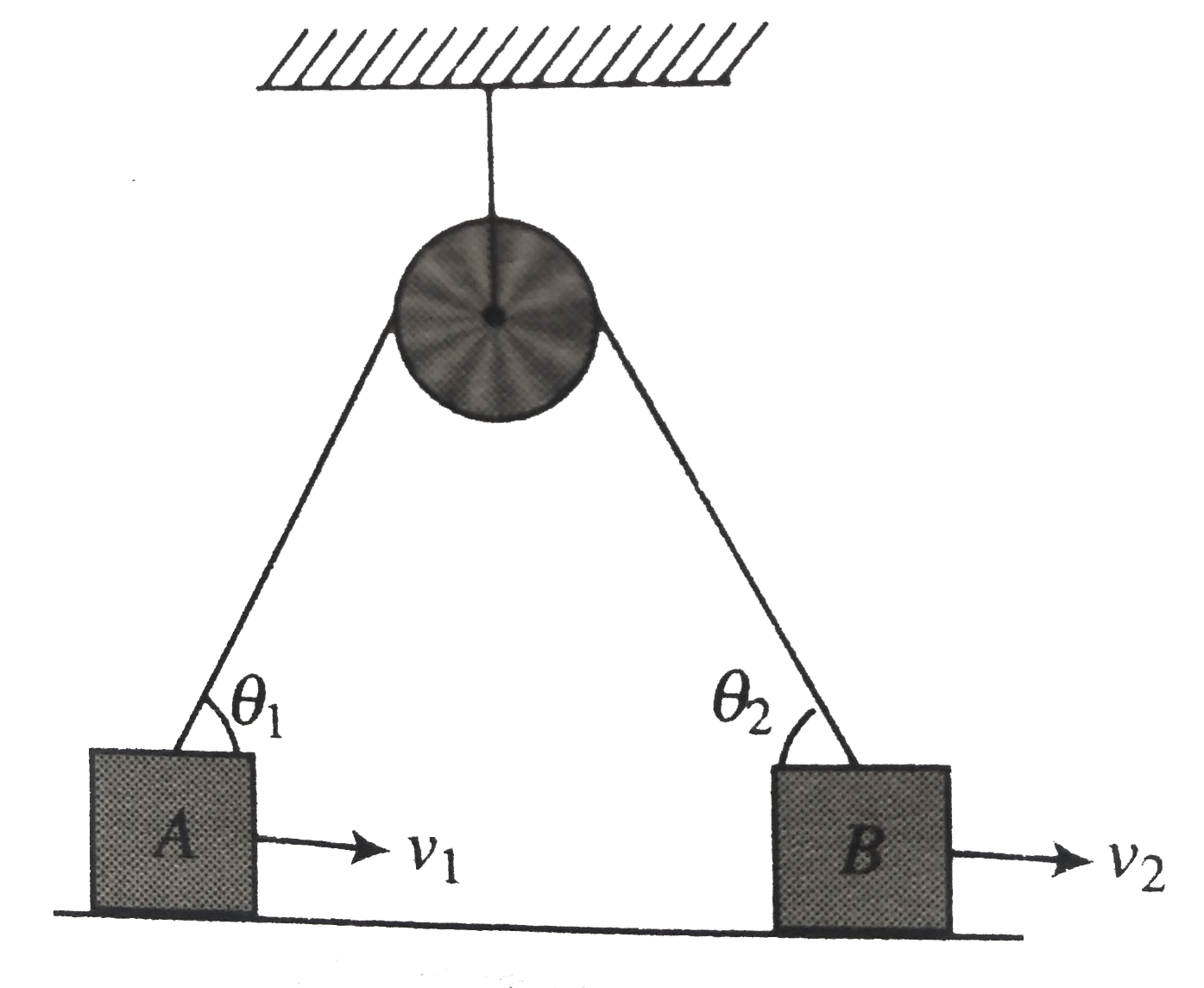
View Solution
