Under the influence of a unifrom magnetic field a charged particle is moving on a circle of radius R with Constnant speed v. The time period of the motion
depends on v and not on R
Depends on both R and v
is independent of both R and v
depends on R and not on v
The correct Answer is:c
To find the time period of a charged particle moving in a uniform magnetic field while tracing a circular path, we can follow these steps:
1. Understanding the Forces:
When a charged particle moves in a magnetic field, it experiences a magnetic force that acts as the centripetal force required for circular motion. The magnetic force F on a charged particle with charge Q moving with velocity v in a magnetic field B is given by:
F=QvBsin(θ)
Since the particle moves perpendicular to the magnetic field, θ=90∘ and sin(90∘)=1. Therefore, the magnetic force simplifies to:
F=QvB
2. Centripetal Force:
For circular motion, the centripetal force Fc required to keep the particle moving in a circle of radius R is given by:
Fc=mv2R
where m is the mass of the particle.
3. Equating Forces:
Since the magnetic force provides the necessary centripetal force, we can set the two forces equal to each other:
QvB=mv2R
4. Solving for Radius R:
Rearranging the equation to solve for R:
R=mvQB
5. Finding the Time Period T:
The time period T is the time taken to complete one full circular motion. The distance traveled in one complete revolution is the circumference of the circle, which is 2πR. Therefore, the time period can be expressed as:
T=DistanceSpeed=2πRv
6. Substituting for R:
Now substitute the expression for R into the equation for T:
T=2π(mvQB)v
The v in the numerator and denominator cancels out:
T=2πmQB
7. Conclusion:
The final expression for the time period T of the charged particle moving in a magnetic field is:
T=2πmQB
This shows that the time period is independent of the velocity v and the radius R.
When a charged particle moves in a magnetic field, it experiences a magnetic force that acts as the centripetal force required for circular motion. The magnetic force F on a charged particle with charge Q moving with velocity v in a magnetic field B is given by:
F=QvBsin(θ)
Since the particle moves perpendicular to the magnetic field, θ=90∘ and sin(90∘)=1. Therefore, the magnetic force simplifies to:
F=QvB
For circular motion, the centripetal force Fc required to keep the particle moving in a circle of radius R is given by:
Fc=mv2R
where m is the mass of the particle.
Since the magnetic force provides the necessary centripetal force, we can set the two forces equal to each other:
QvB=mv2R
Rearranging the equation to solve for R:
R=mvQB
The time period T is the time taken to complete one full circular motion. The distance traveled in one complete revolution is the circumference of the circle, which is 2πR. Therefore, the time period can be expressed as:
T=DistanceSpeed=2πRv
Now substitute the expression for R into the equation for T:
T=2π(mvQB)v
The v in the numerator and denominator cancels out:
T=2πmQB
The final expression for the time period T of the charged particle moving in a magnetic field is:
T=2πmQB
This shows that the time period is independent of the velocity v and the radius R.
Similar Questions
Knowledge Check
A particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B
 AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
A particle is moving in a circle with uniform speed its motion is
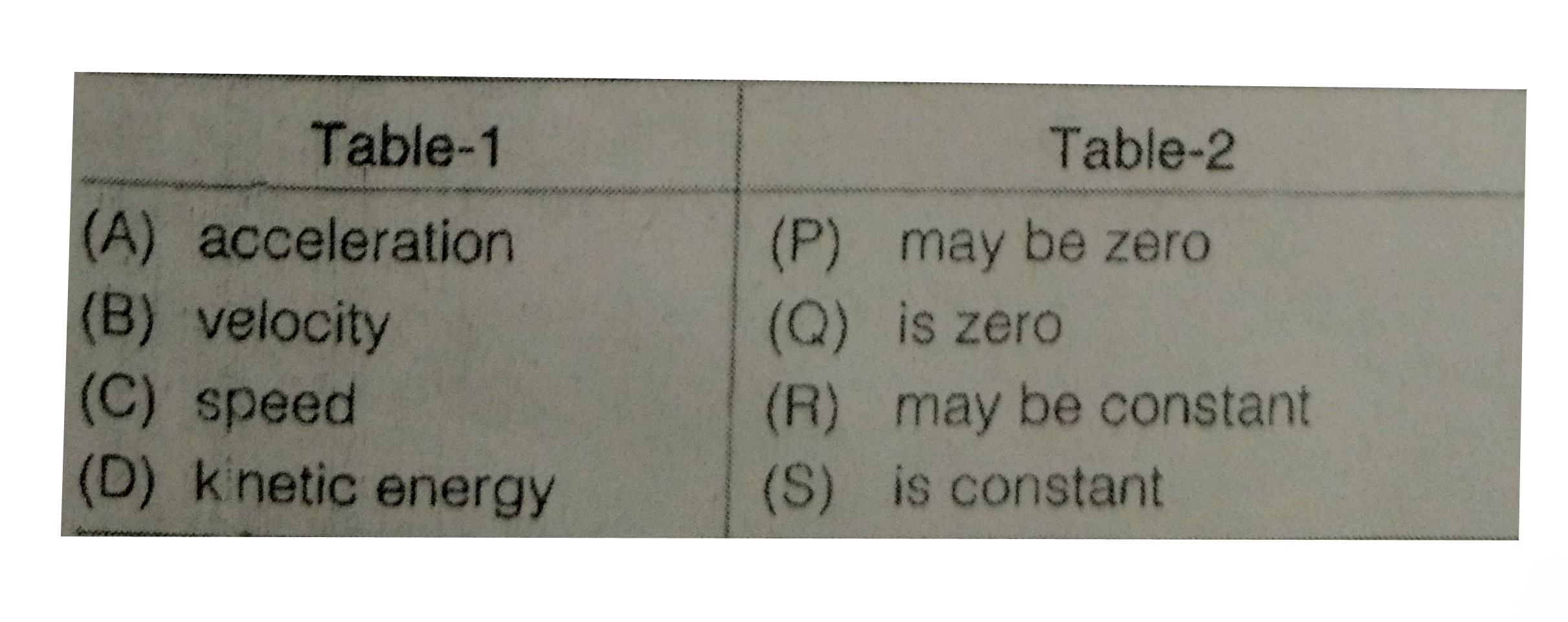
View SolutionIn magnetic field, for a charged particle (in motion) match the following table
View SolutionThe acceleration of an object moving in a circle of radius R with uniform speed v is
View SolutionA particle moves along a circle of radius R with a constant angular speed ω . Its displacement (only magnitude) in time t will be
View SolutionA particle is moving with speed v in a circle of radius R. Find the magnitude for average velocity of point
for the half revolution
View SolutionA particle with the constant speed in a circle of radius r and time period T.The centripetal acceleration of a particle is
View SolutionAn electron is moving on a circular path of radius r with speed v in a transverse magnetic field B. e/m for it will be
View Solution
