The ration of resolving powers of an optical microscope for two wavelangths
λ1=4000 Åandλ2=6000Åis
8:27
9:4
3:2
16:81
The correct Answer is:C
To find the ratio of the resolving powers of an optical microscope for two different wavelengths, we can follow these steps:
Step 1: Understand the relationship between resolving power and wavelength
The resolving power (R) of an optical instrument, such as a microscope, is inversely proportional to the wavelength (λ) of the light used. This can be expressed mathematically as:
R∝1λ
Step 2: Set up the ratio of resolving powers
Let R1 be the resolving power for wavelength λ1 and R2 be the resolving power for wavelength λ2. According to the relationship established in Step 1, we can write:
R1R2=λ2λ1
Step 3: Substitute the given values
Given:
- λ1=4000Å
- λ2=6000Å
We can substitute these values into the ratio:
R1R2=6000Å4000Å
Step 4: Simplify the ratio
Now, we simplify the fraction:
R1R2=60004000=64=32
Step 5: State the final answer
Thus, the ratio of the resolving powers of the optical microscope for the two wavelengths is:
R1R2=32
Final Answer:
The ratio of the resolving powers of the optical microscope for the two wavelengths λ1=4000Å and λ2=6000Å is 32.
---
The resolving power (R) of an optical instrument, such as a microscope, is inversely proportional to the wavelength (λ) of the light used. This can be expressed mathematically as:
R∝1λ
Let R1 be the resolving power for wavelength λ1 and R2 be the resolving power for wavelength λ2. According to the relationship established in Step 1, we can write:
R1R2=λ2λ1
Given:
- λ1=4000Å
- λ2=6000Å
R1R2=6000Å4000Å
Now, we simplify the fraction:
R1R2=60004000=64=32
Thus, the ratio of the resolving powers of the optical microscope for the two wavelengths is:
R1R2=32
The ratio of the resolving powers of the optical microscope for the two wavelengths λ1=4000Å and λ2=6000Å is 32.
Similar Questions
The ratio of resolving power of an optical microscope for two wavelength λ1=4000Å and λ2=6000Å is:
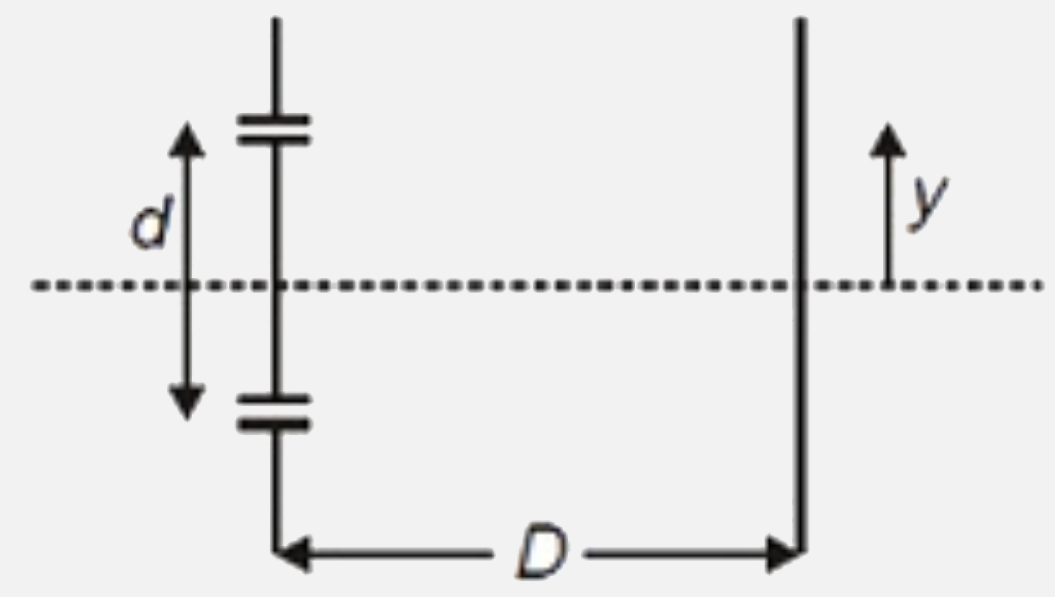
View SolutionIn YDSE a parallel beam of incident light consists of two wavelengths λ1=4000Åandλ2=5600Å. The minimum distance y on the screen, measured from the central axis, where the bright fringe due to two wavelengths coincide is nλ1Dd. Find n.
View SolutionWavelength of light used in an optical instrument are λ1=4000Å and λ2=5000Å then ratio of their respective resolving powers (corresponding to λ1 and λ2) is
View SolutionThe resolving power of a telescope whose lens has a diameter of 1.22 m for a wavelength of 5000Å is
View SolutionIf λ1,λ2andλ3 are the wavelengths of the wave giving resonance with the fundamental, first and second overtones respectively of a closed organ pipe Then the ratio of wavelength λ1,λ2andλ3 is
View SolutionV1 and V2 are the stopping potentials for the incident radiations of wave lengths λ1 and λ2 respectively are incident on a metallic surface. If λ1=3λ2 then
View SolutionWhat is the ratio between the energies of two types of radiation whose wavelengths are 6000 Å and 2000 Å respectively.
View SolutionStatement-1:An electron microscope can achieve better resolving power than an optical microscope
Statement-2:The de Broglie wavelength of the electrons emitted from an electron gun is much less than 500 nm
View SolutionA light source, which emits two wavelength λ1=400nm and λ2=600nm, is used in a Young's double slit experiment. If recorded fringe width for λ1 and λ2 are β1 and β2 and the number of fringes for them within a distance y on one side of the central maximum are m1 and m2 respectively, then
View SolutionWhen an object is viewed with a light of wavelength 6000Å under a microscope, its resolving power is 104. The resolving power of the microscope when the same object is viewed with a light of wavelength 4000Å, is n×103. The vlaue of n is
View Solution
