Two containers A and B are partly filled with water and closed. The volume of A is twice that of B and it contains half the amount of water in B. If both are at the same temperature, the water vapour in the containers will have pressure in the ratio of
The correct Answer is:b
To solve the problem, we need to analyze the information given about the two containers A and B.
1. Understanding the Containers:
- Container A has a volume that is twice that of container B.
- Let the volume of container B be V. Then, the volume of container A is 2V.
- Container A contains half the amount of water compared to container B.
- If container B has m grams of water, then container A has m2 grams of water.
2. Vapor Pressure Concept:
- The vapor pressure of a liquid is the pressure exerted by its vapor when the liquid and vapor are in equilibrium.
- Importantly, the vapor pressure of a substance depends only on the temperature and is independent of the amount of liquid present.
3. Applying the Concept to Containers:
- Since both containers are at the same temperature, the vapor pressure of water in both containers will be the same.
- Therefore, the vapor pressure in container A (let's denote it as PA) will be equal to the vapor pressure in container B (denote it as PB).
4. Finding the Ratio of Vapor Pressures:
- Since PA=PB, we can express the ratio of the vapor pressures as:
PAPB=1
Final Answer:
The ratio of the vapor pressures in the two containers A and B is 1:1.
---
- Container A has a volume that is twice that of container B.
- Let the volume of container B be V. Then, the volume of container A is 2V.
- Container A contains half the amount of water compared to container B.
- If container B has m grams of water, then container A has m2 grams of water.
- The vapor pressure of a liquid is the pressure exerted by its vapor when the liquid and vapor are in equilibrium.
- Importantly, the vapor pressure of a substance depends only on the temperature and is independent of the amount of liquid present.
- Since both containers are at the same temperature, the vapor pressure of water in both containers will be the same.
- Therefore, the vapor pressure in container A (let's denote it as PA) will be equal to the vapor pressure in container B (denote it as PB).
- Since PA=PB, we can express the ratio of the vapor pressures as:
PAPB=1
The ratio of the vapor pressures in the two containers A and B is 1:1.
Similar Questions
Two separate bulbs contain ideal gas A and B. The density of a gas A is twice that of a gas B. The molecular mass of A is half that of gas B. The two gases are at the same temperature. The ratio of the pressure of A to that gas B is
View SolutionContainers A and B have same gases. Pressure, volume and temperature of A are all twice that of B, then the ratio of number of molecules of A and B are
View SolutionContainers A and B have same gases. Pressure, volume and temperature of A are all twice that of B, then the ratio of number of molecules of A and B are
View SolutionTwo containers A and B have the same volume. Container A contains 5 moles of O2 gas. Container B contains 3 moles of He and 2 moles of N2. Both the containers are separately kept in vacuum at the same temperature. Both the containers have very small orifices of the same area through which the gases leak out. Compare the rate of effusion of O2 with that of He gas mixture.
View Solution10 g of solute A and 20gm of solute B are both dissolved in 500ml water. The solution has the same osmotic pressure as 6.67 gm of A and 30 gm of B dissolved in the same amount of water at the same temperature. What is the ratio of molar masses of A and B?
View SolutionThe density of a gas A is twice that of a gas B at the same temperature. The molecular mass of gas B is thrice that of A. The ratio of the pressure acting on A and B will be
View SolutionA tank is filled with water and two holes A and B are made in it.- For getting same range, ratio of h' and h is
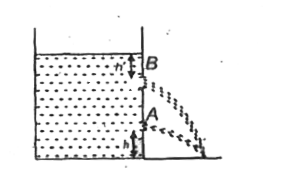
View SolutionA tumbler contains 3 L of water Calculate the volume of the water in m3.
View SolutionTwo containers A and B of equal volume V0/2 each are connected by a narrow tube which can be closed by a value. The containers are fitted with pistons which can be moved to change the volumes. Initially the value is open and the containers contain an ideal gas (Cp/Cv=γ) at atmospheric pressure P0 and atmospheric temperature 2T0. The walls of the containers A are highly conducting and of B are non-conducting. The value is now closed and the pistons are slowly pulled out to increase the volumes of the containers to double the original value. (a) Calculate the temperature and pressure in the two containers. (b) The value is now opened for sufficient time so that the gases acquire a common temperature and pressure. Find the new values of the temperature and the pressure.
View SolutionA container having some gas was kept in a moving train. The temperature of the gas in the container will
A. increase slightly
B. decrease
C. remain the same
D. become infinite
View Solution
