The values of ΔH and ΔS for the reaction,
Cgraphite+CO2(g)→2CO(g)
are 170KJ and 170JK−1 respectively. This reaction will be spontaneous at
710 K
910 K
1110 K
510 K
The correct Answer is:C
To determine the temperature at which the reaction C(graphite)+CO2(g)→2CO(g) becomes spontaneous, we can use the Gibbs free energy equation:
ΔG=ΔH−TΔS
For a reaction to be spontaneous, ΔG must be negative. Therefore, we need to find the temperature T at which:
ΔH−TΔS<0
This can be rearranged to:
TΔS>ΔH
Step 1: Convert ΔH to Joules
Given:
- ΔH=170kJ=170×103J
- ΔS=170J/K
Step 2: Set up the inequality
We need to find T such that:
T⋅170J/K>170×103J
Step 3: Solve for T
Rearranging the inequality gives:
T>170×103J170J/K
Step 4: Simplify the expression
The 170 in the numerator and denominator cancels out:
T>1000K
Conclusion
The reaction will be spontaneous at temperatures greater than 1000K.
Given:
- ΔH=170kJ=170×103J
- ΔS=170J/K
We need to find T such that:
Rearranging the inequality gives:
The 170 in the numerator and denominator cancels out:
The reaction will be spontaneous at temperatures greater than 1000K.
Similar Questions
Knowledge Check
Consider the following reaction :
CO(g)+12O2(g)→CO2(g)
How are ΔU and ΔH related for the reaction ?A(a) ΔH=ΔU−0.5RTB(b) ΔH=ΔU−RTC(c) ΔH=ΔU+0.5RTD(d) ΔH=ΔU−1.5RTThe value of enthalpy change (ΔH) for the reaction
C2H5OH(l)+3O2(g)→2CO2(g)+3H2O(l)
at 27∘C is −1366.5kJmol−1. The value of internal energy change for the above reaction at this temperature will beA−1371.5kJB−1369.0kJC−1364.0kJD−1361.5kJ
Kp/Kc for the reaction
CO(g)+12O2(g)⇔CO2(g) is
View SolutionKp/Kc for the reaction
CO(g)+12O2(g)⇔CO2(g) is
View SolutionΔH and ΔS for
Br2(l)+CI2(g)→2BrCI(g)
are 29.00kJmol−1 and 100.0JK−1mol−1 respectively. Above what temperature will this reaction become spontaneous?
View SolutionA schematic representation of enthalpy changes for the reaction,Cgraphite+12O2(g)→CO(g) is given below. The missing value is
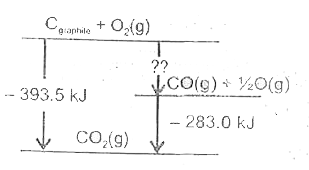
View SolutionFor the reaction
CO(g)+12O2(g)⇔CO2(g),Kp/Kc is
View SolutionFor hypothetical reversible reaction
1/2A2(g)+3/2B2(g)→AB3(g),ΔH=−20kJ if standard entropies of A2,B2, and AB3 are 60,40 and 50JK−1mol−1 respectively. The above reaction will be equilibrium at
View Solution
