A solid cylinder and a hollow cylinder, both of the same mass and same external diameter are released from the same height at the same time on an inclined plane. Both roll down without slipping. Which one will reach the bottom first ?
Both together
Hollow cylinder
Solid cylinder
Both together only when angle of inclination of plane is 45∘
The correct Answer is:C
To solve the problem of which cylinder reaches the bottom of the incline first, we need to analyze the motion of both the solid cylinder and the hollow cylinder as they roll down the inclined plane.
1. Understanding the Problem:
We have two cylinders (one solid and one hollow) of the same mass and external diameter released from the same height on an inclined plane. Both are rolling without slipping.
2. Acceleration of Rolling Objects:
The acceleration a of a rolling object on an incline can be expressed as:
a=gsinθ1+IMR2
where:
- g is the acceleration due to gravity,
- θ is the angle of the incline,
- I is the moment of inertia of the object,
- M is the mass of the object,
- R is the radius of the object.
3. Moments of Inertia:
- For a solid cylinder, the moment of inertia I is given by:
Isolid=12MR2
- For a hollow cylinder (or hollow ring), the moment of inertia I is:
Ihollow=MR2
4. Substituting Moments of Inertia:
- For the solid cylinder:
asolid=gsinθ1+12MR2MR2=gsinθ1+12=gsinθ32=2gsinθ3
- For the hollow cylinder:
ahollow=gsinθ1+MR2MR2=gsinθ1+1=gsinθ2
5. Comparing Accelerations:
- We can see that:
asolid=2gsinθ3andahollow=gsinθ2
- To compare these, we can find a common denominator:
- asolid=4gsinθ6
- ahollow=3gsinθ6
6. Conclusion:
Since asolid>ahollow, the solid cylinder has a greater acceleration than the hollow cylinder. Therefore, the solid cylinder will reach the bottom of the incline first.
Final Answer:
The solid cylinder will reach the bottom first.
We have two cylinders (one solid and one hollow) of the same mass and external diameter released from the same height on an inclined plane. Both are rolling without slipping.
The acceleration a of a rolling object on an incline can be expressed as:
a=gsinθ1+IMR2
where:
- g is the acceleration due to gravity,
- θ is the angle of the incline,
- I is the moment of inertia of the object,
- M is the mass of the object,
- R is the radius of the object.
- For a solid cylinder, the moment of inertia I is given by:
Isolid=12MR2
- For a hollow cylinder (or hollow ring), the moment of inertia I is:
Ihollow=MR2
- For the solid cylinder:
asolid=gsinθ1+12MR2MR2=gsinθ1+12=gsinθ32=2gsinθ3
- For the hollow cylinder:
ahollow=gsinθ1+MR2MR2=gsinθ1+1=gsinθ2
- We can see that:
asolid=2gsinθ3andahollow=gsinθ2
- To compare these, we can find a common denominator:
- asolid=4gsinθ6
- ahollow=3gsinθ6
Since asolid>ahollow, the solid cylinder has a greater acceleration than the hollow cylinder. Therefore, the solid cylinder will reach the bottom of the incline first.
The solid cylinder will reach the bottom first.
Similar Questions
A solid cylinder and a solid sphere, both having the same mass and radius, are released from a rough inclined plane of inclination θ one by one. They roll on the inclined plane without slipping. The force of friction that acts
View SolutionA solid cylinder C and a hollow pipe P of same diameter are in contact when they are released from rest as shown in the figure on a long incline plane. Cylinder C and pipe P roll with out slipping. Determine the clear gap (in m) between them after 4 seconds.
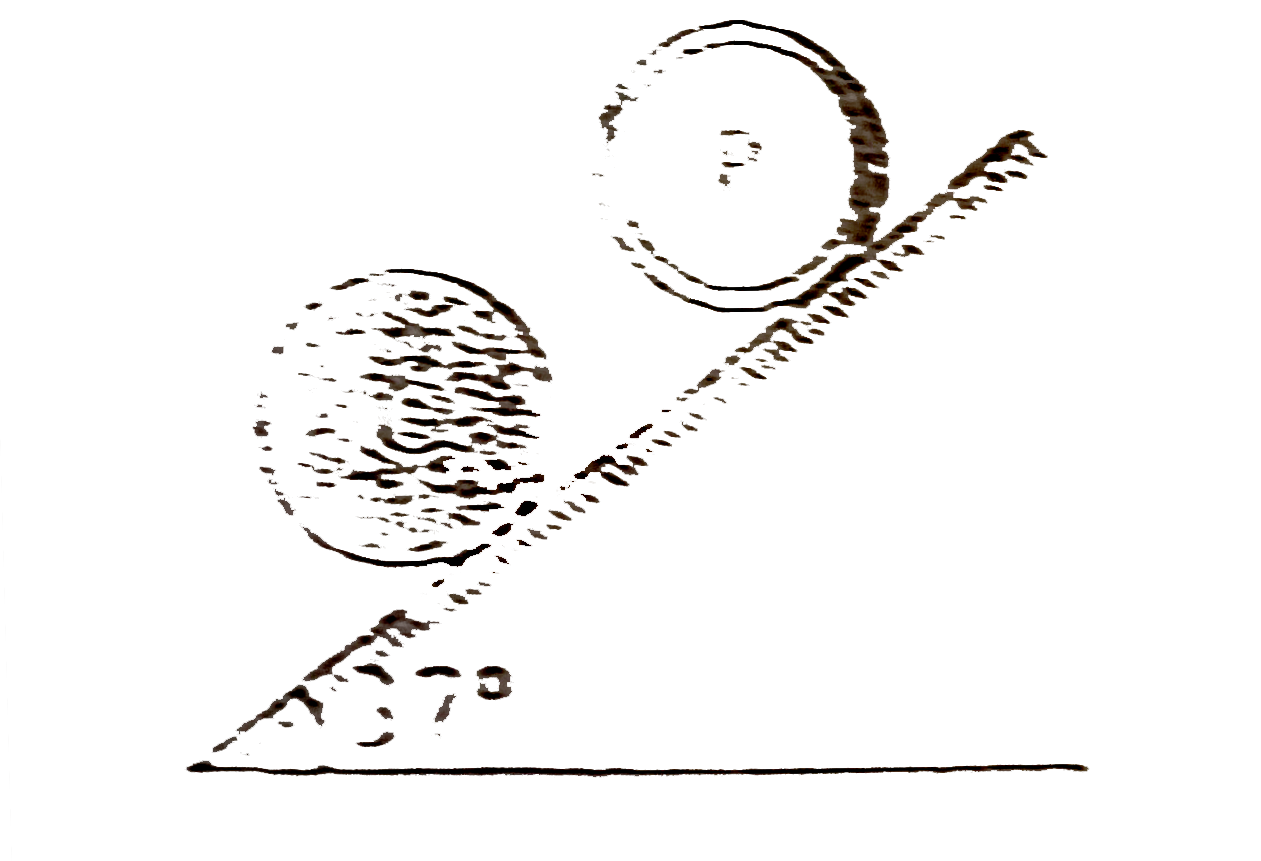
View SolutionA solid sphere and a solid cylinder having the same mass and radius, rolls down the same incline. The ratio of their acceleration will be
View SolutionTwo uniform solid spheres having unequal radii are released from rest from the same height on a rough incline. If the spheres roll without slipping
View SolutionTwo uniform solid spheres having unequal radii are released from rest from the same height on a rough incline. the spheres roll without slipping
View SolutionA ring starts to roll down the inclined plane of height h without slipping . The velocity when it reaches the ground is
View SolutionAssertion : A solid sphere and a ring of same mass and radius are released simultaneously from the top of an inclined surface. The two objects roll down the plane without slipping. They reach the bottom of the incline with equal linear speeds
Reason : Decrease in potential enery for both is the same.
View SolutionA ring, a solid cylinder, a hollow sphere and a solid sphere are released from rest on an inclined plane from same level. If there is no slipping then which one of these will reach the ground at last?
View SolutionA solid cylinder is rolling down the inclined plane without slipping. Which of the following is correct?

View SolutionTwo objects, a ring and a spherical shell of same mass and radius are released from the top of two Identical inclined plane. If they are rolling without slipping, then ratio of speed of center of mass of the two objects when they will reach the bottom of the inclined plane is
View Solution
