For the diffraction from a crystal with λ=1Å and Bragg's angle θ=60∘, then for the second order diffraction 'd' will be :
1.15 Å
0.75 Å
0.55 Å
2.1 Å
The correct Answer is:A
To solve the problem, we will use Bragg's Law, which is given by the equation:
nλ=dsinθ
Where:
- n is the order of diffraction,
- λ is the wavelength of the incident wave,
- d is the distance between the crystal planes,
- θ is the Bragg's angle.
1. Identify the given values:
- Wavelength λ=1Å=1×10−10m
- Bragg's angle θ=60∘
- Order of diffraction n=2
2. Write down Bragg's Law:
nλ=dsinθ
3. Rearrange the equation to solve for d:
d=nλsinθ
4. Substitute the known values into the equation:
d=2×1×10−10sin60∘
5. Calculate sin60∘:
sin60∘=√32
6. Substitute sin60∘ into the equation:
d=2×1×10−10√32
7. Simplify the equation:
d=2×1×10−10×2√3
d=4×10−10√3
8. Calculate the final value of d:
- Using √3≈1.732:
d≈4×10−101.732≈2.31×10−10m
- Converting to angstroms (1 Å = 10−10m):
d≈2.31Å
Final Answer:
The distance d for the second order diffraction is approximately 2.31Å.
- n is the order of diffraction,
- λ is the wavelength of the incident wave,
- d is the distance between the crystal planes,
- θ is the Bragg's angle.
- Wavelength λ=1Å=1×10−10m
- Bragg's angle θ=60∘
- Order of diffraction n=2
nλ=dsinθ
d=nλsinθ
d=2×1×10−10sin60∘
sin60∘=√32
d=2×1×10−10√32
d=2×1×10−10×2√3
d=4×10−10√3
- Using √3≈1.732:
d≈4×10−101.732≈2.31×10−10m
- Converting to angstroms (1 Å = 10−10m):
d≈2.31Å
The distance d for the second order diffraction is approximately 2.31Å.
Similar Questions
Light is incident normally on a diffraction grating through which the first order diffraction is seen at 32∘. The second order diffraction will be seen at
View SolutionWave property of electron implies that they will show diffraction effected . Davisson and Germer demonstrated this by diffracting electron from crystals . The law governing the diffraction from a crystals is obtained by requiring that electron waves reflected from the planes of atoms in a crystal inter fere constructiely
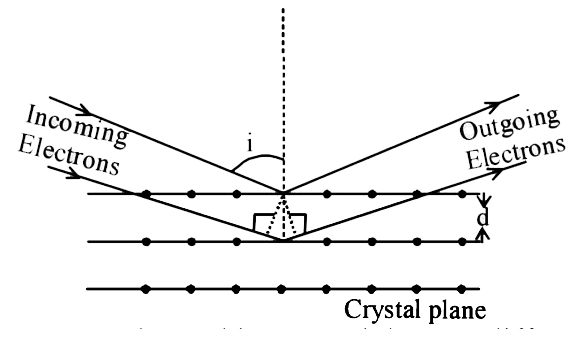
If a strong diffraction peak is observed when electrons are incident at an angle i from the normal to the crystal planes with distance d between them (see fig) de Brogle wavelength λdB of electrons can be calculated by the relationship (n is an intenger)
View SolutionA slit of width a is illuminiated by white light. The first diffraction minimum for light of λ=6500Å is formed at θ=30∘, then the width a of the slit is
View SolutionWave property of electron implies that they will show diffraction effected . Davisson and Germer demonstrated this by diffracting electron from crystals . The law governing the diffraction from a crystals is obtained by requiring that electron waves reflected from the planes of atoms in a crystal interfere constructiely
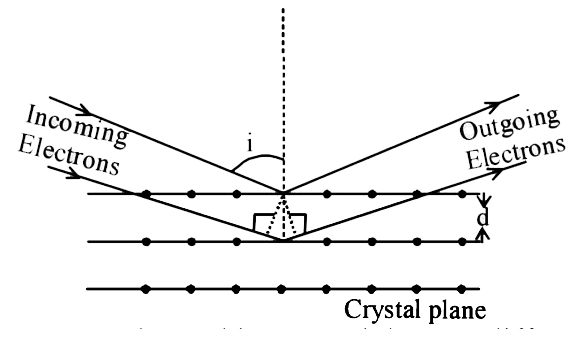
Electron accelerated by potential V are diffracted from a crystal if d=1Åandi=30∘,V should be about (h=6.6×10−34Js,me=9.1×10−31kg,e=1.6×10−19C)
View SolutionCalculate λ of X-rays which give a diffraction angle 2θ=16.8∘ for crystal, if the interplanar distance in the crystal is 0.2nm and that only for the first-order diffraction is observed. Given sin8.40∘=0.146.
View SolutionWave property of electrons implies that they will show diffraction effects. Davission and Germer demonstrated this by diffracting electrons from crystals. The law governing the diffraction from a crystal is obtained by requiring that electron waves reflected from the planes of atoms in a crystal interfere constructively (see figure)
_E01_233_Q01.png)
The surface of a metal is illuminated with the light of 400nm. The kinetic energy of the ejected photoelectrons was found to be 1.68eV. The work function of the metal is : (hc=1240eV−nm)
View SolutionA beam of monochromatic light of wavelength λ falls normally on a diffraction grating of line spacing d. If θ is the angle between the second-order diffracted beam and the direction of the incident light, what is the value of sinθ ?
View SolutionA beam of monochromatic light of wavelength λ falls normally on a diffraction grating of line spacing d. If θ is the angle between the second-order diffracted beam and the direction of the incident light, what is the value of sinθ ?
View SolutionAn X-ray beam (λ=70.9pm) was scattered by a crystalline solid. The angle (2θ) of the diffraction for a second order reflection is 14.660. Calculate the distance between parallel planes of atoms of the crystalline solid.
View SolutionIn a double-slit experiment, green light (5303Å) falls on a double slit having a separation of 19.44μm and a width of 4.05μm. The number of bright fringes between the first and the second diffraction minima is:
View Solution
