An object is placed on principal axis of concave mirror at distance of 1.5 f Image will be at
-3f
1.5f
-1.5f
3f
The correct Answer is:A
To solve the problem of finding the image position when an object is placed at a distance of 1.5f from a concave mirror, we can follow these steps:
1. Identify the given values:
- Object distance (u) = -1.5f (the negative sign indicates that the object is in front of the mirror).
- Focal length (f) = -f (for a concave mirror, the focal length is considered negative).
2. Use the mirror formula:
The mirror formula is given by:
1f=1v+1u
where:
- f is the focal length,
- v is the image distance,
- u is the object distance.
3. Substitute the values into the mirror formula:
1−f=1v+1−1.5f
4. Rearranging the equation:
1v=1−f−11.5f
5. Finding a common denominator:
The common denominator for the right side is 1.5f:
1v=−1.51.5f+11.5f
1v=−1.5+11.5f=−0.51.5f
6. Simplifying the equation:
1v=−13f
7. Taking the reciprocal to find v:
v=−3f
8. Conclusion:
The image will be formed at a distance of -3f from the mirror, which means it is located on the same side as the object, and it is a real and inverted image.
Final Answer:
The image will be at a distance of -3f.
- Object distance (u) = -1.5f (the negative sign indicates that the object is in front of the mirror).
- Focal length (f) = -f (for a concave mirror, the focal length is considered negative).
The mirror formula is given by:
1f=1v+1u
where:
- f is the focal length,
- v is the image distance,
- u is the object distance.
1−f=1v+1−1.5f
1v=1−f−11.5f
The common denominator for the right side is 1.5f:
1v=−1.51.5f+11.5f
1v=−1.5+11.5f=−0.51.5f
1v=−13f
v=−3f
The image will be formed at a distance of -3f from the mirror, which means it is located on the same side as the object, and it is a real and inverted image.
The image will be at a distance of -3f.
Similar Questions
A 2.0 cm high object is placed on the principal axis of a concave mirror at a distance of 12 cm from the pole. Ilf the image is inverted, real and 5.0 cm high, find the location of the image the focal length of the mirror.
View SolutionA spherical mirror produces an image of magnification -1, when an object is placed on the principal axis of the mirror at a distance of 40 cm form its pole.
(a) Identify the nature of image.
(b) Identify the nature of spherical mirror.
(c) Find the distance between the object and its image.
(d) How will the nature of the image change, if the object is shifted 10 cm towards the mirror ? Draw a ray diagram to justify your answer.
[Hint. (a) Image is real, inverted/same size as the object, (b) Concave mirror, (c) Zero, (d) Image will be formed beyond C, It will be larger in size.]
View SolutionAn object is present on the principal axis of a concave mirror at a distance 30cm from it. Focal length of mirror is 20cm.
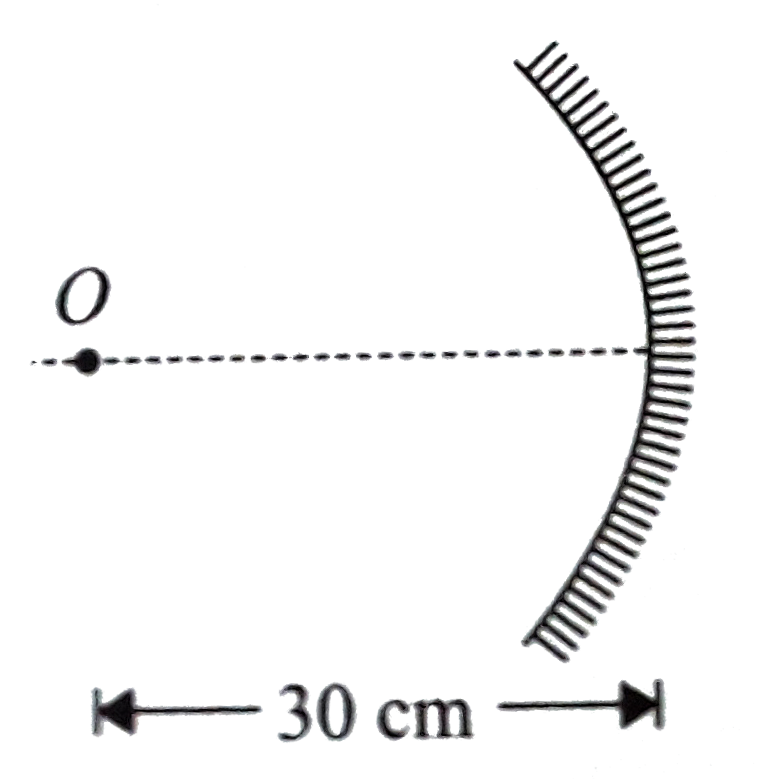
Q. Image formed by mirror is
View SolutionAn object is present on the principal axis of a concave mirror at a distance 30cm from it. Focal length of mirror is 20cm.
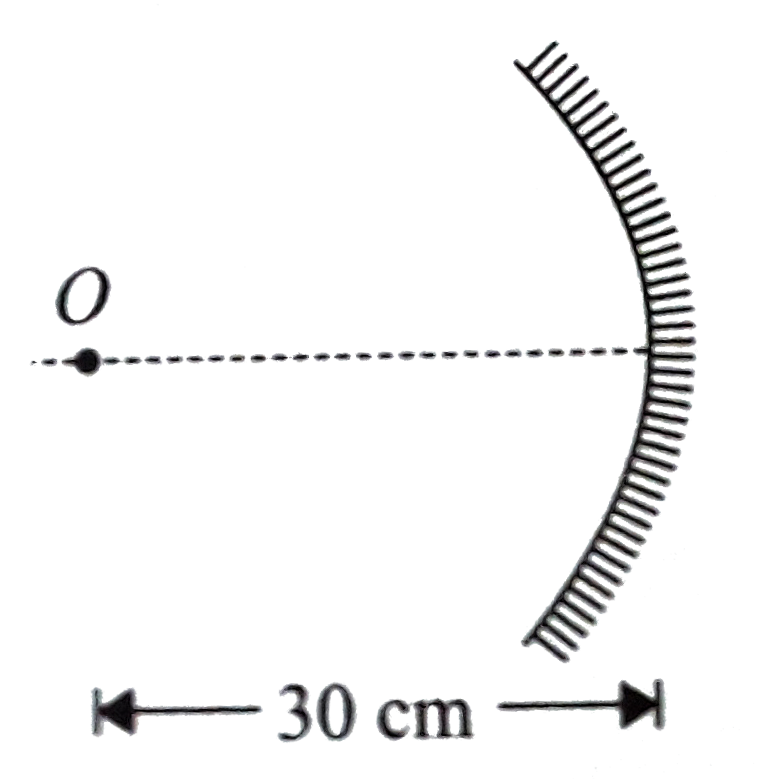
Q. If object starts moving with 2cms−1 perpendicular to principal axis above the principal axis then,
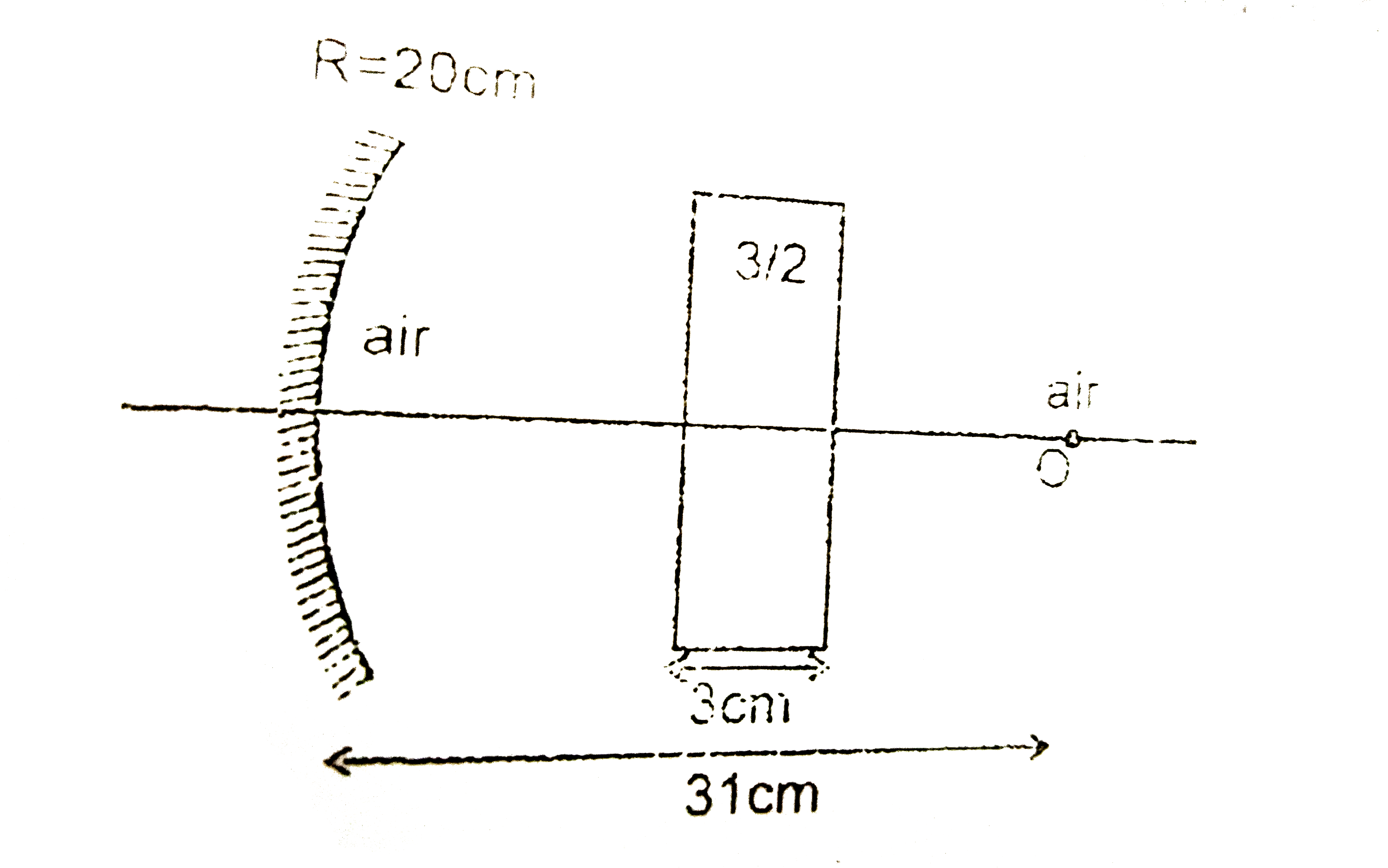
View SolutionA point object is placed on principal axis of a concave mirror of radius of curvature 20cm at a distance 31cm from poll of the mirror. A glass slab of thikness 3cm and refractive index 1.5 is placed between object and mirror as shown in the figure. Find the distance (in cm) of final image formed by the system from the mirror.
View SolutionAn object is placed in front of a concave mirror at a distance of 7.5 cm from it. If the real image is formed at a distance of 30 cm from the mirror, find the focal length of the mirror. What would be the focal length if the image is virtual.
View SolutionAn object is placed on the principal axis of concave mirror of focal length 10 cm at a distance of 8.0 cm from the pole. Find the position and the nature of the image.
View SolutionThe image of an object placed on the principal axis of a concave mirror of focal length 12 cm is formed at a point which is 10cm more distance form the mirror than the object. The magnification of the image is
View SolutionAn object is placed on the principle axis of a concave mirror of focal length 10cm at a distance of 21cm from it. A glass slab is placed between the mirror and the object as shown in figure.
Find the distance of final image formed by the mirror.

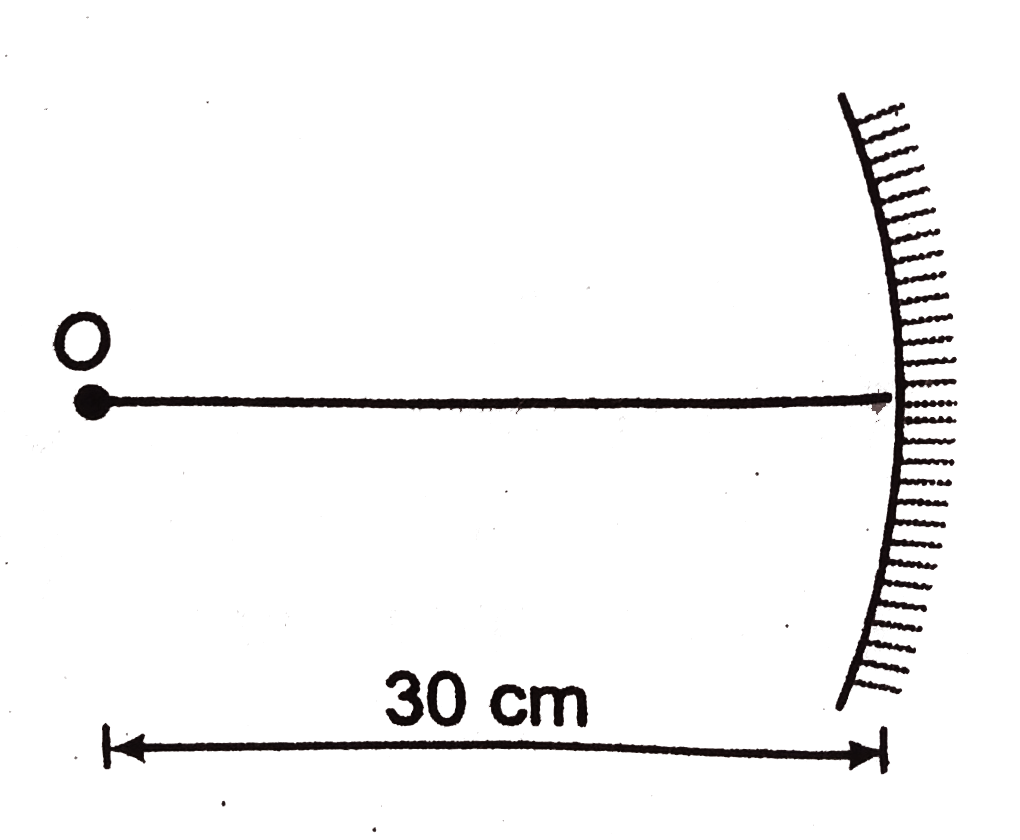
View SolutionA point object is placed on the principal axis of a concave mirror of focal length 20 cm. At this instant object is given a velocity v towards the axis (event-I) or perpendicular to axis (event-2). Then, speed of image
View Solution
