If the sum of two unit vectors is a unit vector, then the magnitude of their difference is
Let ˆa and ˆb be two unit vectors represented by sides OA and AB of a ΔOAB.
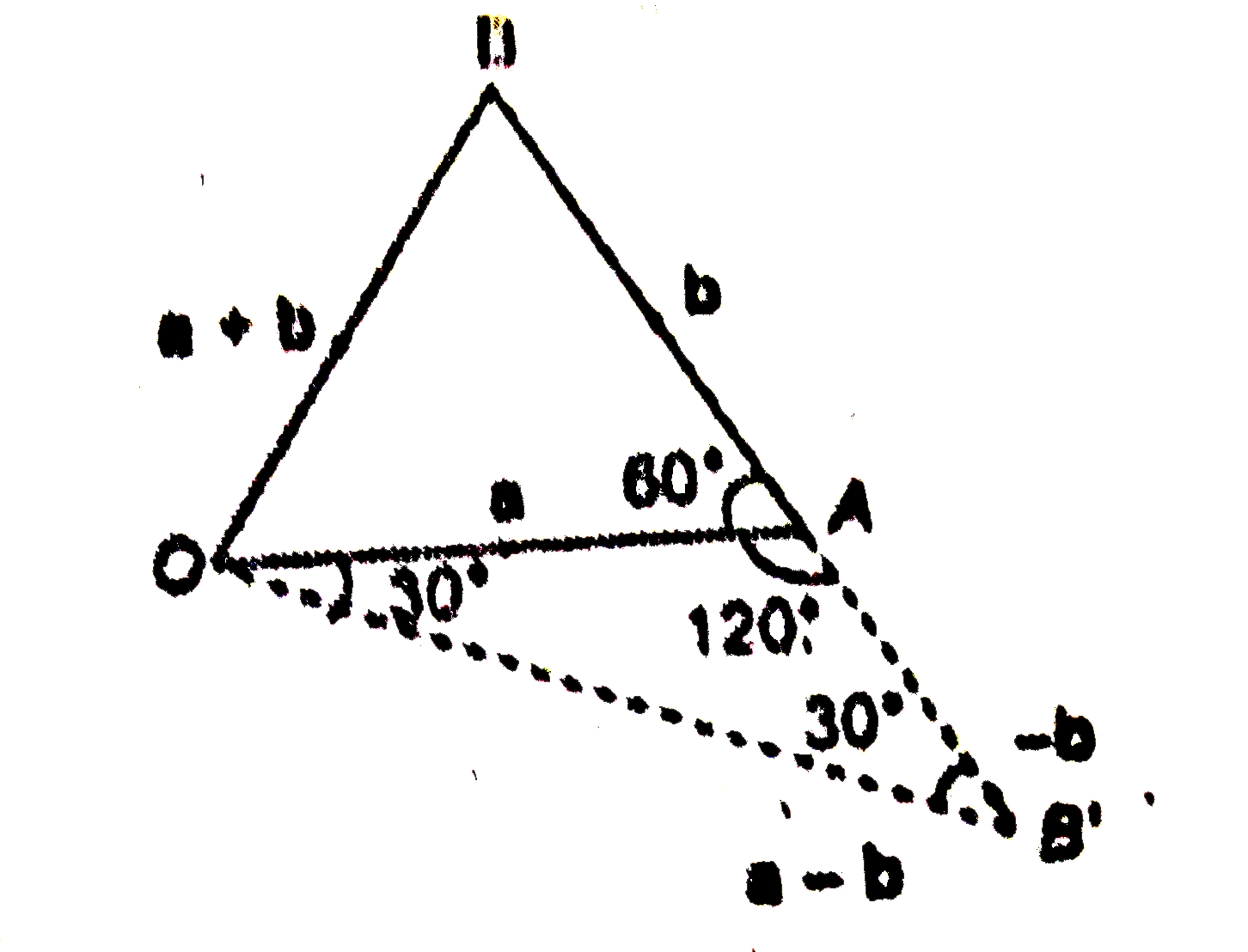
Then, OA=ˆa,AB=ˆb
OB=OA+AB=ˆa+ˆb (using triangle law of vector addition)
It is given that, |ˆa|=∣∣ˆb∣∣=∣∣ˆa+ˆb∣∣=1
⇒|OA|+|AB|=|OB|=1
ΔOAB is equilateral triangle.
Since, |OA|=|ˆa|=1=|=|−ˆb|=|AB'∣∣
therefore, ΔOAB' is a isosceles triangle.
⇒∠AB'O=∠AOB'=30∘
⇒∠BOB'=∠BOA+∠AOB'=60∘+30∘=90∘ (since, ΔBOB' is right angled)
∴In ΔBOB', we have
|BB'|=|OB|2+|OB'|2
=∣∣ˆa+ˆb∣∣2+∣∣ˆa−ˆb∣∣2
22=12+∣∣ˆa−ˆb∣∣2
ˆa−ˆb∣=√3 . . . hence proved.
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 1
7 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 2
17 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
19 Videos
Similar Questions
If the sum of two unit vectors is a unit vector, show that magnitude of their difference is √3.
View SolutionAssertion: If the magnitude of the sum of two unit vectors is a unit vector, then magnitude of their differnce is √3 Reason: |→a|+∣∣→b∣∣=∣∣→a+→b∣∣ (A) Both A and R are true and R is the correct explanation of A (B) Both A and R are true R is not te correct explanation of A (C) A is true but R is false. (D) A is false but R is true.
View SolutionIf the sum of two unit vectors is a unit vector, prove that the magnitude of their difference is √3.
View SolutionIf the sum of two unit vectors is a unit vector prove that the magnitude of their difference is √3.
View SolutionIf sum of two unit vectors is a unit vector; prove that the magnitude of their difference is √3
View SolutionIf the sum of two unit vectors is also a unit vector. Then magnituce of their difference and angle between the two given unit vectors is
View SolutionIf the sum of two unit vectors is a unit vector, then magnitude of difference is-
View SolutionIf the difference of two unit vectors is also a vector of unit magnitude, the magnitude of the sum of the two unit vectors is
View SolutionIf magnitude of sum of two unit vectors is √2 then find the magnitude of subtraction of these unit vectors.
View SolutionIf the magnitude of sum of two vectors is equal to the magnitude of difference of the two vector, the angle between these Vector is
View Solution