A doctor is called to see a sick child. The doctor knows (prior to the visit) that 90% of the sick children in that neighbourhood are sick with the flu, denoted by F, while 10% are sick with the measles, denoted by M. A well-known symptom of measles is a rash, denoted by R. The probability having a rash for a child sick with the measles is 0.95. however, occasionally children with the flu also develop a rash, with conditional probability 0.08. upon examination the child, the doctor finds a rash. The what is the probability that the child has the measles? 91/165 b. 90/163 c. 82/161 d. 95/167
89167
91167
93167
95167
To solve the problem, we will use Bayes' theorem to find the probability that the child has measles given that the child has a rash.
1. Identify the Given Probabilities:
- Probability of flu (F): P(F)=0.9
- Probability of measles (M): P(M)=0.1
- Probability of rash given measles: P(R|M)=0.95
- Probability of rash given flu: P(R|F)=0.08
2. Use Bayes' Theorem:
We need to find P(M|R), the probability that the child has measles given that the child has a rash. According to Bayes' theorem:
P(M|R)=P(M)⋅P(R|M)P(M)⋅P(R|M)+P(F)⋅P(R|F)
3. Substitute the Known Values:
Substitute the values we have into the formula:
P(M|R)=0.1⋅0.950.1⋅0.95+0.9⋅0.08
4. Calculate the Numerator:
Numerator=0.1⋅0.95=0.095
5. Calculate the Denominator:
Denominator=0.1⋅0.95+0.9⋅0.08=0.095+0.072=0.167
6. Final Calculation:
Now, substitute the numerator and denominator back into the equation:
P(M|R)=0.0950.167
7. Simplify the Fraction:
To express this as a fraction:
P(M|R)=95167
Conclusion:
The probability that the child has measles given that the child has a rash is 95167.
- Probability of flu (F): P(F)=0.9
- Probability of measles (M): P(M)=0.1
- Probability of rash given measles: P(R|M)=0.95
- Probability of rash given flu: P(R|F)=0.08
We need to find P(M|R), the probability that the child has measles given that the child has a rash. According to Bayes' theorem:
P(M|R)=P(M)⋅P(R|M)P(M)⋅P(R|M)+P(F)⋅P(R|F)
Substitute the values we have into the formula:
P(M|R)=0.1⋅0.950.1⋅0.95+0.9⋅0.08
Numerator=0.1⋅0.95=0.095
Denominator=0.1⋅0.95+0.9⋅0.08=0.095+0.072=0.167
Now, substitute the numerator and denominator back into the equation:
P(M|R)=0.0950.167
To express this as a fraction:
P(M|R)=95167
The probability that the child has measles given that the child has a rash is 95167.
Topper's Solved these Questions
- PROBABILITYBOOK - ARIHANT MATHS ENGLISHCHAPTER - PROBABILITYEXERCISE - Example
4 Videos
- PROBABILITYBOOK - ARIHANT MATHS ENGLISHCHAPTER - PROBABILITYEXERCISE - Exercise For Session 1
20 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
28 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
51 Videos
Similar Questions
Knowledge Check
A family has three children.Assuming that the probability of having a boy is 0.5, what is the probability that at least one child is a boy?
A0.875B0.67C0.5D0.375The vector of sleeping sickness' is :
Aa) house flyBb) sand flyCc) mosquitoDd) tsetse fly
How many times must a man toss a fair com so that the probability of having at least one head is more than 90%?
View SolutionWrite the causative agent and the most important symptom of the following disease:
Measles
View SolutionThe domain of the function f(x) is denoted by Df
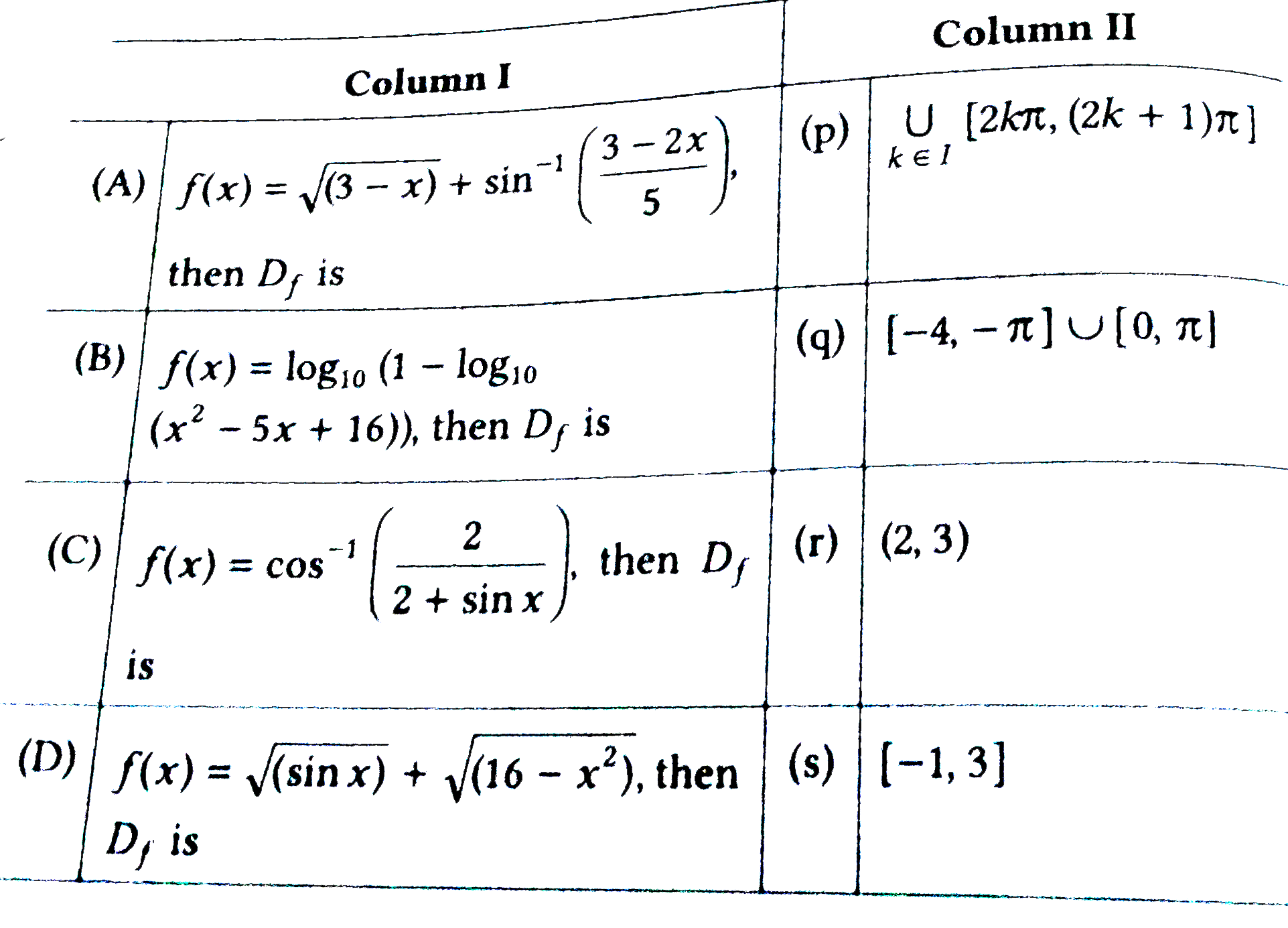
View SolutionA couple has two children. Find the probability that both the children are (i) males, if it is known that at least one of the children is male. (ii) females, if it is known that the elder child is a female.
View SolutionA couple has two children. Find the probability that both the children are (i) males, if it si known that at least one of the children is male. (ii) females, if it is known that the elder child is a female.
View SolutionIt is known that 10% of certain articles manufactured are defective. What is the probability that in a random sample of 12 such articles, 9 are defective?
View Solution
