PA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and .
On the basis of above information answer the questions .
value of sin2theta + cos4theta +sin5theta+tan7theta +cos 8theta
The correct Answer is:B
To solve the problem step by step, let's break it down:
Step 1: Understand the Geometry
We have a circle with a radius of 5 units. From point P, two tangents PA and PB are drawn to the circle. A line from point P intersects the points C and D such that PC = 5 units and PD = 15 units.
Step 2: Calculate CD
Since PD = 15 and PC = 5, we can find the length of CD:
CD=PD−PC=15−5=10 units
Step 3: Identify the Triangle
The points A and B are where the tangents touch the circle. The radius at points A and B is perpendicular to the tangents. Therefore, triangles PCA and PCB are right triangles.
Step 4: Use the Pythagorean Theorem
In triangle PCA, we can apply the Pythagorean theorem:
PA2+PC2=PO2
Where PO is the distance from point P to the center O of the circle. Since PA is a tangent to the circle, we know:
PA=√PO2−OA2
Given OA (the radius) = 5 units, we need to find PO.
Step 5: Find PO
From the triangle, we know:
PO=PC+OA=5+5=10 units
Step 6: Find angle θ
Using the right triangle PCA:
sin(θ/2)=OAPO=510=12
Thus,
θ/2=30∘⟹θ=60∘
Step 7: Calculate the Required Expression
Now we need to calculate:
sin(2θ)+cos(4θ)+sin(5θ)+tan(7θ)+cos(8θ)
Substituting θ=60∘:
- sin(2θ)=sin(120∘)=√32
- cos(4θ)=cos(240∘)=−12
- sin(5θ)=sin(300∘)=−√32
- tan(7θ)=tan(420∘)=tan(60∘)=√3
- cos(8θ)=cos(480∘)=cos(120∘)=−12
Step 8: Combine the Results
Now, we combine these values:
sin(2θ)+cos(4θ)+sin(5θ)+tan(7θ)+cos(8θ)=√32−12−√32+√3−12
Simplifying:
=(√32−√32+√3)+(−12−12)=√3−1
Final Answer
Thus, the value of the expression is:
√3−1
---
We have a circle with a radius of 5 units. From point P, two tangents PA and PB are drawn to the circle. A line from point P intersects the points C and D such that PC = 5 units and PD = 15 units.
Since PD = 15 and PC = 5, we can find the length of CD:
CD=PD−PC=15−5=10 units
The points A and B are where the tangents touch the circle. The radius at points A and B is perpendicular to the tangents. Therefore, triangles PCA and PCB are right triangles.
In triangle PCA, we can apply the Pythagorean theorem:
PA2+PC2=PO2
Where PO is the distance from point P to the center O of the circle. Since PA is a tangent to the circle, we know:
PA=√PO2−OA2
Given OA (the radius) = 5 units, we need to find PO.
From the triangle, we know:
PO=PC+OA=5+5=10 units
Using the right triangle PCA:
sin(θ/2)=OAPO=510=12
Thus,
θ/2=30∘⟹θ=60∘
Now we need to calculate:
sin(2θ)+cos(4θ)+sin(5θ)+tan(7θ)+cos(8θ)
Substituting θ=60∘:
- sin(2θ)=sin(120∘)=√32
- cos(4θ)=cos(240∘)=−12
- sin(5θ)=sin(300∘)=−√32
- tan(7θ)=tan(420∘)=tan(60∘)=√3
- cos(8θ)=cos(480∘)=cos(120∘)=−12
Now, we combine these values:
sin(2θ)+cos(4θ)+sin(5θ)+tan(7θ)+cos(8θ)=√32−12−√32+√3−12
Simplifying:
=(√32−√32+√3)+(−12−12)=√3−1
Thus, the value of the expression is:
√3−1
Topper's Solved these Questions
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - EXAMPLES ( Matching Type Questions )
1 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - EXAMPLES ( Subjective Type Examples )
2 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHS ENGLISHCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam
2 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
19 Videos
Similar Questions
PA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ∠APB=θ.
On the basis of above information answer the questions .
Area of ΔAPB is
View SolutionPA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ∠APB=θ.
On the basis of above information answer the questions .
Number of solution(s) of the equation logcosθ(x+2)=2+3log(x+2)sin(5θ2) is
View SolutionSolve cos4θ+sin5θ=2
View SolutionIf sin7θ=cos5θ, then general value of θ is:
View SolutionIf 5cosθ=6,sinθ, evalutate :
tanθ
View SolutionIf 5 tan θ=4, find the value of : 8sinθ−3cosθ8sinθ+2cosθ
View SolutionConsider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
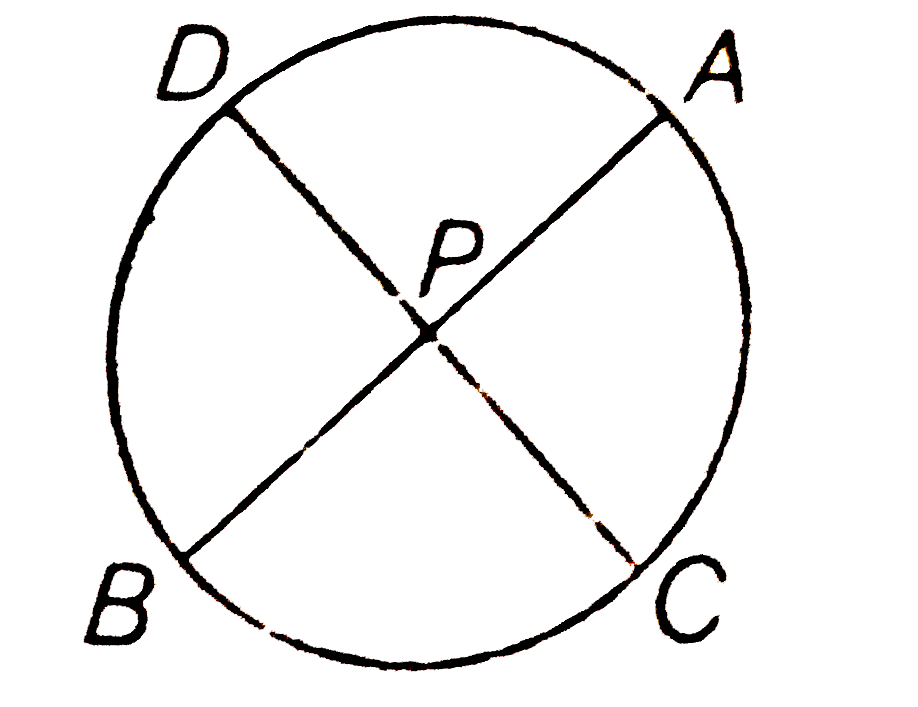
On the basis of above information , answer the questions
If PA=|cosθ+sinθ|andPB=|cosθ−sinθ|, then maximum value of (PC)(PD) , is equal to
View SolutionIf θ lies in the first quadrant and 5tanθ=4, then 5sinθ−3cosθsinθ+2cosθ=
View SolutionThe greater value of the function −5sinθ+12cosθ is
View SolutionIf cosecθ=√5 , find the value of :
2−sin2θ−cos2θ
View Solution
