Find periods for
(i)cos4x.(ii)sin3x.(iii)cos√x.(iv)cosx.
To find the periods of the given functions, we will analyze each function one by one.
1. Function (i): cos4x
The function can be expressed as cos4x. Here, n=4 (which is even).
- The period of cosnx for even n is π.
- Therefore, the period of cos4x is π.
2. Function (ii): sin3x
The function is sin3x. Here, n=3 (which is odd).
- The period of sinnx for odd n is 2π.
- Therefore, the period of sin3x is 2π.
3. Function (iii): cos(√x)
The function is cos(√x). To determine if this function is periodic, we check if there exists a period T such that:
cos(√x+T)=cos(√x)for all x.
- If we let T=1, then cos(√x+1) is not equal to cos(√x) for all x.
- In fact, since √x is not periodic (it continuously increases), cos(√x) does not repeat its values.
- Therefore, cos(√x) is not a periodic function.
4. Function (iv): cosx
The function is cosx. Here, n=1 (which is odd).
- The period of cosx is 2π.
- Therefore, the period of cosx is 2π.
Summary of Periods:
- (i) cos4x: Period = π
- (ii) sin3x: Period = 2π
- (iii) cos(√x): Not periodic
- (iv) cosx: Period = 2π
The function can be expressed as cos4x. Here, n=4 (which is even).
- The period of cosnx for even n is π.
- Therefore, the period of cos4x is π.
The function is sin3x. Here, n=3 (which is odd).
- The period of sinnx for odd n is 2π.
- Therefore, the period of sin3x is 2π.
The function is cos(√x). To determine if this function is periodic, we check if there exists a period T such that:
cos(√x+T)=cos(√x)for all x.
- If we let T=1, then cos(√x+1) is not equal to cos(√x) for all x.
- In fact, since √x is not periodic (it continuously increases), cos(√x) does not repeat its values.
- Therefore, cos(√x) is not a periodic function.
The function is cosx. Here, n=1 (which is odd).
- The period of cosx is 2π.
- Therefore, the period of cosx is 2π.
- (i) cos4x: Period = π
- (ii) sin3x: Period = 2π
- (iii) cos(√x): Not periodic
- (iv) cosx: Period = 2π
Topper's Solved these Questions
- FUNCTIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - FUNCTIONSEXERCISE - Exercise For Session 1
5 Videos
- FUNCTIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - FUNCTIONSEXERCISE - Exercise For Session 2
6 Videos
- ESSENTIAL MATHEMATICAL TOOLS BOOK - ARIHANT MATHS ENGLISHCHAPTER - ESSENTIAL MATHEMATICAL TOOLS EXERCISE - Exercise (Single Integer Answer Type Questions)
3 Videos
- GRAPHICAL TRANSFORMATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
10 Videos
Similar Questions
find the period of sin(x2)−cos(x3) is
View SolutionIf 4cos2x=3andx is an acute angle find the value of :
(i) x
(ii) cos2x+cot2x
(iii) cos 3x
(iv) sin 2x
View SolutionIntegrate with respect to x: i) sin2x, ii) cos3x , iii) sin2xcos3x, iv) 1√x+3−√x+2
View SolutionWhich of the following functions are decreasing on (0, π/2) ? (i) cosx (ii) cos2x (iii) tanx (iv) cos3x
View SolutionWhich of the following functions are decreasing on (0, π/2) ? (i) cosx (ii) cos2x (iii) tanx (iv) cos3x
View SolutionFind the derivative of the following functions:(i) sinxcosx (ii) secx (iii) 5secx+4cosx(iv) cosecx (v) 3cotx+5cosecx
View SolutionWhich of the following functions is not periodic?
(a) |sin3x|+sin2x (b) cos√x+cos2x
(c ) cos4x+tan2x (d ) cos2x+sinx
View SolutionExpress sin−1x in terms of (i) cos−1√1−x2 (ii) tan−1x√1−x2 (iii) cot−1√1−x2x
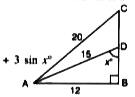
View SolutionIn the given figure ∠B=90∘and∠ADB=x∘, Find :
(i) sin∠CAB
(ii)cos2C∘+sin2C∘
(iii) tanx∘−cosx∘+3sinx∘
View SolutionThe period of sin(π4)x+cos(π2)x+cos(π3)x is
View Solution
