Suppose f(x)=(x+1)2 for x≥−1. If g(x) is the function whose graph is the reflection of the graph of f(x) with respect to the line y = x, then g(x) equals
1−√x−1,x≥0
1(x+1)2,x>−1
√x+1,x≥−1
√x−1,x≥0
The correct Answer is:D
Step by step video & image solution for Suppose f(x)=(x+1)^(2) " for " x ge -1. If g(x) is the function whose graph is the reflection of the graph of f(x) with respect to the line y = x, then g(x) equals by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FUNCTIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - FUNCTIONSEXERCISE - FUNCTION EXERCISE 8:Questions Asked in Previous 10 Years Exams
1 Videos
- FUNCTIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - FUNCTIONSEXERCISE - FUNCTION EXERCISE 7: Subjective Type Questions
1 Videos
- ESSENTIAL MATHEMATICAL TOOLS BOOK - ARIHANT MATHS ENGLISHCHAPTER - ESSENTIAL MATHEMATICAL TOOLS EXERCISE - Exercise (Single Integer Answer Type Questions)
3 Videos
- GRAPHICAL TRANSFORMATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
10 Videos
Similar Questions
Suppose f(x)=(x+1)2 forx≥−1. If g(x) is the function whose graph is the reflection of the graph of f(x) with respect to the line y=x, then g(x) equal. (a)1−√x−1,x≥0 (b) 1(x+1)2,x>−1 (c)√x+1,x≥−1 (d) √x−1,x≥0
View SolutionSuppose f(x)=(x+1)2forx≥−1. If g(x) is the function whose graph is the reflection of the graph of f(x) with respect to the line y=x, then g(x) equal. a−√x−1,x≥0 (b) 1(x+1)2,x≻1 √x+1,x≥−1 (d) √x−1,x≥0
View SolutionIf g(x) is a curve which is obtained by the reflection of f(x)=ex−e−x2 then by the line y=x then
View SolutionIf g(x) is a curve which is obtained by the reflection of f(x)=ex−e−x2 then by the line y=x then
View SolutionIf the graph of the function y = f(x) is symmetrical about the line x = 2, then
View SolutionConsider the following function f whose graph is given below.
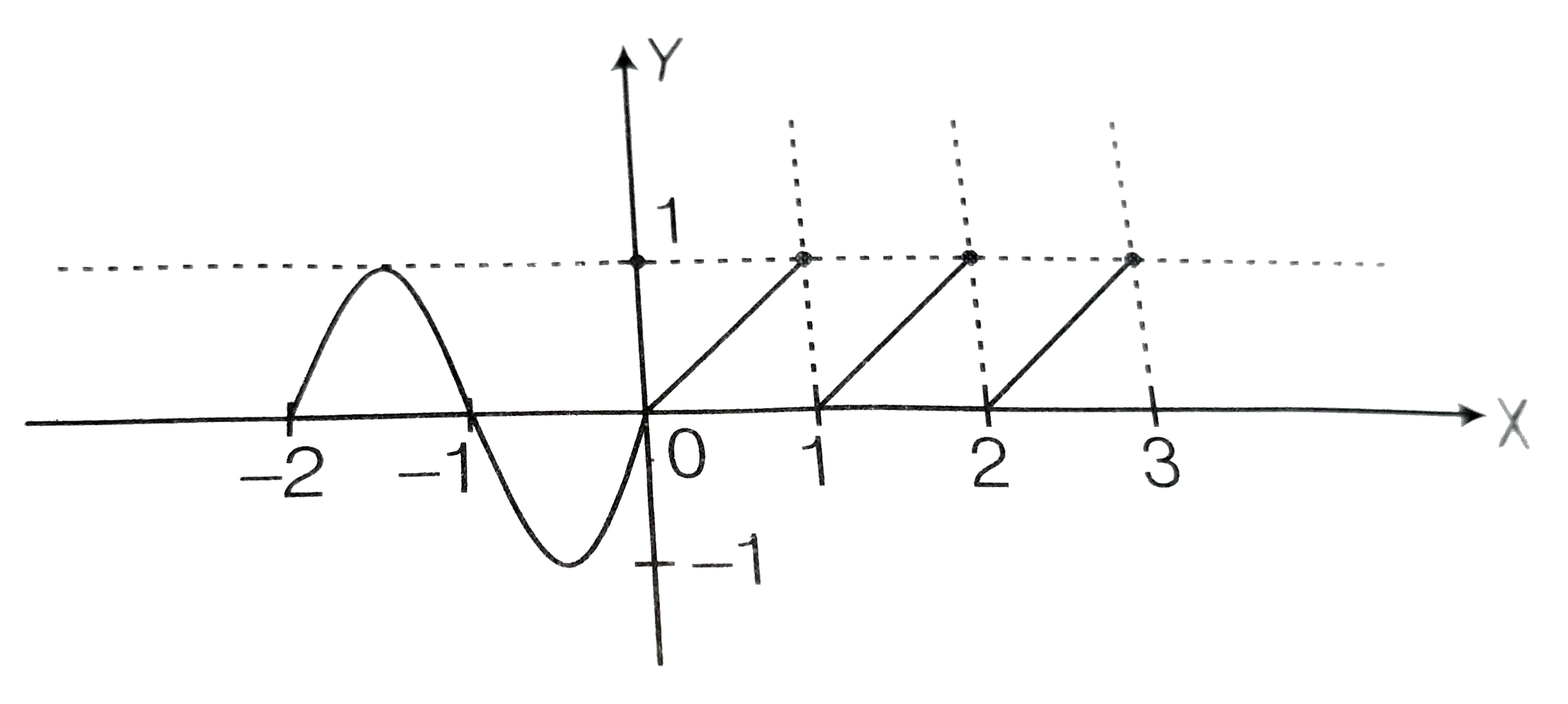
Draw the graph of following functions.
(g) 2f(x)
View SolutionLet f be a differential function such that f(x)=f(2−x) and g(x)=f(1+x) then (1) g(x) is an odd function (2) g(x) is an even function (3) graph of f(x) is symmetrical about the line x= 1 (4) f'(1)=0
View SolutionLet f be a differential function such that f(x)=f(2−x) and g(x)=f(1+x) then (1) g(x) is an odd function (2) g(x) is an even function (3) graph of f(x) is symmetrical about the line x= 1 (4) f'(1)=0
View SolutionConsider the following function f whose graph is given below.
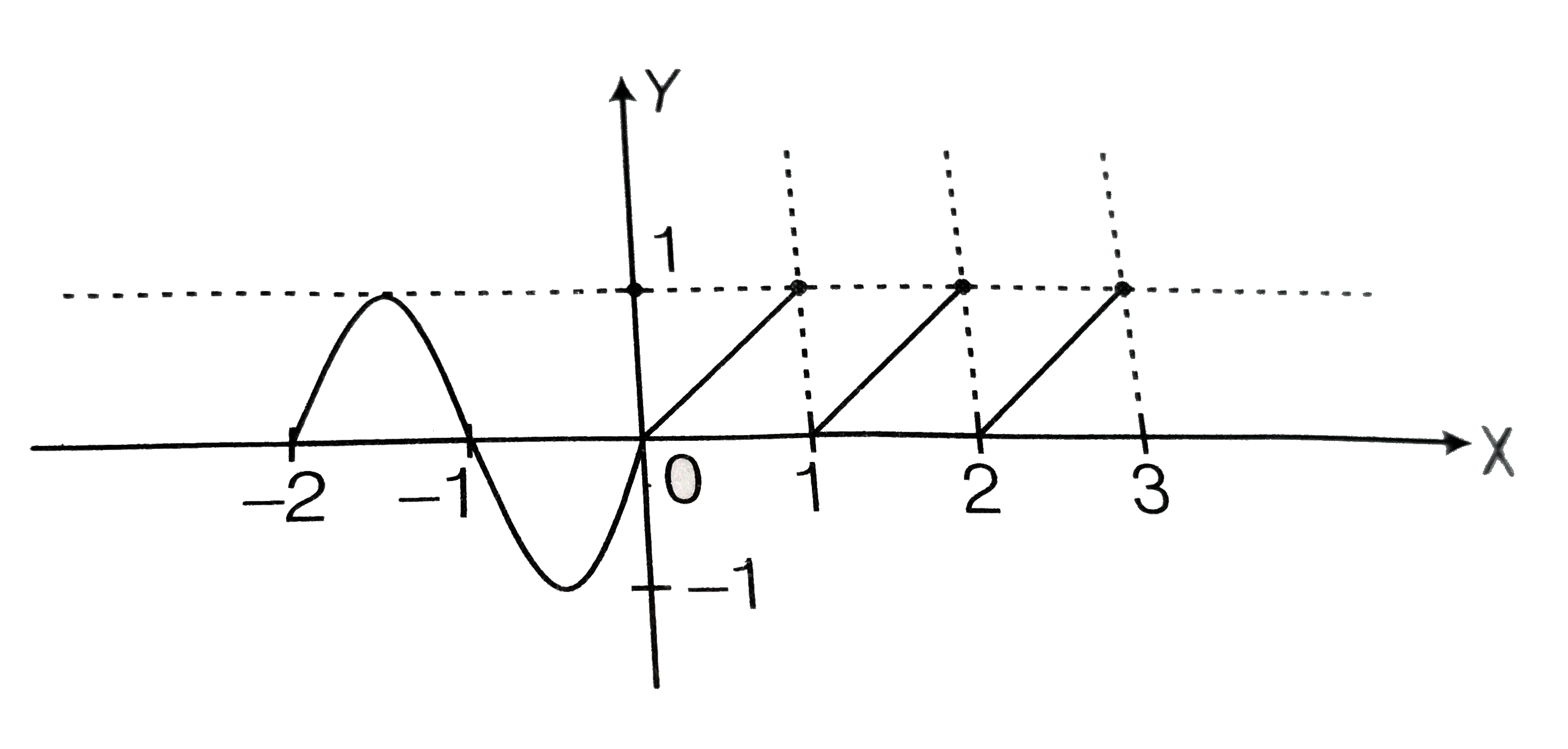
Draw the graph of following functions.
(g) f(x−[x])
View SolutionConsider a function f(x)=xx,∀x∈[1,∞). If g(x) is the inverse function of f(x), then the value of g'(4) is equal to
View Solution
