Let A=⎡⎢⎣abcpqr111⎤⎥⎦andB=A2
If (a−b)2+(p−q)2=25,(b−c)2+(q−r)2=36and(c−a)2+(r−p)2=49, then det B is
192
864
2456
25×36×47
The correct Answer is:B
To solve the problem step by step, we will first analyze the given information and then calculate the required determinant.
Step 1: Understand the Given Information
We have a matrix A defined as:
A=⎛⎜⎝abcpqr111⎞⎟⎠
We also know that B=A2.
We are given three equations:
1. (a−b)2+(p−q)2=25
2. (b−c)2+(q−r)2=36
3. (c−a)2+(r−p)2=49
Step 2: Calculate the Lengths of the Sides of the Triangle
From the equations, we can interpret them as the lengths of the sides of a triangle formed by the points (a,p), (b,q), and (c,r).
- Length a:
a=√(a−b)2+(p−q)2
From the first equation, we have:
a=√25=5
- Length b:
b=√(b−c)2+(q−r)2
From the second equation, we have:
b=√36=6
- Length c:
c=√(c−a)2+(r−p)2
From the third equation, we have:
c=√49=7
Step 3: Calculate the Semi-Perimeter s
The semi-perimeter s of the triangle is given by:
s=a+b+c2=5+6+72=9
Step 4: Calculate the Area of the Triangle Using Heron's Formula
Using Heron's formula:
Area=√s(s−a)(s−b)(s−c)
Substituting the values we found:
Area=√9(9−5)(9−6)(9−7)=√9⋅4⋅3⋅2=√216=6√6
Step 5: Relate the Area to the Determinant of Matrix A
The area of the triangle can also be expressed in terms of the determinant of matrix A:
Area=12⋅det(A)
Thus, we have:
6√6=12⋅det(A)
From this, we can find:
det(A)=12√6
Step 6: Calculate the Determinant of Matrix B
Since B=A2, we have:
det(B)=det(A)2
Substituting the value of det(A):
det(B)=(12√6)2=144⋅6=864
Final Answer
Thus, the value of det(B) is:
det(B)=864
We have a matrix A defined as:
A=⎛⎜⎝abcpqr111⎞⎟⎠
We also know that B=A2.
1. (a−b)2+(p−q)2=25
2. (b−c)2+(q−r)2=36
3. (c−a)2+(r−p)2=49
From the equations, we can interpret them as the lengths of the sides of a triangle formed by the points (a,p), (b,q), and (c,r).
a=√(a−b)2+(p−q)2
From the first equation, we have:
a=√25=5
b=√(b−c)2+(q−r)2
From the second equation, we have:
b=√36=6
c=√(c−a)2+(r−p)2
From the third equation, we have:
c=√49=7
The semi-perimeter s of the triangle is given by:
s=a+b+c2=5+6+72=9
Using Heron's formula:
Area=√s(s−a)(s−b)(s−c)
Substituting the values we found:
Area=√9(9−5)(9−6)(9−7)=√9⋅4⋅3⋅2=√216=6√6
The area of the triangle can also be expressed in terms of the determinant of matrix A:
Area=12⋅det(A)
Thus, we have:
6√6=12⋅det(A)
From this, we can find:
det(A)=12√6
Since B=A2, we have:
det(B)=det(A)2
Substituting the value of det(A):
det(B)=(12√6)2=144⋅6=864
Thus, the value of det(B) is:
det(B)=864
Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (More Than One Correct Option Type Questions)
23 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Statement I And Ii Type Questions)
11 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - PROPERTIES AND SOLUTIONS OF TRIANGLES EXERSISE 1: SINGLE OPTION CORRECT TYPE QUESTIONS
1 Video
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
51 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
Knowledge Check
Δ=∣∣ ∣ ∣∣pqrp+2aq+2br+2cabc∣∣ ∣ ∣∣ then
Aa) Δ=0Bb) Δ=abcCc) Δ=pqrDd) None of these
Show that : (4pq+3q)2−(4qp−3q)2=48pq2 (a−b)(a+b)+(b−c)(b+c)+(c−a)(c+a)=0
View SolutionIf pa+qb+rc=1 and ap+bq+cr=0, then the value of p2a2+q2b2+r2c2 is:
View SolutionLet A = {p, q, r} and B = {1, 2}. Represent
(i) A×B
(ii) B×B
View SolutionThe roots of the equation (q−r)x2+(r−p)x+p−q=0 are (A) r−pq−r,1 (B) p−qq−r,1 (C) q−rp−q,1 (D) r−pp−q,1
View SolutionWithout, prove that :
|(1+b,b+c,c+a),(p+q,q+r,r+p),(x+y,y+z,z+x),|=2∣∣ ∣ ∣∣abcpqrxyz∣∣ ∣ ∣∣
View SolutionIf ∣∣ ∣ ∣∣a−bb−cc−ax−yy−zz−xp−qq−rr−p∣∣ ∣ ∣∣=m∣∣ ∣ ∣∣cabzxyrpq∣∣ ∣ ∣∣, then m=
View SolutionMatch te columns
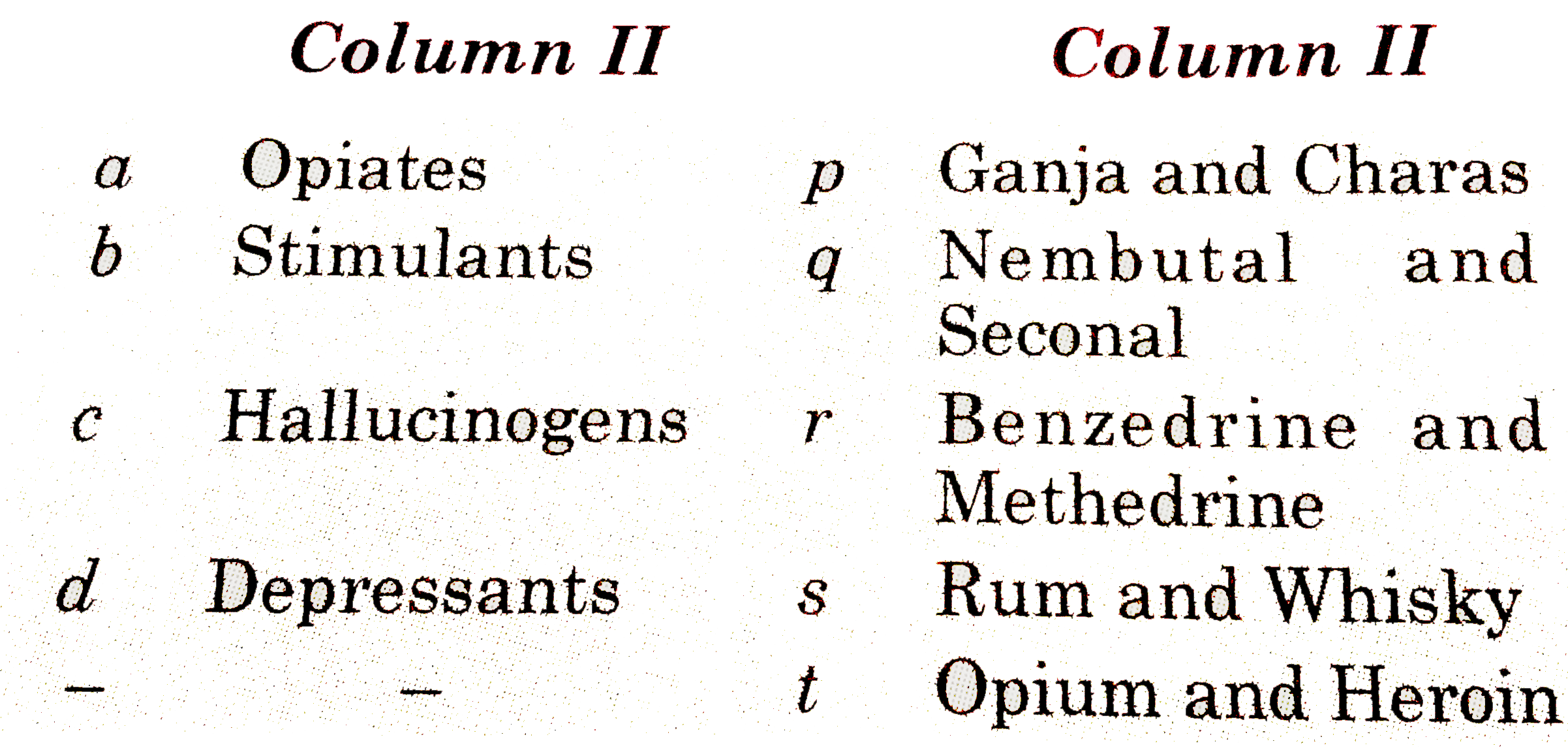
(1) a-s, b-t, c-r, d-p
(2) a-t, b-q, c-r, d-p
(3) a-t, b-r, c-q, d-p
(4) a-t, b-r, c-p, d-q
View Solution
