Let L1,L2,L3 be three straight lines a plane and n be the number of circles touching all the lines . Find the value of n.
The correct Answer is:A→p;B→p,q;C→p,rD→p,r,s
To solve the problem of finding the number of circles that can touch three straight lines L1,L2,L3 in a plane, we can follow these steps:
1. Understanding the Configuration:
We start by visualizing the three lines L1,L2,L3. These lines can be arranged in various ways (intersecting, parallel, etc.), but for the purpose of this problem, we will assume they are not parallel and intersect each other.
Hint: Draw three non-parallel lines that intersect each other to visualize the scenario.
2. Identifying Possible Circles:
When three lines intersect, they create different regions in the plane. We need to determine how many distinct circles can be drawn that touch all three lines.
Hint: Consider the angles formed by the intersections of the lines and how circles can be positioned in relation to these angles.
3. Positioning the Circles:
For three lines, there are typically four distinct regions where circles can be placed:
- One circle can be placed in the interior region formed by the intersection of the three lines.
- Three additional circles can be placed in the exterior regions, one in each of the three corners created by the angles between the lines.
Hint: Visualize or sketch the circles in each of these regions to ensure they touch all three lines.
4. Counting the Circles:
After analyzing the possible placements, we find that:
- 1 circle can be placed in the interior region.
- 3 circles can be placed in the exterior regions.
Therefore, the total number of circles n that can touch all three lines is 1+3=4.
Hint: Sum the number of circles from both interior and exterior placements to arrive at the final count.
5. Conclusion:
Thus, the value of n, the number of circles that can touch all three lines L1,L2,L3, is 4.
Final Answer: n=4
We start by visualizing the three lines L1,L2,L3. These lines can be arranged in various ways (intersecting, parallel, etc.), but for the purpose of this problem, we will assume they are not parallel and intersect each other.
When three lines intersect, they create different regions in the plane. We need to determine how many distinct circles can be drawn that touch all three lines.
For three lines, there are typically four distinct regions where circles can be placed:
- One circle can be placed in the interior region formed by the intersection of the three lines.
- Three additional circles can be placed in the exterior regions, one in each of the three corners created by the angles between the lines.
After analyzing the possible placements, we find that:
- 1 circle can be placed in the interior region.
- 3 circles can be placed in the exterior regions.
Thus, the value of n, the number of circles that can touch all three lines L1,L2,L3, is 4.
Topper's Solved these Questions
- THE STRAIGHT LINESBOOK - ARIHANT MATHS ENGLISHCHAPTER - THE STRAIGHT LINESEXERCISE - Exercise (Statement I And Ii Type Questions)
8 Videos
- THE STRAIGHT LINESBOOK - ARIHANT MATHS ENGLISHCHAPTER - THE STRAIGHT LINESEXERCISE - Exercise (Subjective Type Questions)
8 Videos
- THE STRAIGHT LINESBOOK - ARIHANT MATHS ENGLISHCHAPTER - THE STRAIGHT LINESEXERCISE - Exercise (Single Integer Answer Type Questions)
9 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
- THEORY OF EQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
35 Videos
Similar Questions
Let L1=0andL2=0 be two intarecting straight lines. Then the number of points, whose distacne from L1 is 2 units and from L2 2 units is
View SolutionLet L be the set of all straight lines in plane. l1 and l2 are two lines in the set. R1,R2 and R3 are defined relations.
(i) l1R1l2:l1 is parallel to l2
(ii) l1R2l2:l1 is perpendicular to l2
(iii) l1R3l2:l1 intersects l2
Then which of the following is true ?
View Solution
Knowledge Check
Line l is shown in the xy-plane below.
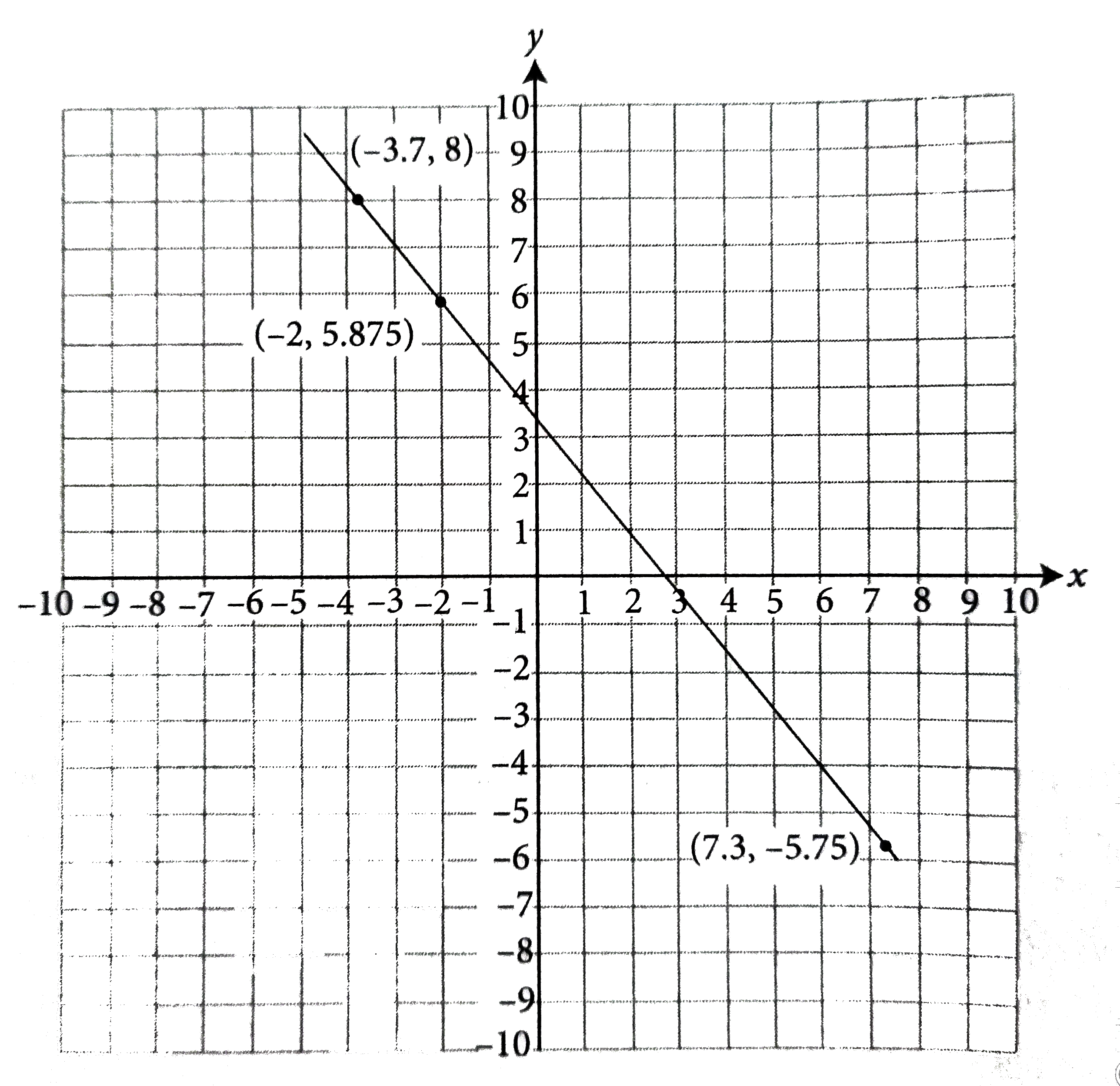
What is the slope of line l?A−1.25B0.80C−0.80D1.25
Let L1 be a straight line passing through the origin and L2 be the straight line x+y=1 if the intercepts made by the circle x2+y2−x+3y=0 on L1 and L2 are equal, then which of the following equations can represent L1?
View SolutionLet C:y=x2−3,D:y=kx2 be two parabolas and
L1 : x=a,L2:x=1(a≠0) be two straight lines.
IF the line L1 meets the parabola C at a point B on the line L2, other than A, then a may be equal to
View SolutionIf l,l1,l2andl3 be respectively the radii of the in-circle and the three escribed circles of a ΔABC, then find l1l2l3−l(l1l2+l2l3+l1l3).
View SolutionLet L be the set of all straight lines drawn in a plane, prove that the relation 'is perpendicular to' on L is not transitive.
View SolutionLet L be the set of all lines in a plane and R be the relation in L defined as R={(L1,L2):L1(is perpendicular to L2} Show that R is symmetric but neither reflexive nor transitive.
View Solutiont1,t2,t3 are lengths of tangents drawn from a point (h,k) to the circles x2+y2=4,x2+y2−4=0andx2+y2−4y=0 respectively further, t41=t22 t23+16. Locus of the point (h,k) consist of a straight line L1 and a circle C1 passing through origin. A circle C2 , which is equal to circle C1 is drawn touching the line L1 and the circle C1 externally.
Equation of L1 is
View SolutionLet L be the set of all lines in XY -plane and R be the relation in L defined as R={(L1,L2):L1 is parallel to L2} . Show that R is an equivalence relation. Find the set of all lines related to the line y=2x+4 .
View Solution
