Find the value of
(i)sin22∘30' (ii) cos 22∘30' (iii) tan 22∘30'
The correct Answer is:(i) √2−12√2 (ii) ⎷(√2+1)2√2 (iii) (√2−1)
To find the values of sin22∘30′, cos22∘30′, and tan22∘30′, we can follow these steps:
Step 1: Convert 22 degrees 30 minutes to decimal degrees
30 minutes is equal to 3060=0.5 degrees. Therefore:
22∘30′=22.5∘
Step 2: Calculate sin22∘30′
We will use the identity:
sin2θ=1−cos2θ2
Here, θ=22.5∘ so 2θ=45∘. Thus:
sin222.5∘=1−cos45∘2
Since cos45∘=1√2:
sin222.5∘=1−1√22
=√2−12√2
So:
sin22.5∘=√√2−12√2
Step 3: Calculate cos22∘30′
We will use the identity:
cos2θ=1+cos2θ2
Using θ=22.5∘:
cos222.5∘=1+cos45∘2
Substituting cos45∘=1√2:
cos222.5∘=1+1√22
=√2+12√2
Thus:
cos22.5∘=√√2+12√2
Step 4: Calculate tan22∘30′
Using the identity:
tanθ=sinθcosθ
We have:
tan22.5∘=sin22.5∘cos22.5∘
Substituting the values we found:
tan22.5∘=√√2−12√2√√2+12√2
This simplifies to:
tan22.5∘=√√2−1√2+1
Final Results
1. sin22∘30′=√√2−12√2
2. cos22∘30′=√√2+12√2
3. tan22∘30′=√√2−1√2+1
30 minutes is equal to 3060=0.5 degrees. Therefore:
22∘30′=22.5∘
We will use the identity:
sin2θ=1−cos2θ2
Here, θ=22.5∘ so 2θ=45∘. Thus:
sin222.5∘=1−cos45∘2
Since cos45∘=1√2:
sin222.5∘=1−1√22
=√2−12√2
So:
sin22.5∘=√√2−12√2
We will use the identity:
cos2θ=1+cos2θ2
Using θ=22.5∘:
cos222.5∘=1+cos45∘2
Substituting cos45∘=1√2:
cos222.5∘=1+1√22
=√2+12√2
Thus:
cos22.5∘=√√2+12√2
Using the identity:
tanθ=sinθcosθ
We have:
tan22.5∘=sin22.5∘cos22.5∘
Substituting the values we found:
tan22.5∘=√√2−12√2√√2+12√2
This simplifies to:
tan22.5∘=√√2−1√2+1
1. sin22∘30′=√√2−12√2
2. cos22∘30′=√√2+12√2
3. tan22∘30′=√√2−1√2+1
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Solved Examples : Single Option Correct Type Questions
2 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise For Session 1
10 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
9 Videos
Similar Questions
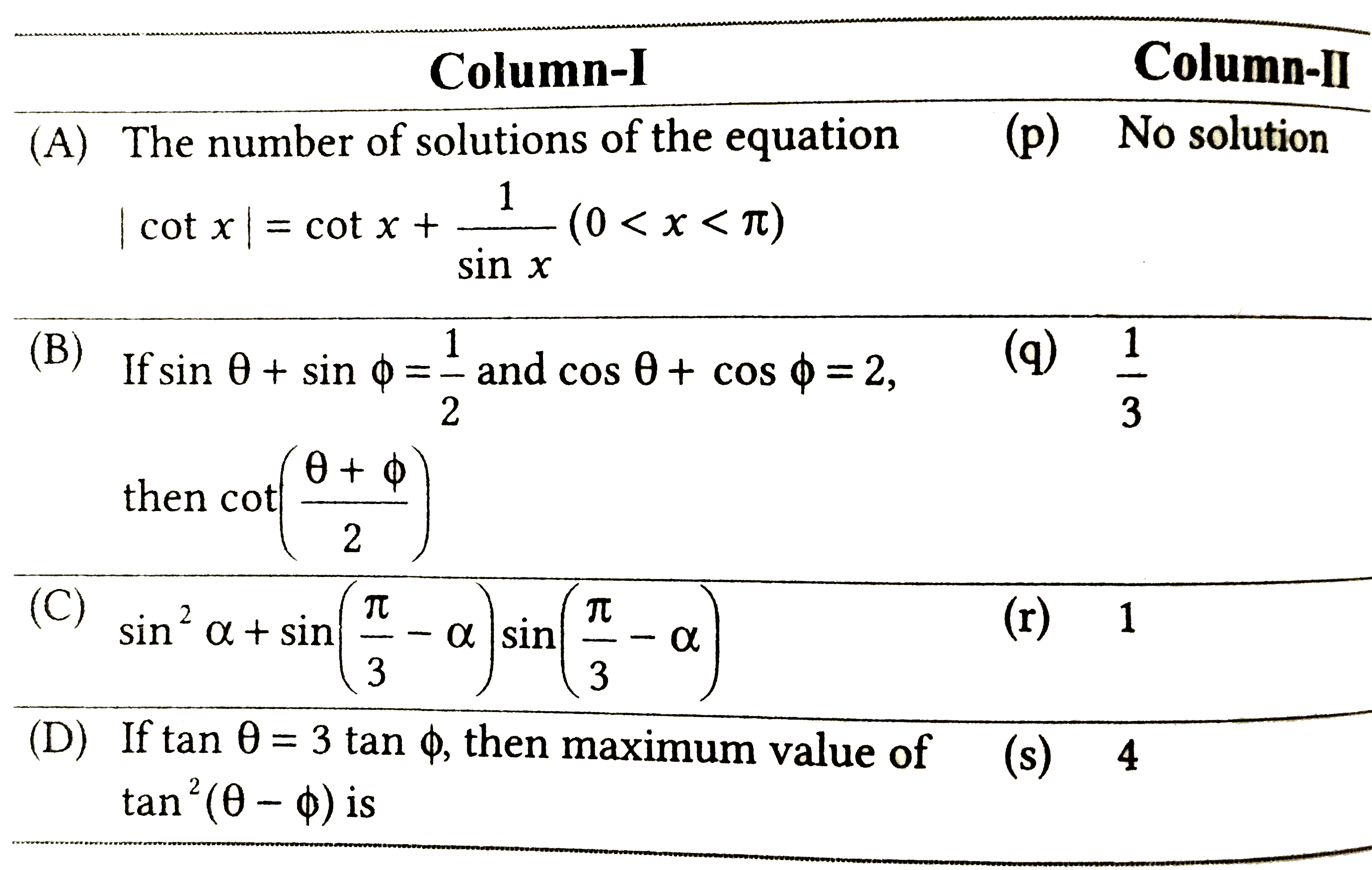
Match the statement of Column I with values of Column II.
View SolutionThe difference between two acute angles of a right angle triangle 3π10 rad. Find the angles in degree.
View SolutionLet S={x∈(−π,π):x≠0,±π2}. The sum of all distinct solutions of the equation √2secx+cosecx+2(tanx−cotx)
=0 in the set S is equal to
View SolutionIf vectors −−→AB=−3ˆi+4ˆkand−−→AC=5ˆi−2ˆj+4ˆk are the sides of a ΔABC, then the length of the median throught A is
View Solution22 at b 30 Find the values of a and b if 35-26
View SolutionThe value of sin222∘+sin268∘+cot230∘ is
View SolutionFind the value of
(i)sin22∘30' (ii) cos 22∘30' (iii) tan 22∘30'
View Solutionमान ज्ञात कीजिए
sin221∘2 (ii)cos221∘2 (iii)tan1421∘2
View Solutionनिम्न को सिद्ध कीजिए ।
tan22∘30'=√2−1
View SolutionUsing the formula tanA=sin2A1+cos2A,find the values of tan75∘ and cot22∘30
View SolutionFind the value of tan 22∘30’
View SolutionFind the value of tan22∘30'.
View SolutionThe value of sin222∘+sin268∘+cot230∘ is
View Solutionনিম্নোক্তটির বৃত্তীয় মান নির্ণয় করোঃ 22∘30'
View SolutionFind the value of tan(22∘30').
View Solution
