For , let ,
where
[For example: and
]
On the basis of above information, answer the following questions :
If , then the value of sin x is expressed as , then (p+q) equals
(a)3
(b)4
(c)7
(d)9
The correct Answer is:C
To solve the problem step by step, we start with the given expression for Pmn(x):
Step 1: Write the expressions for P34(x) and P22(x)
Given:
P34(x)=3logcosx(sinx)+4logcosx(cotx)
P22(x)=2logcosx(sinx)+2logcosx(cotx)
Step 2: Set the two expressions equal to each other
Since we know that P34(x)=P22(x), we can write:
3logcosx(sinx)+4logcosx(cotx)=2logcosx(sinx)+2logcosx(cotx)
Step 3: Rearrange the equation
Rearranging the equation gives:
3logcosx(sinx)−2logcosx(sinx)+4logcosx(cotx)−2logcosx(cotx)=0
This simplifies to:
logcosx(sinx)+2logcosx(cotx)=0
Step 4: Use the properties of logarithms
Using the property of logarithms, we can combine the logs:
logcosx(sinx)+logcosx(cot2x)=0
This can be rewritten as:
logcosx(sinx⋅cot2x)=0
Step 5: Exponentiate to eliminate the logarithm
Exponentiating both sides gives:
sinx⋅cot2x=1
Step 6: Substitute cotx
Recall that cotx=cosxsinx, so substituting gives:
sinx⋅(cos2xsin2x)=1
This simplifies to:
cos2xsinx=1
Step 7: Rearranging the equation
Rearranging gives:
cos2x=sinx
Step 8: Use the Pythagorean identity
Using the identity cos2x=1−sin2x, we can substitute:
1−sin2x=sinx
Step 9: Rearranging the equation
Rearranging gives:
sin2x+sinx−1=0
Step 10: Solve the quadratic equation
Using the quadratic formula sinx=−b±√b2−4ac2a:
Here, a=1,b=1,c=−1:
sinx=−1±√12−4⋅1⋅(−1)2⋅1=−1±√1+42=−1±√52
Step 11: Determine the valid solution
Since 0<x<π2, we need sinx to be positive. Thus, we take:
sinx=−1+√52
Step 12: Express in the required form
We can express sinx as:
sinx=√5−12
Here, p=2 and q=5.
Step 13: Calculate p+q
Thus, p+q=2+5=7.
Final Answer:
7
P34(x)=3logcosx(sinx)+4logcosx(cotx)
P22(x)=2logcosx(sinx)+2logcosx(cotx)
3logcosx(sinx)+4logcosx(cotx)=2logcosx(sinx)+2logcosx(cotx)
3logcosx(sinx)−2logcosx(sinx)+4logcosx(cotx)−2logcosx(cotx)=0
This simplifies to:
logcosx(sinx)+2logcosx(cotx)=0
logcosx(sinx)+logcosx(cot2x)=0
This can be rewritten as:
logcosx(sinx⋅cot2x)=0
sinx⋅cot2x=1
sinx⋅(cos2xsin2x)=1
This simplifies to:
cos2xsinx=1
cos2x=sinx
1−sin2x=sinx
sin2x+sinx−1=0
Here, a=1,b=1,c=−1:
sinx=−1±√12−4⋅1⋅(−1)2⋅1=−1±√1+42=−1±√52
sinx=−1+√52
sinx=√5−12
Here, p=2 and q=5.
7
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBook:ARIHANT MATHS ENGLISHChapter:TRIGONOMETRIC FUNCTIONS AND IDENTITIESExercise:Exercise (Matching Type Questions)
Explore 3 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBook:ARIHANT MATHS ENGLISHChapter:TRIGONOMETRIC FUNCTIONS AND IDENTITIESExercise:Exercise (Single Integer Answer Type Questions)
Explore 24 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBook:ARIHANT MATHS ENGLISHChapter:TRIGONOMETRIC FUNCTIONS AND IDENTITIESExercise:Exercise (Statement I And Ii Type Questions)
Explore 11 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBook:ARIHANT MATHS ENGLISHChapter:TRIGONOMETRIC EQUATIONS AND INEQUATIONSExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 12 Videos
- VECTOR ALGEBRABook:ARIHANT MATHS ENGLISHChapter:VECTOR ALGEBRAExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 9 Videos
Similar Questions
Knowledge Check
∫(sin(logx)+cos(logx)dx is equal to
Asin(logx)+CBcos(logx)+CCxcos(logx)+CDxsin(logx)+C
If logsinx(cosx)=12, where x∈(0,π2), then the value of sinx is equal to-
View SolutionSolve log|sinx|(1+cosx)=2.
View SolutionThe value of ∫π/20sin8xlogcotxcos2xdx, is
View SolutionConsider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
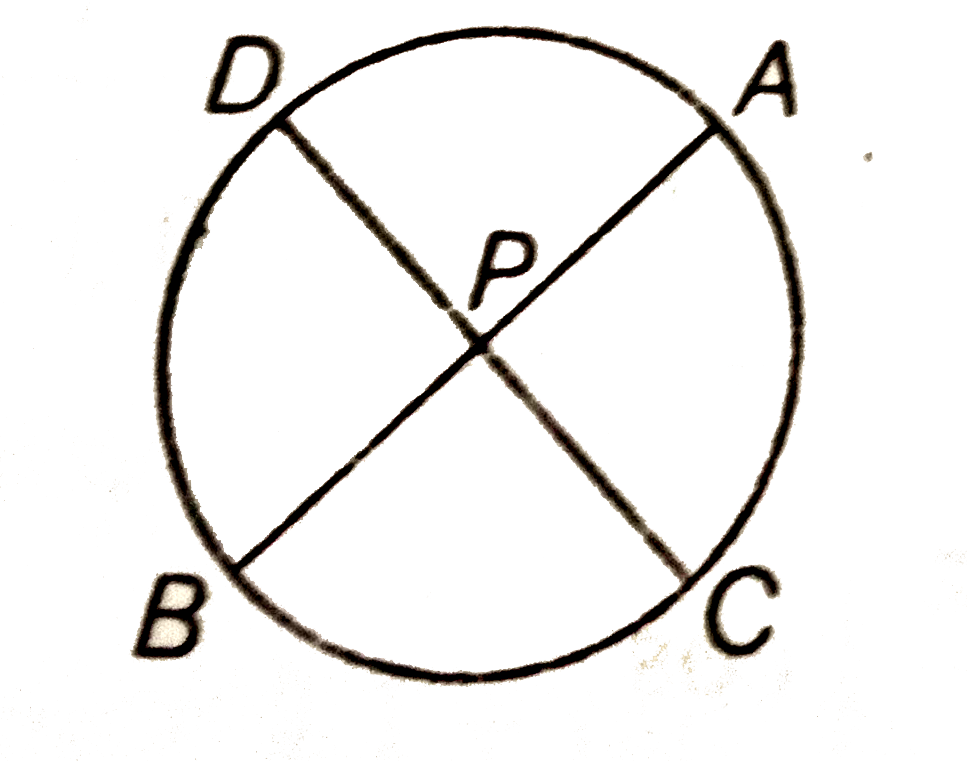
On the basis of above information , answer the questions
If logPAx=2,logPBx=3,logxPC=4, then logPD x is equal to
View SolutionRange of f(x)=log√10(√5(2sinx+cosx)+5) is :
View SolutionThe solution of the equation
logcosxsinx+logsinxcosx=2 is given by
View SolutionLet x∈(0,π2) and log24sinx(24cosx)=32, then find the value of cosec2x.
View Solution