1250 Persons went to see a circus-show. Each adult paid 75rs and each child paid 25rs for the admission ticket. Find the number of adults and number of children, if the total collection from them amounts to 61250rs.
The correct Answer is:x=600, y=650
To solve the problem of finding the number of adults and children who attended the circus show, we can set up a system of equations based on the information provided.
Step 1: Define Variables
Let:
- x = number of adults
- y = number of children
Step 2: Set Up the Equations
From the problem, we have two key pieces of information:
1. The total number of persons (adults + children) is 1250.
2. The total collection from ticket sales is 61250 Rs.
From this, we can create the following equations:
1. x+y=1250 (Equation 1)
2. 75x+25y=61250 (Equation 2)
Step 3: Simplify Equation 2
To make calculations easier, we can simplify Equation 2. We can divide the entire equation by 25:
3x+y=2450(Equation 3)
Step 4: Solve the System of Equations
Now we have two equations:
1. x+y=1250 (Equation 1)
2. 3x+y=2450 (Equation 3)
We can subtract Equation 1 from Equation 3:
(3x+y)−(x+y)=2450−1250
This simplifies to:
2x=1200
Dividing both sides by 2 gives:
x=600
Step 5: Find the Number of Children
Now that we have the number of adults, we can find the number of children using Equation 1:
y=1250−x=1250−600=650
Conclusion
Thus, the number of adults is 600 and the number of children is 650.
Final Answer:
- Number of Adults: 600
- Number of Children: 650
Let:
- x = number of adults
- y = number of children
From the problem, we have two key pieces of information:
1. The total number of persons (adults + children) is 1250.
2. The total collection from ticket sales is 61250 Rs.
1. x+y=1250 (Equation 1)
2. 75x+25y=61250 (Equation 2)
To make calculations easier, we can simplify Equation 2. We can divide the entire equation by 25:
3x+y=2450(Equation 3)
Now we have two equations:
1. x+y=1250 (Equation 1)
2. 3x+y=2450 (Equation 3)
(3x+y)−(x+y)=2450−1250
This simplifies to:
2x=1200
Dividing both sides by 2 gives:
x=600
Now that we have the number of adults, we can find the number of children using Equation 1:
y=1250−x=1250−600=650
Thus, the number of adults is 600 and the number of children is 650.
- Number of Adults: 600
- Number of Children: 650
Topper's Solved these Questions
- SIMULTANEOUS LINEAR EQUATIONS IN TWO VARIABLESBOOK - ICSECHAPTER - SIMULTANEOUS LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Topic 2 (4 Marks questions)
8 Videos
- SIMULTANEOUS LINEAR EQUATIONS IN TWO VARIABLESBOOK - ICSECHAPTER - SIMULTANEOUS LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Topic 1 (4 Marks questions)
8 Videos
- SIMULTANEOUS EQUATIONSBOOK - ICSECHAPTER - SIMULTANEOUS EQUATIONSEXERCISE - EXERCISE 6 (G)
13 Videos
- SOLIDSBOOK - ICSECHAPTER - SOLIDSEXERCISE - Exercise 21(C )
10 Videos
Similar Questions
A cottage industry produces a certain number of pottery articles in a day. It was observed on a particular day that the cost of production of each article (in rupees) was 3 more than twice the number of articles produced on that day. If the total cost of production on that day was Rs 90. Find the number of articles produced and the cost of each article.
View SolutionA cottage industry produces a certain number of pottery articles in a day. It was observed on a particular day that the cost of production of each article (in rupees) was 3 more than twice the number of articles produced on that day. If the total cost of production on that day was Rs 90. Find the number of articles produced and the cost of each article.
View Solution
Knowledge Check
Admission to the martial arts tournament is $30, but participants must purchase separate tickets for each event they with to participate in once inside . Each event is the same price as any other event . The graph below shows the total cost for a person, for admission and events , as a function of the number of events paid for . One of the following is the price of a single event. Which one is it ?
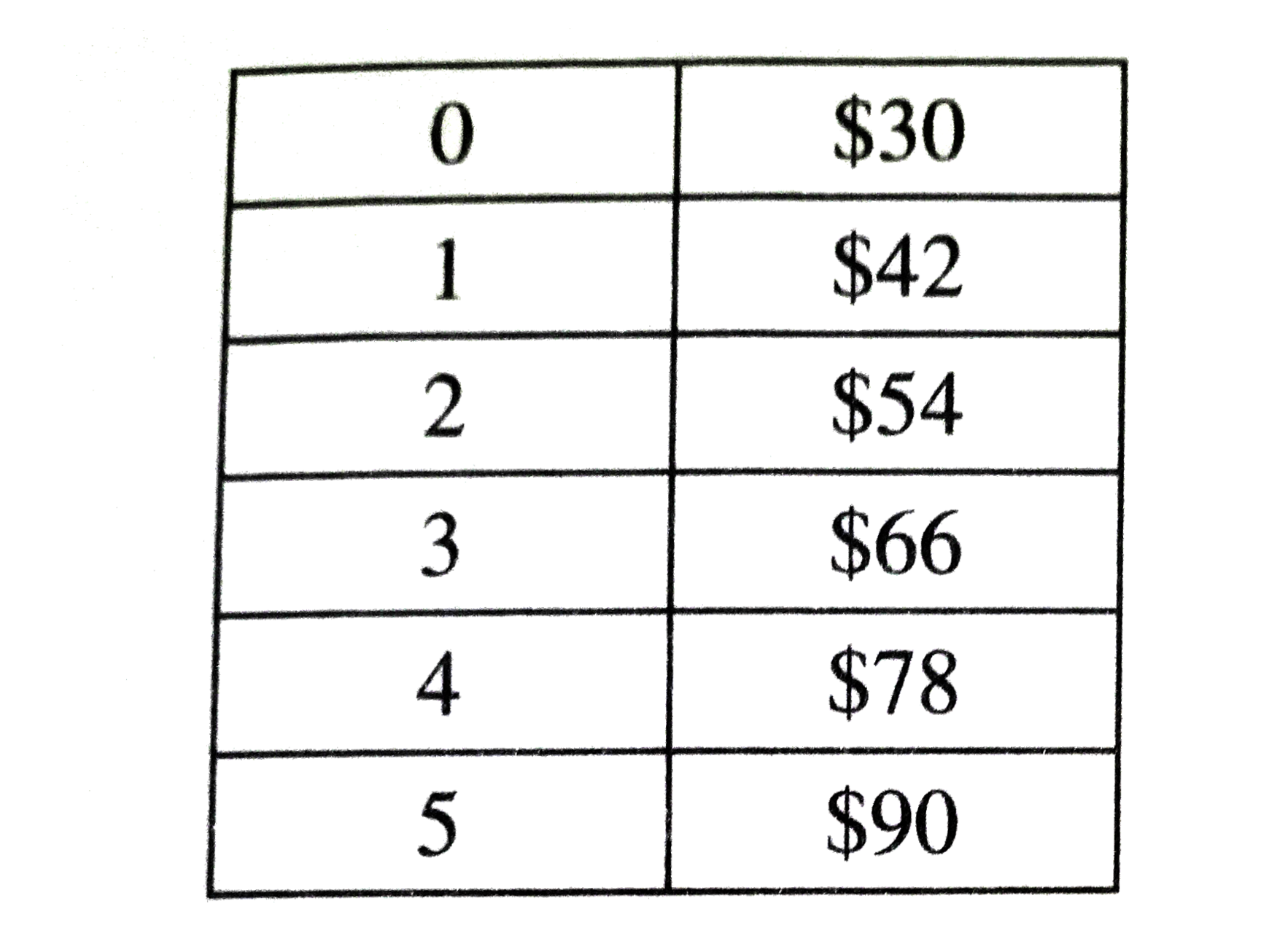 A$11B$12C$13D$14
A$11B$12C$13D$14
A sum of rs 800 is in the form of denomination of rs 10 and rs 20.If the total number of notes are 50, find the number of notes of each type.
View SolutionThere are some 50 paisa and some 25 paisa coins in a ba. If the total number of coins is 30 and their total value is Rs. 11, find the number of coins of each kind.
View SolutionA sum of Rs. 500 is in the form of denominations of Rs. 5 and Rs. 10. If the total number of notes is 90, find the number of notes of each type.
View SolutionA school collected Rs 2304 as fees from its students. If each student paid as many paise as there were students in the school, how many students were there in the school ?
View SolutionThe dimensions of a solid metallic cuboid are 72cm×30cm×75cm. It is melted and recast into identical solid metal cubes with each of edge 6cm. Find the number of cubes formed. Alos, find the cost of polishing the surface of all the cubes formed at the rate Rs 150 per sq. m.
View SolutionRs 7500 were divided equally among a certain number of children. Had there been 20 less children, each would have received Rs 100 more. Find the original number of children.
View SolutionRs 480 is dividend equally among 'x' children. If the number of children were 20 more than each would have got Rs 12 less. Find 'x'.
View Solution
