There are two series of index numbers: P for price index and S to stock of a commodity. The mean and standard deviation of P are 100 and 8 and of S are 103 and 4 respectively. The correlation coefficient between the two series is 0.4. With these data, obtain the regression lines of P on S and S on P.
To solve the problem of finding the regression lines of P on S and S on P, we will follow these steps:
Given Data:
- Mean of P (mX) = 100
- Standard deviation of P (σX) = 8
- Mean of S (mY) = 103
- Standard deviation of S (σY) = 4
- Correlation coefficient (R) = 0.4
Step 1: Find the regression line of P on S (X on Y)
The regression line of X on Y can be expressed as:
X−mX=BYX(Y−mY)
Where BYX is the regression coefficient of X on Y.
Step 2: Calculate BYX
The formula for BYX is:
BYX=R⋅σXσY
Substituting the values:
BYX=0.4⋅84=0.4⋅2=0.8
Step 3: Substitute into the regression line equation
Now substituting BYX and the means into the regression line equation:
X−100=0.8(Y−103)
Step 4: Rearranging the equation
Expanding and rearranging gives:
X−100=0.8Y−82.4
X−0.8Y=100−82.4
X−0.8Y=17.6
Step 5: Write the regression line of P on S
The regression line of P on S is:
X−0.8Y=17.6
Step 6: Find the regression line of S on P (Y on X)
The regression line of Y on X can be expressed as:
Y−mY=BXY(X−mX)
Where BXY is the regression coefficient of Y on X.
Step 7: Calculate BXY
The formula for BXY is:
BXY=R⋅σYσX
Substituting the values:
BXY=0.4⋅48=0.4⋅0.5=0.2
Step 8: Substitute into the regression line equation
Now substituting BXY and the means into the regression line equation:
Y−103=0.2(X−100)
Step 9: Rearranging the equation
Expanding and rearranging gives:
Y−103=0.2X−20
0.2X−Y+103−20=0
0.2X−Y+83=0
Step 10: Write the regression line of S on P
The regression line of S on P is:
0.2X−Y+83=0
Final Answers:
1. The regression line of P on S is:
X−0.8Y=17.6
2. The regression line of S on P is:
0.2X−Y+83=0
- Mean of P (mX) = 100
- Standard deviation of P (σX) = 8
- Mean of S (mY) = 103
- Standard deviation of S (σY) = 4
- Correlation coefficient (R) = 0.4
The regression line of X on Y can be expressed as:
X−mX=BYX(Y−mY)
Where BYX is the regression coefficient of X on Y.
The formula for BYX is:
BYX=R⋅σXσY
Substituting the values:
BYX=0.4⋅84=0.4⋅2=0.8
Now substituting BYX and the means into the regression line equation:
X−100=0.8(Y−103)
Expanding and rearranging gives:
X−100=0.8Y−82.4
X−0.8Y=100−82.4
X−0.8Y=17.6
The regression line of P on S is:
X−0.8Y=17.6
The regression line of Y on X can be expressed as:
Y−mY=BXY(X−mX)
Where BXY is the regression coefficient of Y on X.
The formula for BXY is:
BXY=R⋅σYσX
Substituting the values:
BXY=0.4⋅48=0.4⋅0.5=0.2
Now substituting BXY and the means into the regression line equation:
Y−103=0.2(X−100)
Expanding and rearranging gives:
Y−103=0.2X−20
0.2X−Y+103−20=0
0.2X−Y+83=0
The regression line of S on P is:
0.2X−Y+83=0
1. The regression line of P on S is:
X−0.8Y=17.6
2. The regression line of S on P is:
0.2X−Y+83=0
Topper's Solved these Questions
Similar Questions
Knowledge Check
If coefficient of correlation between two variables X and Y is 0.64,cov(X,Y)=16 and Var(X)=9, then the standard deviation of Y series is
A1.2B8.33C24D25The equivalent resistance of series combination of four equal resistors is S. If they are joined in parallel, the total resistance is P. The relation between S and P is given by S = nP. Then the minimum possible value of n is
A12B14C16D10
The number of radial nodes in 4s and 3p orbitals are respectively
View SolutionThe distance of two points P and Q on the parabola y2=4ax from the focus S are 3 and 12 respectively. The distance of the point of intersection of the tangents at P and Q from the focus S is
View SolutionAt two point P and Q on screen in Young's double slit experiment, waves from slits S1 and S2 have a path difference of 0 and λ4 respectively. The ratio of intensities at P and Q will be:
View SolutionThe coefficient of correlation between the values denoted by X and Y is 0.5. The mean of X is 3 and that of Y is 5. Their standard deviations are 5 and 4 respectively. Find
(i) the two lines of regression.
(ii) the expected value of Y, when X is given 14
(iii) the expected value of when Y is given 9
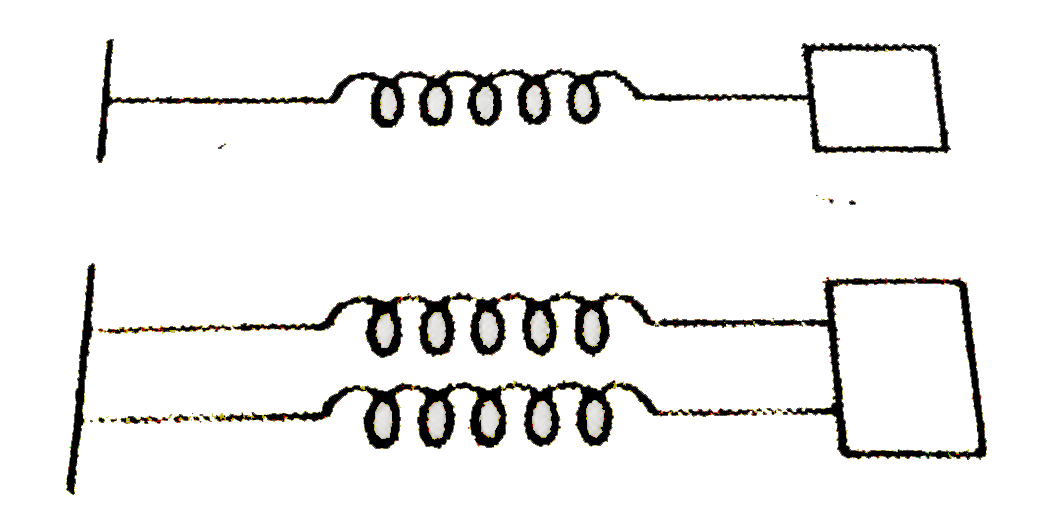
View SolutionIf ks and kp respectively are effective spring constant in series and parallel combination of springs as shown in figure, find kskp.
View SolutionThe resistance of a series combination of the resistance is S. when they are joined in parallel equvalent resistance is P. Find the value of (S2P)
View Solution