An ideal inductor does not consume any power even though both V and I are non zero. Explain n brief.
To explain why an ideal inductor does not consume any power even though both voltage (V) and current (I) are non-zero, we can follow these steps:
Step 1: Understand the Power Formula
The power consumed in an electrical circuit can be calculated using the formula:
P=Vrms⋅Irms⋅cosϕ
where:
- P is the power,
- Vrms is the root mean square (RMS) voltage,
- Irms is the root mean square (RMS) current,
- ϕ is the phase difference between the voltage and current.
Step 2: Analyze the Phase Difference
In the case of an ideal inductor, the current lags the voltage by a phase difference of 90∘. This means that:
ϕ=90∘
Step 3: Calculate the Power Factor
The cosine of the phase angle ϕ is given by:
cos(90∘)=0
Step 4: Substitute into the Power Formula
Substituting the value of cosϕ into the power formula, we get:
P=Vrms⋅Irms⋅cos(90∘)
P=Vrms⋅Irms⋅0
P=0
Step 5: Conclusion
Thus, even though both the voltage and current in an ideal inductor are non-zero, the power consumed is zero because the power factor is zero due to the 90∘ phase difference.
The power consumed in an electrical circuit can be calculated using the formula:
P=Vrms⋅Irms⋅cosϕ
where:
- P is the power,
- Vrms is the root mean square (RMS) voltage,
- Irms is the root mean square (RMS) current,
- ϕ is the phase difference between the voltage and current.
In the case of an ideal inductor, the current lags the voltage by a phase difference of 90∘. This means that:
ϕ=90∘
The cosine of the phase angle ϕ is given by:
cos(90∘)=0
Substituting the value of cosϕ into the power formula, we get:
P=Vrms⋅Irms⋅cos(90∘)
P=Vrms⋅Irms⋅0
P=0
Thus, even though both the voltage and current in an ideal inductor are non-zero, the power consumed is zero because the power factor is zero due to the 90∘ phase difference.
Similar Questions
Answer the following:
(i) When an AC source is connected to an ideal inductor show that the average power supplied by the source every complete cycle is zero.
(ii) A lamp is connected in series with an inductor and an AC source. What happens to the brightness of the lamp when the key is plugged in and an iron rod is inserted in the inducutor ? Explain.

View SolutionA time varying voltage V= 2t (Volt) is applied across and ideal inductor of inductance L =2H as shown in Fig. Then (assume current to be zero at t =0)
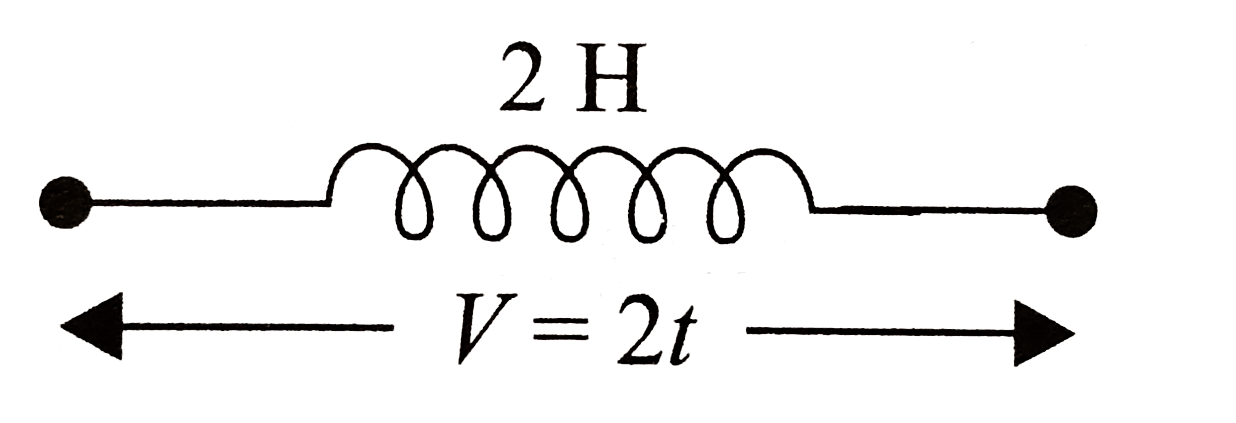
View SolutionEven though an electric field →E exerts a force q→E on a charged particle yet the electric field of an EM wave does not contribute to the radiation pressure (but transfer energy). Explain.
View SolutionNepenthes (commonly called as pitcher plant), an insectivorous plant usually grows in marsh lands. Which part of the plant is modified into a 'pitcher'? How does this modification help the plant for food even though it can photosynthesize like any other green plant?
View SolutionAssertion (A): The nucleus of gold is stable even though there is a very strong coulombic repulsion among the protons.
Reason (R): The inverse square coulomb force is exactly balanced by another inverse square force which is very powerful. i.e., nuclear force.
View SolutionFor an ideal inductor, connected across a sinusoidal ac voltage source, state which one of the following quantity is zero:
(i) Instantaneous power is zero :
(ii) Average power over full cycle of the ac voltage source.
View SolutionA type of single pulley is very often used as a machine even though it does not give any gain in mechanical advantage.
For what purpose is such a pulley used ?
View SolutionA type of single pulley is very often used as a machine even though it does not give any gain in mechanical advantage.
Name the type of pulley used.
View SolutionIn an a.c. circuit, there is no power consumption in an ideal inductor. Explain.
View SolutionCan an inductor have potential difference across it ends even if does not store any energy.
View Solution
