Question

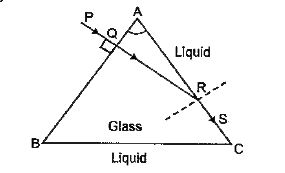
A narrow beam of monochromatic light, PQ, is incident normally on one face of an equiangular glass prism of refractive index $1.45$. When the prism is immersed in a certain liquid, the ray makes a grazing emergence along the other face (figure). Find the refractive index of this liquid .

Explanation

To solve the problem of finding the refractive index of the liquid in which the equiangular glass prism is immersed, we can follow these steps:

Step 1: Understand the Geometry of the Prism
The prism is equiangular, which means all angles are equal. For an equiangular prism, the angle \( A \) is \( 60^\circ \).

Step 2: Identify the Given Values
- Refractive index of glass, \( \mu_g = 1.45 \)
- Angle of the prism, \( A = 60^\circ \)

Step 3: Determine the Emergence Condition
When the light ray emerges from the prism grazing along the other face, it means that the angle of refraction at the second face is \( 90^\circ \). This implies that the angle of incidence at the second face is equal to the critical angle for the glass-liquid interface.

Step 4: Use Snell's Law
According to Snell's Law:
\( \mu_g \sin(i) = \mu_l \sin(r) \)
Where:
- \( i \) is the angle of incidence at the second face (which is \( 60^\circ \) for an equiangular prism).
- \( r \) is the angle of refraction (which is \( 90^\circ \) since the ray grazes the surface).

Since \( \sin(90^\circ) = 1 \), we can simplify the equation:
\( \mu_g \sin(60^\circ) = \mu_l \cdot 1 \)

Step 5: Calculate \( \sin(60^\circ) \)
We know:
\( \sin(60^\circ) = \frac{\sqrt{3}}{2} \)

Step 6: Substitute Values into the Equation
Substituting the known values into the equation:
\( 1.45 \cdot \frac{\sqrt{3}}{2} = \mu_l \)

Step 7: Calculate the Refractive Index of the Liquid
Now, calculate \( \mu_l \):
\( \mu_l = 1.45 \cdot \frac{\sqrt{3}}{2} \approx 1.45 \cdot 0.866 \approx 1.256 \)

Conclusion
The refractive index of the liquid is approximately \( 1.256 \).

---