The molar conductivity at infinite dilution of a strong electrolyte can be obtained by extrapolating the curve versus but same cannot be done for a weak electrolyte. Explain.
To explain why the molar conductivity at infinite dilution of a strong electrolyte can be obtained by extrapolating the curve of molar conductivity (λ_m) versus the square root of concentration (√c), while the same cannot be done for a weak electrolyte, we can follow these steps:
1. Understanding Molar Conductivity (λ_m):
- Molar conductivity (λ_m) is a measure of how well an electrolyte conducts electricity when dissolved in a solution. It is defined as the conductivity (κ) of the solution divided by the concentration (c) of the electrolyte.
2. Strong Electrolytes and Their Behavior:
- Strong electrolytes, such as NaCl or KCl, completely dissociate into their ions in solution. This means that their molar conductivity increases linearly with the concentration of the electrolyte.
- The relationship can be described by the Debye-Hückel-Onsager equation, which states:
λm=λ0m−b√c
where λ0m is the molar conductivity at infinite dilution, b is an empirical constant, and c is the concentration of the electrolyte.
3. Graphing λ_m vs. √c:
- When plotting λ_m against √c for strong electrolytes, the graph is a straight line with a negative slope. The y-intercept of this line corresponds to λ0m, allowing us to easily extrapolate to find the molar conductivity at infinite dilution.
4. Weak Electrolytes and Their Behavior:
- Weak electrolytes, such as acetic acid (CH₃COOH), do not fully dissociate in solution. Instead, they establish an equilibrium between the undissociated and dissociated forms.
- The degree of dissociation is low, and as a result, the relationship between λ_m and √c is not linear. Weak electrolytes do not obey the same equation as strong electrolytes.
5. Graphing λ_m vs. √c for Weak Electrolytes:
- When plotting λ_m against √c for weak electrolytes, the graph does not yield a straight line. Instead, it tends to curve and approaches a limit without ever reaching the y-axis at a finite point.
- This means that we cannot extrapolate to find λ0m because the graph does not intersect the y-axis at a defined point.
6. Conclusion:
- Therefore, while we can determine the molar conductivity at infinite dilution for strong electrolytes through extrapolation of a linear graph, we cannot do the same for weak electrolytes due to their non-linear behavior and incomplete dissociation in solution.
- Molar conductivity (λ_m) is a measure of how well an electrolyte conducts electricity when dissolved in a solution. It is defined as the conductivity (κ) of the solution divided by the concentration (c) of the electrolyte.
- Strong electrolytes, such as NaCl or KCl, completely dissociate into their ions in solution. This means that their molar conductivity increases linearly with the concentration of the electrolyte.
- The relationship can be described by the Debye-Hückel-Onsager equation, which states:
λm=λ0m−b√c
where λ0m is the molar conductivity at infinite dilution, b is an empirical constant, and c is the concentration of the electrolyte.
- When plotting λ_m against √c for strong electrolytes, the graph is a straight line with a negative slope. The y-intercept of this line corresponds to λ0m, allowing us to easily extrapolate to find the molar conductivity at infinite dilution.
- Weak electrolytes, such as acetic acid (CH₃COOH), do not fully dissociate in solution. Instead, they establish an equilibrium between the undissociated and dissociated forms.
- The degree of dissociation is low, and as a result, the relationship between λ_m and √c is not linear. Weak electrolytes do not obey the same equation as strong electrolytes.
- When plotting λ_m against √c for weak electrolytes, the graph does not yield a straight line. Instead, it tends to curve and approaches a limit without ever reaching the y-axis at a finite point.
- This means that we cannot extrapolate to find λ0m because the graph does not intersect the y-axis at a defined point.
- Therefore, while we can determine the molar conductivity at infinite dilution for strong electrolytes through extrapolation of a linear graph, we cannot do the same for weak electrolytes due to their non-linear behavior and incomplete dissociation in solution.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE (PART-II Descriptive Questions)(LONG ANSWER QUESTIONS)33videos
ELECTROCHEMISTRY
- BOOK - ICSECHAPTER - ELECTROCHEMISTRYEXERCISE - ISC EXAMINATION QUESTIONS (PART-I Objective Questions) (Fill in the blanks)8videos
ELECTROCHEMISTRY
- BOOK - ICSECHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE (PART-II Descriptive Questions)74videos
ELECTROCHEMISTRY
- BOOK - ICSECHAPTER - DISTINCTION BETWEEN PAIRS OF COMPOUNDS EXERCISE - QUESTIONS155videos
DISTINCTION BETWEEN PAIRS OF COMPOUNDS
- BOOK - ICSECHAPTER - GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTS EXERCISE - EXERCISE (PART-I ( OBJECTIVE QUESTIONS )) (Fill in the blanks)60videos
GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTS
Similar Questions
Knowledge Check
- Question 1 - Select One
The variation in Λm with concentration for a strong electrolyte can be represented by the equation, Λm=Λ∘m−AC1/2 The value of constant A for a given solvent and temperature depends upon the type of electrolyte i.e., cations and anions produced on dissociation of electrolyte in the solution .
NaCl, MgCl2 and CaSO4 are known asA1-1,2-1, and 2-2 type electrolytes respectivelyBstrong, weak and strong electrolytes respectivelyCelectrolytes with different value of ADelectrolytes with same molar conductivity . - Question 2 - Select One
The variation in Λm with concentration for a strong electrolyte can be represented by the equation, Λm=Λ∘m−AC1/2 The value of constant A for a given solvent and temperature depends upon the type of electrolyte i.e., cations and anions produced on dissociation of electrolyte in the solution .
Which of the following statements is correct regarding variations of molar conductivity with concentration.AMolar conductivity decrease with decrease in concentrationBVariation in molar conductivity of weak and strong electrolytes is same.CMolar conductivity increases with decrease in concentration.DWhen concentration of the solution approaches zero, the molar conductivity is known as conductance.
Statement-I: Molar conductivity of a weak electrolyte at infinite dilution cannot be determined experimenttally.
Because Statement-II: Kohlrausch law help to find the moar conductivity of a weak electrolyte at infinite dilution.View SolutionA: Molar conductivity of solution increase with dilution for weak electrolyte.
R: Degree of ionization increases with dilution.View SolutionExplain with a graph the variation of molar conductivity of a strong electrolyte with dilution.
View SolutionSTATEMENT 1 The molar conductance of weak electrolyte at infinite dilutin is equal to sum of molar conductanced of cation and anion and
STATEMENT 2 Kohlraushc 's law is applicable for both strong and weak electrolytesView SolutionThe following curve is obtained when molar conductivity (∧m) is plotted against the square root of concentration, c1/2 for two electrolytes A and B
(a) How do you account for the increase in the molar conductivity of the electrolyte A on dilution.
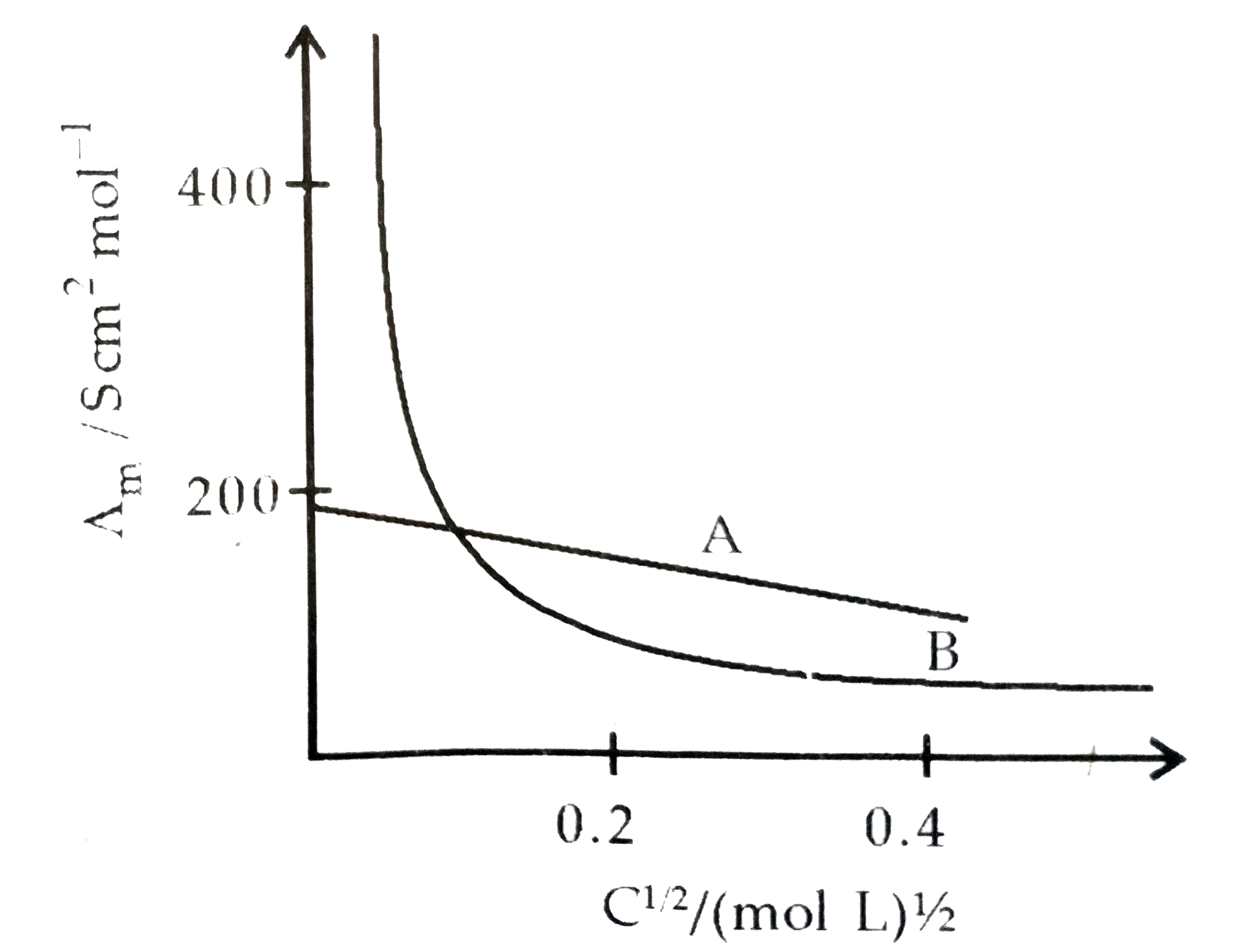
(b) As seen from the graph, the value of limiting molar conductivity (∧0m) for electrolyte B cannot be obtained graphically. How can this value be obtained?1View SolutionThe molar conductance at infinite dilution for electrolytes BA and CA are 140 and 120 ohm−1Cm2mol−1 respectively. If the molar conductance at infinite dilution of BX is 198 ohm−1cm2mol−1, then then at infinite dilution, the molar conductance of is:
View Solution

