Make any acute angle in your notebook. Using a compass, construct its angle bisector.
Step-by-Step Solution
1. Draw a Line Segment: Begin by drawing a straight line segment on your notebook. Label the endpoints of this line segment as points A and B.
Hint: Use a ruler to ensure that the line segment is straight and neat.
2. Create an Acute Angle: From point A, draw a ray that forms an acute angle with line segment AB. Label the point where this ray intersects the space as point C. Thus, you have formed angle CAB.
Hint: An acute angle is less than 90 degrees, so make sure the angle you create is sharp and not too wide.
3. Identify the Vertex: Identify the vertex of the angle, which is point A in this case.
Hint: The vertex is the point where the two rays of the angle meet.
4. Set the Compass: Place the compass point on vertex A and draw an arc that intersects both rays of the angle (AC and AB). Label the points where the arc intersects the rays as points D and E.
Hint: Ensure that the compass width is not too large; it should be able to intersect both rays comfortably.
5. Draw Arcs from Points D and E: Without changing the compass width, place the compass point on point D and draw an arc above the angle. Then, place the compass point on point E and draw another arc that intersects the first arc. Label the intersection point of these two arcs as point G.
Hint: Make sure to keep the compass width the same for both arcs to ensure they intersect correctly.
6. Draw the Angle Bisector: Finally, use a ruler to draw a straight line from point A through point G. This line AG is the angle bisector of angle CAB.
Hint: The angle bisector divides the angle into two equal parts, so check that both angles AGB and AGC appear to be equal.
Summary of Steps
- Draw line segment AB.
- Create an acute angle CAB.
- Draw an arc from vertex A intersecting rays AC and AB at points D and E.
- Draw arcs from points D and E to find intersection point G.
- Connect point A to point G to complete the angle bisector.
- Draw line segment AB.
- Create an acute angle CAB.
- Draw an arc from vertex A intersecting rays AC and AB at points D and E.
- Draw arcs from points D and E to find intersection point G.
- Connect point A to point G to complete the angle bisector.
Similar Questions
By using ruler and compass, construct angles of :
150∘
View SolutionBy using ruler and compass, construct angles of :
105∘.
View SolutionConstruct ∠BACand∠BAD as shown below in your notebook ! using protractor. Then, using a compass construct:
∠SMT=∠BAC+∠BAD
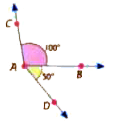
View SolutionConstruct ∠BACand∠BAD as shown below in your notebook ! using protractor. Then, using a compass construct:
∠PQR=∠BAC−∠BAD

View SolutionConstruct an angle of 90∘ at the initial point of a given ray and justify the construction. Draw its angle bisector.
View SolutionWith the help of compass, construct an angle measuring 52.5∘
View SolutionWith the help of compass , construct an angle measuring 22.5∘
View SolutionDraw an angle ABC of measure 135∘ using a compass.
View SolutionUsing a protractor , construct ∠ABC=85∘and∠PQR=50∘ . Using a compass construct ∠ABC+∠PQR.
View SolutionUsing ruler and compasses only, draw a right angle.
View Solution
