Name the following from the polygon ABCD shown alongside.
a. All the sides
b. All the vertices
c. Four pairs of adjacent sides
d. All the pairs of opposite sides
e. all of its diagonals.
 .
.
The correct Answer is:a. AB,BC,CD,DA
b. A,B,C,D
c. (AB,BC),(BC,CD),(CD,DA),(DA,AB).
d. (AB,CD),(BC,DA)
e. AC and BD.
Step by step video, text & image solution for Name the following from the polygon ABCD shown alongside. a. All the sides b. All the vertices c. Four pairs of adjacent sides d. All the pairs of opposite sides e. all of its diagonals. . by Maths experts to help you in doubts & scoring excellent marks in Class 6 exams.
Updated on:21/07/2023Similar Questions
In Figure, write all pairs of adjacent angles and all the linear pairs.
View SolutionAnswer the following from the figure given alongside.
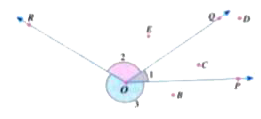 .
.
a. Name ∠POQ in two more ways.
b. Name all the pairs of adjacent angles.
c. Name the points in the interior of ∠1.
d. Name the points in the exterior of ∠1.
e. Name the points on ∠1.
View SolutionIf →a and →b represent two adjacent sides of a parallel then write vectors representing its diagonals.
View SolutionIn Figure, ABCD is a quadrilateral. Name a pair of adjacent sides. Name a pair of opposite sides. How many pairs of adjacent sides are there? How many pairs of opposite sides are there? Name a pair of adjacent angles. Name a pair of opposite angles. How many pairs of adjacent angles are there? How many pairs of opposite angles are there?
View SolutionDraw a rough sketch of a quadrilateral ABCD and name of the following :
Four pairs of adjacent sides
View SolutionProve that in a quadrilateral the sum of all the sides is greater than the sum of its diagonals.
View SolutionProve that in a quadrilateral the sum of all the sides is greater than the sum of its diagonals.
View SolutionIf the two sides of a pair of opposite sides of a cyclic quadrilateral are equal, prove that its diagonals are equal.
View SolutionIf the two sides of a pair of opposite sides of a cyclic quadrilateral are equal, prove that its diagonals are equal.
View SolutionIf the two sides of a pair of opposite sides of a cyclic quadrilateral are equal, prove that its diagonals are equal.
View Solution
