Find the value of ∫+l2−l2Mlx2dx where M and l are constants .
To solve the integral ∫+l2−l2Mlx2dx, where M and l are constants, we can follow these steps:
Step 1: Factor out the constant
The integral can be simplified by factoring out the constant Ml:
∫+l2−l2Mlx2dx=Ml∫+l2−l2x2dx
Step 2: Evaluate the integral
Next, we need to evaluate the integral ∫+l2−l2x2dx. The formula for the integral of xn is:
∫xndx=xn+1n+1+C
For our case, n=2:
∫x2dx=x33+C
Step 3: Apply the limits
Now we apply the limits from −l2 to +l2:
∫+l2−l2x2dx=(x33)+l2−l2=(l2)33−(−l2)33
Calculating this gives:
=l383−⎛⎜⎝−l383⎞⎟⎠=l324+l324=2l324=l312
Step 4: Substitute back into the equation
Now substitute this result back into the equation:
Ml∫+l2−l2x2dx=Ml⋅l312
Step 5: Simplify the expression
Simplifying this gives:
=Ml212
Final Result
Thus, the value of the integral ∫+l2−l2Mlx2dx is:
Ml212
The integral can be simplified by factoring out the constant Ml:
∫+l2−l2Mlx2dx=Ml∫+l2−l2x2dx
Next, we need to evaluate the integral ∫+l2−l2x2dx. The formula for the integral of xn is:
∫xndx=xn+1n+1+C
For our case, n=2:
∫x2dx=x33+C
Now we apply the limits from −l2 to +l2:
∫+l2−l2x2dx=(x33)+l2−l2=(l2)33−(−l2)33
Calculating this gives:
=l383−⎛⎜⎝−l383⎞⎟⎠=l324+l324=2l324=l312
Now substitute this result back into the equation:
Ml∫+l2−l2x2dx=Ml⋅l312
Simplifying this gives:
=Ml212
Thus, the value of the integral ∫+l2−l2Mlx2dx is:
Ml212
Topper's Solved these Questions
- BOOK - ICSECHAPTER - VECTORS SCALARS ELEMENTARY CALCULUS EXERCISE - CONCEPTUAL SHORT ANSWER QUESTIONS WITH ANSWERS12videos
VECTORS SCALARS ELEMENTARY CALCULUS
- BOOK - ICSECHAPTER - VECTORS SCALARS ELEMENTARY CALCULUS EXERCISE - LONG ANSWER QUESTIONS5videos
VECTORS SCALARS ELEMENTARY CALCULUS
- BOOK - ICSECHAPTER - VECTORS SCALARS ELEMENTARY CALCULUS EXERCISE - EXAMPLES26videos
VECTORS SCALARS ELEMENTARY CALCULUS
- BOOK - ICSECHAPTER - UNITSEXERCISE - MODULE 3 (SELECTED PROBLEMS)38videos
UNITS
- BOOK - ICSECHAPTER - WAVESEXERCISE - From Musical Sound10videos
WAVES
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the x value for which l is parallel to m.
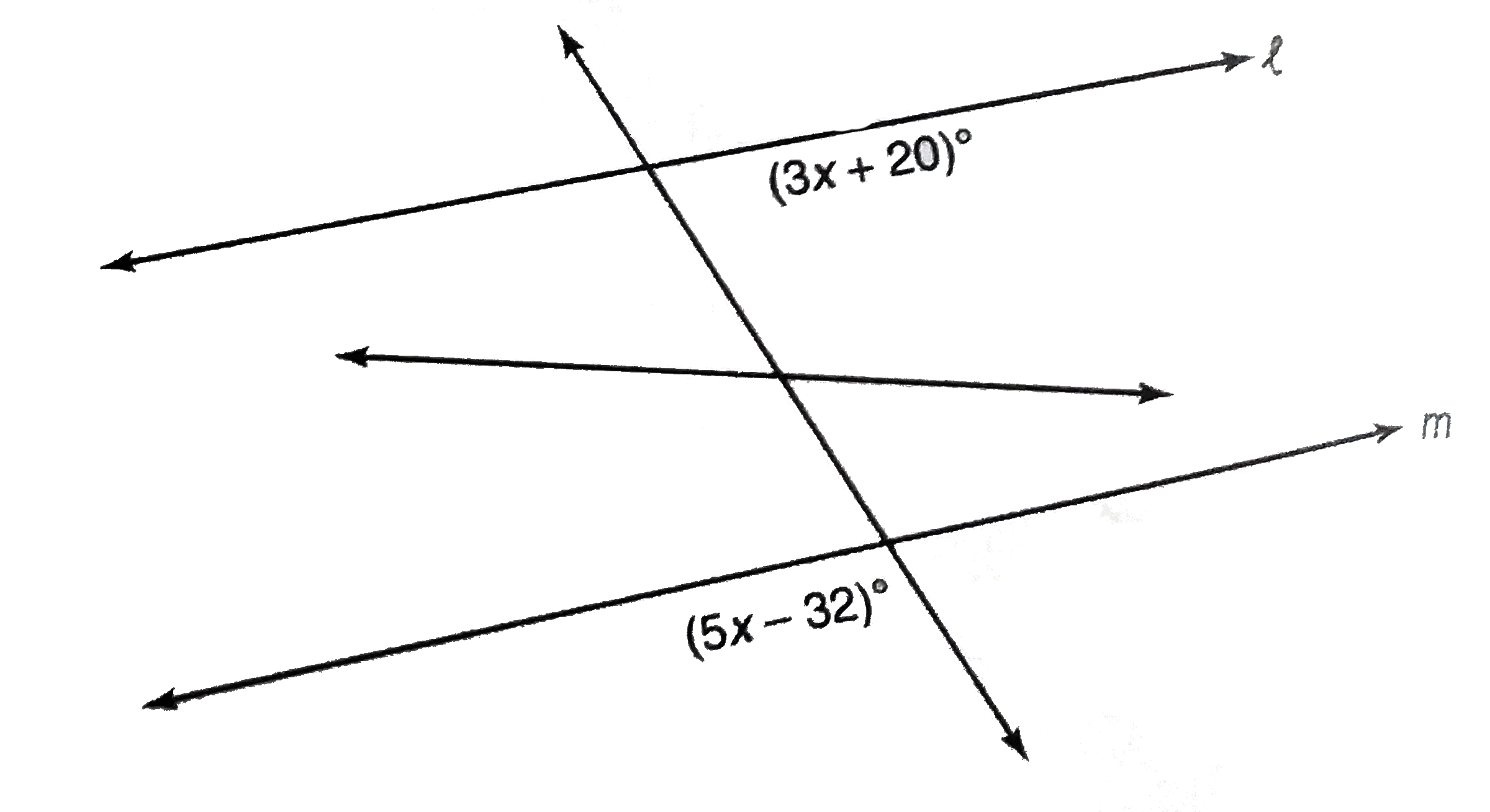 A6B16C20D24
A6B16C20D24
Let L=limn→∞∫∞andx1+n2x2, where a∈R, then L can be
View SolutionLet l=∫π/20cosxacosx+bsinxdx and J=∫π/20sinxacosx+bsinxdx, where a >0andb>0 Compute the values of l and j
View SolutionEvaluate ln=∫dx(x2+a2)n.
View SolutionLet l1=∫1041{√x}√xdx and l2=∫100x{x2}dx where {x} denotes fractional part of x then
View SolutionIf the area enclosed by the curve y2=4x and 16y2=5(x−1)3 can be expressed in the form L√MN where L and N are relatively prime and M is a prime, find the value of (L+M+N).
View SolutionLet the lines l1 and l2 be normals to y2=4x and tangents to x2=−12y (where l1andl2 are not x - axis). The absolute value of the difference of slopes of l1andl2 is
View SolutionEvaluate the integral l=∫20|1−x|dx.
View Solution

