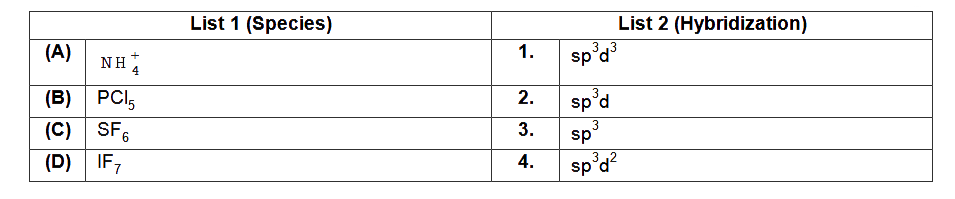Match List I with List II and select the correct answer
List IList II(System)(Expression for moment of inertia)A. A ring about its axis(1)MR2/2B. A uniform circular disc about its axis(2)2/5MR2C. A solid sphere about any diameter(3)7/5MR2D. A solid sphere about any tangent(4)MR2
Selec the answer from the codes given below
ABCD2134
ABCD4321
ABCD1432
ABCD4123
The correct Answer is:D
To solve the problem of matching the systems in List I with their corresponding expressions for the moment of inertia in List II, we will go through each item step by step.
1. Identify the Moment of Inertia for a Ring about its Axis:
- The moment of inertia I for a ring about its axis is given by the formula:
I=MR2
- Therefore, for option A (A ring about its axis), the correct expression is:
- A → (4) MR2
2. Identify the Moment of Inertia for a Uniform Circular Disc about its Axis:
- The moment of inertia I for a uniform circular disc about its axis is given by the formula:
I=12MR2
- Therefore, for option B (A uniform circular disc about its axis), the correct expression is:
- B → (1) 12MR2
3. Identify the Moment of Inertia for a Solid Sphere about any Diameter:
- The moment of inertia I for a solid sphere about any diameter is given by the formula:
I=25MR2
- Therefore, for option C (A solid sphere about any diameter), the correct expression is:
- C → (2) 25MR2
4. Identify the Moment of Inertia for a Solid Sphere about any Tangent:
- The moment of inertia I for a solid sphere about a tangent is given by the formula:
I=75MR2
- Therefore, for option D (A solid sphere about any tangent), the correct expression is:
- D → (3) 75MR2
Final Matching:
- A → (4) MR2
- B → (1) 12MR2
- C → (2) 25MR2
- D → (3) 75MR2
Conclusion:
The correct answer is:
- 4, 1, 2, 3
- The moment of inertia I for a ring about its axis is given by the formula:
I=MR2
- Therefore, for option A (A ring about its axis), the correct expression is:
- A → (4) MR2
- The moment of inertia I for a uniform circular disc about its axis is given by the formula:
I=12MR2
- Therefore, for option B (A uniform circular disc about its axis), the correct expression is:
- B → (1) 12MR2
- The moment of inertia I for a solid sphere about any diameter is given by the formula:
I=25MR2
- Therefore, for option C (A solid sphere about any diameter), the correct expression is:
- C → (2) 25MR2
- The moment of inertia I for a solid sphere about a tangent is given by the formula:
I=75MR2
- Therefore, for option D (A solid sphere about any tangent), the correct expression is:
- D → (3) 75MR2
- A → (4) MR2
- B → (1) 12MR2
- C → (2) 25MR2
- D → (3) 75MR2
The correct answer is:
- 4, 1, 2, 3
Topper's Solved these Questions
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - NDA EXAM QUESTIONS55videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)24videos
CIRCULAR MOTION
- BOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)9videos
DIMENSIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
Match List I with List II and select the correct answer using the codes given below the lists.
List IList II(Physical Quantity)(Dimension)A. Angular momentum(1)ML−1T−2B. Torque(2)MT−2C. Surface Tension(3)ML2T−1D. Coefficient of viscosity(4)ML2T−2AABCD4321BABCD3421CABCD1423DABCD3142
Match List 1 with List 2. Select the correct answer using the codes given below the list.
 View Solution
View SolutionMatch the list I with list II and select the correct answer using the code given below the lists.
List IList IIPSr2+1Golden yellowQK+2Apple greenRNa+3Crimson redSBa2+4LilacView SolutionMatch List I with List II and select the correct answer using the codes given below the lists :
List IList IIa.(SiH3)3Ni.3 centre -2-electron bondb.BF3ii.sp3−hybridizationc.SiO2iii.pπ−pπ bondd.B2H6iv.pπ−dπ bond
:View SolutionMatch list I with List II and select the correct answer using the codes given below
List IList II(types of ore )(example)POxide ore A.FeldsparQSulphide ore B.Barytes Rsulphate ore C.FluorsparSHalide ore D.GalenaE.CorundumView SolutionMatch list I with List II and select the correct option
List IList IIAProtozoa1 PennatulaBAschelminthes2 BeroeCPoorifera3 MonocystisDCtenophora4 WuchereriaECnidaria5 ClionaView SolutionMatch List I with List II and select the correct answer using the codes given below the lists :
List IList IIa.BBr3i.Dimerb.Tl2Oii.Trigonol planarc.B(OH)3iii.Basicd.AlCl3iv.Monobasic acid
View SolutionThe moment of inertia of a solid cylinder about its axis of rotation is MR22. What is the value of the radius of gyration of the cylinder about this axis ?
View Solution