Question

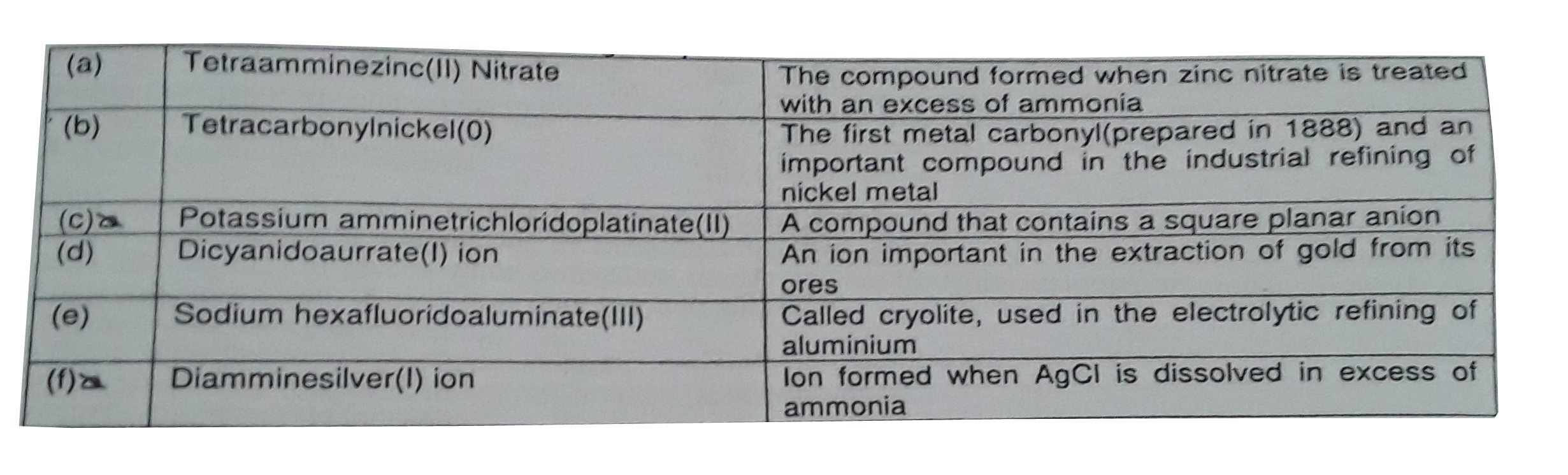
Write down the Newton's formula for the velocity of sound in a gas. Why did his formula give wrong results ?

Explanation

Step-by-Step Solution:

1. Newton's Formula for Velocity of Sound in Gas:
According to Newton, the velocity of sound in a gas is given by the formula:
\( v = \sqrt{\frac{p}{\rho}} \)
where:
- \( v \) is the velocity of sound,
- \( p \) is the pressure of the gas,
- \( \rho \) is the density of the gas.

2. Explanation of Why the Formula Gives Wrong Results:
Newton's formula is based on the assumption that sound travels isothermally. This means that he assumed the temperature of the gas remains constant during the propagation of sound waves. However, this assumption is incorrect for sound waves in gases.

3. Correct Nature of Sound Propagation:
In reality, sound travels as an adiabatic process. An adiabatic process is one in which there is no heat exchange with the surroundings. Sound waves travel very quickly, and there is not enough time for heat to be exchanged during the propagation of the wave. Therefore, the conditions under which sound travels are not isothermal but adiabatic.

4. Conclusion:
Since Newton's assumption about isothermal propagation of sound is incorrect, his formula does not yield accurate results for the velocity of sound in gases. The correct approach involves considering the adiabatic nature of sound propagation.