Which among the following relations is a function ?
x2+y2=r2
x2a2+y2b2=r2
y2=4ax
x2=dxy
The correct Answer is:D
To determine which among the given relations is a function, we need to analyze each relation based on the definition of a function. A relation is considered a function if for every value of x, there is exactly one corresponding value of y.
1. Relation 1: x2+y2=r2
- Rearranging gives y2=r2−x2.
- Taking the square root leads to y=±√r2−x2.
- For each value of x, there are two possible values of y (one positive and one negative).
- Conclusion: This relation is not a function.
2. Relation 2: x2a2+y2b2=r2
- Rearranging gives y2b2=r2−x2a2.
- This leads to y2=b2(r2−x2a2).
- Taking the square root gives y=±b√r2−x2a2.
- Again, for each value of x, there are two possible values of y.
- Conclusion: This relation is not a function.
3. Relation 3: y2=4ax
- Rearranging gives y=±√4ax.
- Similar to previous cases, for each positive value of x, there are two corresponding values of y (one positive and one negative).
- Conclusion: This relation is not a function.
4. Relation 4: x2=dxy
- Rearranging gives y=x2dx.
- Simplifying gives y=xd (assuming x≠0).
- For each value of x, there is exactly one corresponding value of y.
- Conclusion: This relation is a function.
Final Answer:
Among the given relations, the only relation that is a function is x2=dxy.
- Rearranging gives y2=r2−x2.
- Taking the square root leads to y=±√r2−x2.
- For each value of x, there are two possible values of y (one positive and one negative).
- Conclusion: This relation is not a function.
- Rearranging gives y2b2=r2−x2a2.
- This leads to y2=b2(r2−x2a2).
- Taking the square root gives y=±b√r2−x2a2.
- Again, for each value of x, there are two possible values of y.
- Conclusion: This relation is not a function.
- Rearranging gives y=±√4ax.
- Similar to previous cases, for each positive value of x, there are two corresponding values of y (one positive and one negative).
- Conclusion: This relation is not a function.
- Rearranging gives y=x2dx.
- Simplifying gives y=xd (assuming x≠0).
- For each value of x, there is exactly one corresponding value of y.
- Conclusion: This relation is a function.
Among the given relations, the only relation that is a function is x2=dxy.
Topper's Solved these Questions
- FUNCTION BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - FUNCTION EXERCISE - ONE OR MORE THAN ONE ANSWE IS/ARE CORRECT
23 Videos
- FUNCTION BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - FUNCTION EXERCISE - COMPREHENSION TYPE PROBLEMS
13 Videos
- ELLIPSE BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - ELLIPSE EXERCISE - Exercise-4 : Subjective Type Problems
2 Videos
- HYPERBOLABOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - HYPERBOLAEXERCISE - Exercise-4 : Subjective Type Problems
3 Videos
Similar Questions
Knowledge Check
Which of the following relations is a function?
AR= {(4,6), (3,9),(-11, 6), (3, 11)}BR = {(1,2), (2, 4), (2,6), (3,5)}CR = {(2,1),(4,3), (6,5), (8,7), (10,9)}DR= {(0,1),(1,3), (2,4), (3, 1), (3,5)}Which among the following is not a function of cytokinis?
AEssential for cytokinesis during cell divisionBDelays the senescence of leavesCHelps in fruit ripeningDHelps to overcome apical dominance
Which of the following relations are functions ? y=3x+2.
View SolutionWhich of the following relations are functions ?
y is a Maths pupil of x, where x represents any Maths teacher in a school.
View SolutionWhich of the following relation are functions? Give reasons. If it is a function, determine its domain and range.
{(a,b),(b,c),(c,d),(d,e)}
View SolutionWhich of the following relation are functions? Give reasons. In cane of a function, determine its domain and range.
{(1,0),(1,−1),(2,3),(4,10)}
View SolutionWhich of the following relation are functions? Give reasons. In cane of a function, determine its domain and range.
{(1,−2),(3,7),(4,−6),(8,11)}
View SolutionAcetic acid undergoes dimerisation, when dissolved in benzene
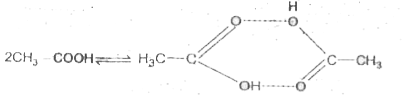
Molecular mass of acetic acid is found 120. which among the following relation is correct ?
View Solution
