Which option (s) is/are ture ?
f:R→R,f(x)=e|x|−e−x is many-one into function
f:R→R,f(x)=2x+|sinx| is one-one onto
f:R→R,f(x)=x2+4x+30x2−8x+18 is many-one onto
f:R→R,f(x)=2x2−x+57x2+2x+10 is many-one into
The correct Answer is:A, B, D
To solve the problem, we need to analyze the given function options one by one to determine which ones are true based on their properties (one-one, onto, etc.).
Step 1: Analyze Option A
- Function: f(x)=e|x|−e−x
- For x<0:
- |x|=−x
- Therefore, f(x)=e−x−ex
- This function is decreasing and takes the value 0 when x=−1 and x=−2.
- For x≥0:
- f(x)=2sinh(x) which is positive and increasing.
- Conclusion: The function is not onto since its range does not cover all of R (it only covers (0,∞)). Thus, Option A is true.
Step 2: Analyze Option B
- Function: f(x)=2x+|sinx|
- The derivative f′(x)=2+sinx|sinx|cosx is always positive.
- This indicates that f(x) is strictly increasing.
- The range of this function is (−∞,∞) and matches the codomain.
- Conclusion: The function is one-one and onto. Thus, Option B is true.
Step 3: Analyze Option C
- Function: f(x)=x2+4x+30x2−8x+18
- To check if it is one-one, we find the derivative using the quotient rule:
- f′(x)=(2x+4)(x2−8x+18)−(x2+4x+30)(2x−8)(x2−8x+18)2
- The numerator can be positive or negative, indicating that the function is not necessarily one-one.
- Additionally, for y=0, there is no x that satisfies this, indicating it is not onto.
- Conclusion: Option C is false.
Step 4: Analyze Option D
- Function: f(x)=2x2−x+57x2+2x+10
- Again, we find the derivative using the quotient rule:
- f′(x)=(4x−1)(7x2+2x+10)−(2x2−x+5)(14x+2)(7x2+2x+10)2
- The sign of the derivative can vary, indicating that it is not one-one.
- Also, for y=0, there is no x that satisfies this, indicating it is not onto.
- Conclusion: Option D is false.
Final Conclusion:
- The true options are A and B.
- Function: f(x)=e|x|−e−x
- For x<0:
- |x|=−x
- Therefore, f(x)=e−x−ex
- This function is decreasing and takes the value 0 when x=−1 and x=−2.
- For x≥0:
- f(x)=2sinh(x) which is positive and increasing.
- Conclusion: The function is not onto since its range does not cover all of R (it only covers (0,∞)). Thus, Option A is true.
- Function: f(x)=2x+|sinx|
- The derivative f′(x)=2+sinx|sinx|cosx is always positive.
- This indicates that f(x) is strictly increasing.
- The range of this function is (−∞,∞) and matches the codomain.
- Conclusion: The function is one-one and onto. Thus, Option B is true.
- Function: f(x)=x2+4x+30x2−8x+18
- To check if it is one-one, we find the derivative using the quotient rule:
- f′(x)=(2x+4)(x2−8x+18)−(x2+4x+30)(2x−8)(x2−8x+18)2
- The numerator can be positive or negative, indicating that the function is not necessarily one-one.
- Additionally, for y=0, there is no x that satisfies this, indicating it is not onto.
- Conclusion: Option C is false.
- Function: f(x)=2x2−x+57x2+2x+10
- Again, we find the derivative using the quotient rule:
- f′(x)=(4x−1)(7x2+2x+10)−(2x2−x+5)(14x+2)(7x2+2x+10)2
- The sign of the derivative can vary, indicating that it is not one-one.
- Also, for y=0, there is no x that satisfies this, indicating it is not onto.
- Conclusion: Option D is false.
- The true options are A and B.
Topper's Solved these Questions
- FUNCTION BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - FUNCTION EXERCISE - COMPREHENSION TYPE PROBLEMS
13 Videos
- FUNCTION BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - FUNCTION EXERCISE - MATCHING TYPE PROBLEMS
6 Videos
- FUNCTION BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - FUNCTION EXERCISE - SUBJECTIVE TYPE PROBLEMS
33 Videos
- ELLIPSE BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - ELLIPSE EXERCISE - Exercise-4 : Subjective Type Problems
2 Videos
- HYPERBOLABOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - HYPERBOLAEXERCISE - Exercise-4 : Subjective Type Problems
3 Videos
Similar Questions
Knowledge Check
Which of the following is ture for CO2 concentration ?
AMore in alveolar air than in expired airBMore in expired air than in alveolar airCMore in inspired air than in alveolar airDMore in inspired air than in expired air
Which of these options is categorized as a lectin ?
View SolutionIn which option products is not correct.
View SolutionWhich option is not matched in correct sequence
View SolutionWhich of the following option(s) is correct?
View SolutionWhich of the following is/are ture?
View SolutionGraph of y = ax^(2) + bx + c is as shown in the figure . If PQ=9,
OR=5 and OB=2.5, the which of the following is /are ture?
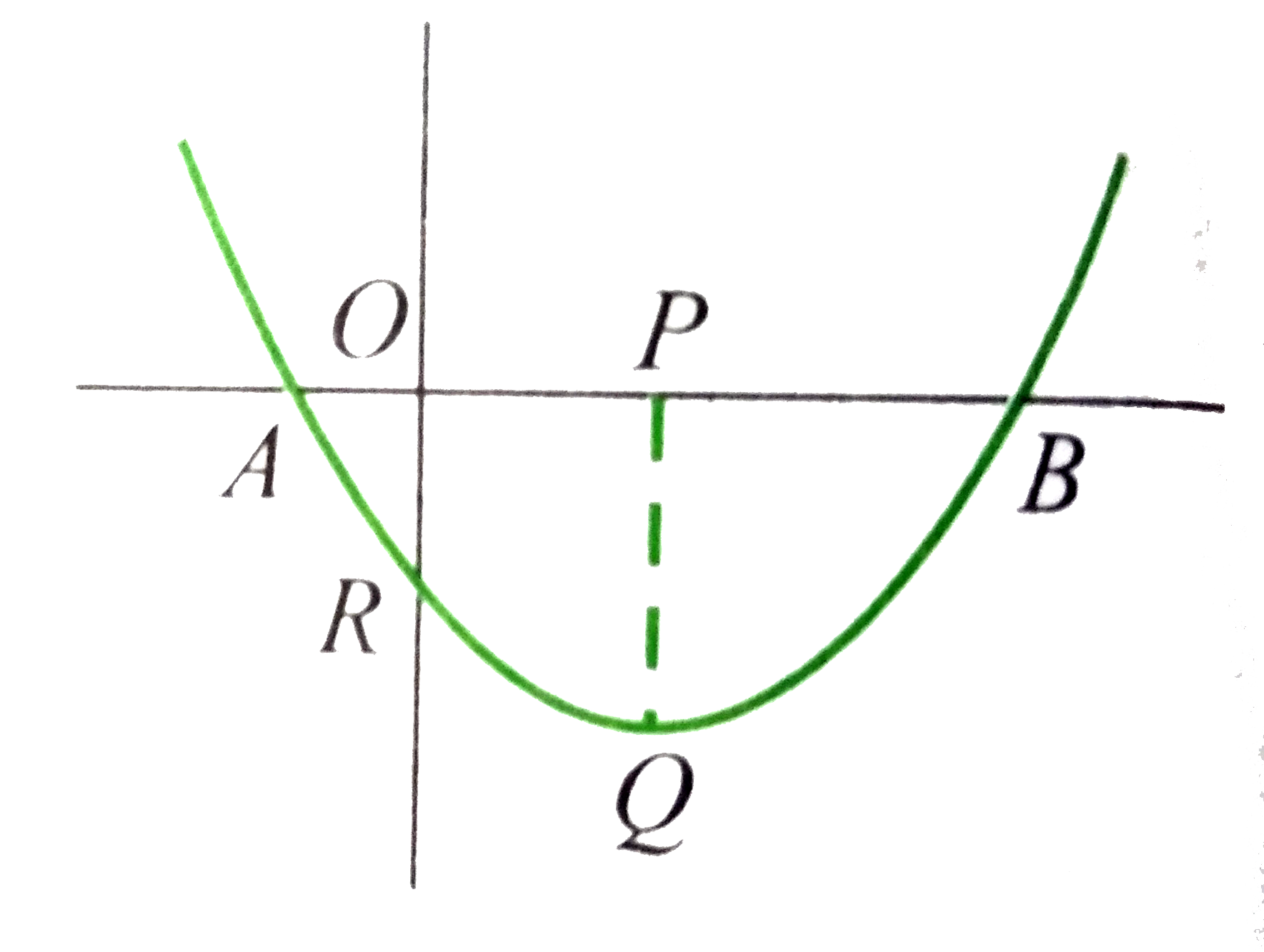
View SolutionWhich of the statement are ture?
View Solution
