The curve y=(λ=1)x2+2 intersects the curve y=λx+3 in exactly one point, if λ equals {−2,2} b. {1} c. {−2} d. {2}
The correct Answer is:4
Step by step video & image solution for The curve y=(lambda=1)x^2+2 intersects the curve y=lambdax+3 in exactly one point, if lambda equals {-2,2} b. {1} c. {-2} d. {2} by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - QUADRATIC EQUATIONS EXERCISE - EXERCISE (MATCHING TYPE PROBLEMS)4videos
QUADRATIC EQUATIONS
- BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - PROBABILITY EXERCISE - Exercise -5 : Subjective Type problems11videos
PROBABILITY
- BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)21videos
SEQUENCE AND SERIES
Similar Questions
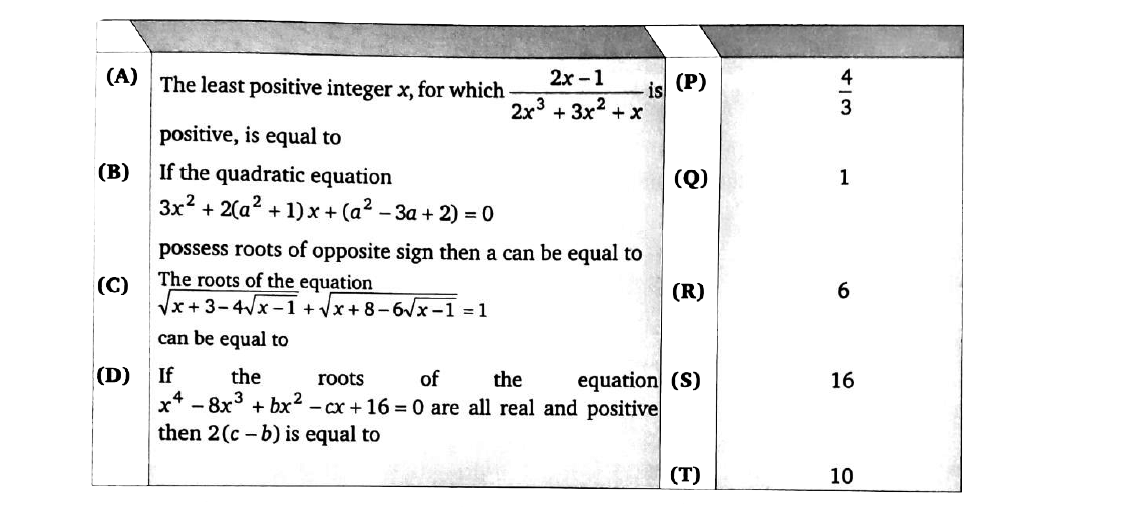
07:49View SolutionMr. A writes an article. The article originally is error free. Each day Mr. B introduces one new error into the article. At the end of the day, Mr. A checks the article and has 23 chance of catching each individual error still in the article. After 3 days, the probability that the article is error free can be expressed as pq where p and q are relatively prime positive integers. Let λ=q−p, then find the sum of the digits of λ.
01:36View SolutionLet a,b,c,d be four distinct real number in A.P.Then the smallest positive vlaue of k satisfying 2(a−b)+k(b−c)2+(c−a)3=2(a−d)+(b−d)2+(c−d)3is_________
03:22View SolutionThe lines joining the point of intersection of the line x+y=1 and the curve x^2+y^2-2y+lambda=0 to the origin are perpendicular, then value of lambda wil be: (A). 1/2 (B). -1/2 (C). 1/sqrt2 (D). 0
03:55View SolutionThe curve y=(λ+1)x2+2 intersects the curve y=λx+3 in exactly one point, if λ equals {−2,2} b. {1} c. {−2} d. {2}
06:05View SolutionTangents are drawn to the curve y=sin x from the origin.Then the point of contact lies on the curve (1)/(y^(2))-(1)/(x^(2))=lambda. The value of lambda is equal to
View SolutionThe area bounded by the curve y=x^(2) and y=(2)/(1+x^(2)) is lambda sq.units,then 2 the value of [lambda] is (A)2(B)3(C)4(D)5
04:27View SolutionIf the curves x2a2+y2b2=1andx2c2+y2d2=1 intersect orthogonally, then
04:08View SolutionConsider two curves y2=4a(x−λ) and x2=4a(y−λ), where a>0 and λ is a parameter. Show that
(i) there is a single positive value of λ for which the two curves have exactly one point of intersection in the 1st quadrant find it.
(ii) there are infinitely many nagetive values of λ for which the two curves have exactly one points of intersection in the 1st quadrant.
(iii) if λ=−a , then find the area of the bounded by the two curves and the axes in the 1st quadrant.04:43View SolutionIf the lines x−11=y−31=z−2λ and x−1λ=y−32=z−41 intersect at a point, then the value of λ2+4 is equal to
02:26View SolutionThe orthogonal trajectories of the curves x2a2+λ+y2b2+λ=1, λ being the parameter of the family is
01:38View SolutionThe differential equation of the system of curves given by x2a2+y2a2+λ=1
(λ is arbitrary constant) is05:23View SolutionThe curve y=(λ+1)x2+2 intersects the curve y=λx+3 in exactly one point, if λ equals {−2,2} b. {1} c. {−2} d. {2}
04:46View SolutionThe curve y=(λ+1)x2+2 intersects the curve y=λx+3 in exactly one point, if λ equals {−2,2} b. {1} c. {−2} d. {2}
10:14View SolutionProve that the family of curves x2a2+λ+y2b2+λ=1, where λ is a parameter, is self orthogonal.
06:58View Solutionमूल बिंदु से वक्र y = sin x पर स्पर्श रेखाऐं खींची जाती है, तो स्पर्श बिंदु वक्र 1y2−1x2=λ पर स्थित है, तब λ का मान है
03:18View Solution