A is the orthocentre of ΔABC and D is reflection point of A w.r.t. perpendicualr bisector of BC, then orthocenter of ΔDBC is :
D
C
B
A
The correct Answer is:A
Step by step video solution for A is the orthocentre of DeltaABC and D is reflection point of A w.r.t. perpendicualr bisector of BC, then orthocenter of DeltaDBC is : by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SOLUTION OF TRIANGLESBOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-2 : One or More than One Answer is/are Correct
15 Videos
- SOLUTION OF TRIANGLESBOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-3 : Comprehension Type Problems
13 Videos
- SEQUENCE AND SERIES BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
21 Videos
- STRAIGHT LINES BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - STRAIGHT LINES EXERCISE - Exercise-5 : Subjective Type Problems
10 Videos
Similar Questions
Differentiation of x2 w.r.t. x is
View SolutionIf the coordinates of points A,B,C satisfy the relation xy=1000 and (α,β) be the coordinates of the orthocentre of ΔABC, then the value of αβ is
View SolutionLet the equations of perpendicular bisectors of sides ACandofΔABCbex+y=3andx−y=1 respectively Then vertex A is is (0,0)
The orthocentre of ΔABC is
View SolutionThe equations of the sides AB and CA of a ΔABC are x+2y=0 and x−y=3 respectively. Given a fixed point P(2, 3).
Q. If P be orthocentre of ΔABC then equation of side BC is :
View SolutionDifferentiate log sin x w.r.t. √x.
View SolutionDifferentiate log sin x w.r.t. √x.
View SolutionDifferentiation of sin(x2) w.r.t. x is
View SolutionIn ΔABC,AB=AC and D is a point in BC so that BD=CD. Prove that AD bisects ∠BAC.
View Solutionlocus of point of reflection of point (a,0) w.r.t. the line yt=x+at2 is given by:
View SolutionIn figure, ⌢AB≅⌢AC and O is the centre of the circle,Prove that OA is the perpendicualr bisector of BC.
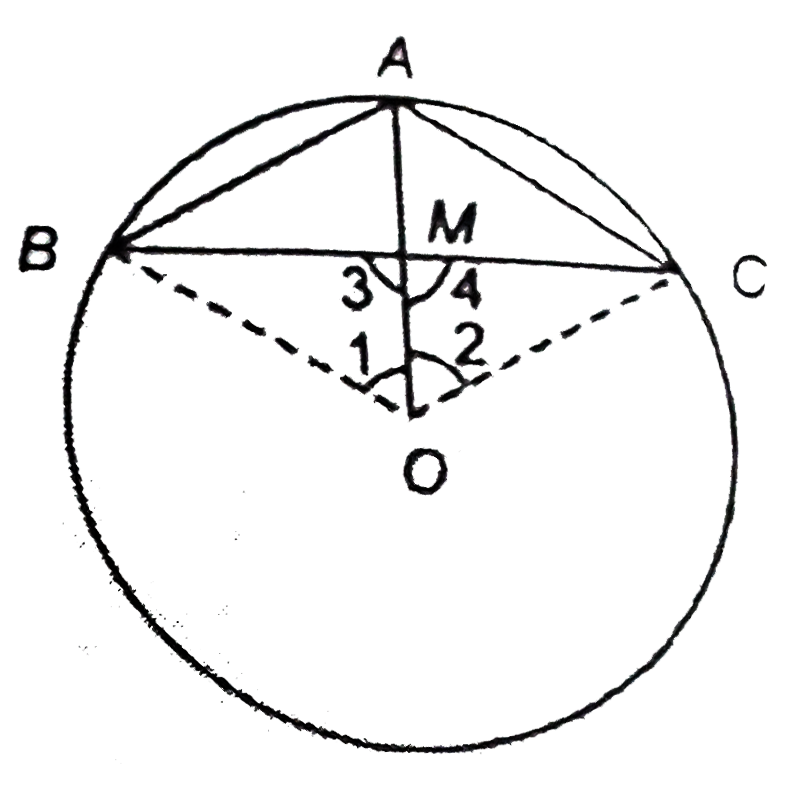
View Solution
