Consider the following table regarding interhalogen compounds, XYn (where Y is more electronegative than X)

Thn according to given information calculate value of expression P2×(P3−P1(Q1+Q2)
The correct Answer is:4
P1=3,P2=5,Q1=2,P3=7,Q2=3
=5(7−3)3+2=4
Topper's Solved these Questions
- CHEMICAL BONDING (BASIC)BOOK - VK JAISWAL ENGLISHCHAPTER - CHEMICAL BONDING (BASIC)EXERCISE - ASSERTION-REASON TYPE QUESTIONS
23 Videos
- CHEMICAL BONDING (ADVANCED)BOOK - VK JAISWAL ENGLISHCHAPTER - CHEMICAL BONDING (ADVANCED)EXERCISE - SUBJECTIVE PROBLEMS
64 Videos
- CO-ORDINATION COMPOUNDSBOOK - VK JAISWAL ENGLISHCHAPTER - CO-ORDINATION COMPOUNDSEXERCISE - LEVEL 2
144 Videos
Similar Questions
Knowledge Check
Solve the following questions on the basis of the following questions on the basis of following functions
(a) P(n+1)=P(n)−P(n−1),P is the term of the sequence and P(0)=0,P(1)=1
(b) Q(n+1)=Q(n)+Q(n+1),Q is the term of the sequence and Q(0)=0,Q(1)=1
The value of Q(n−1)+Q(n+1)+L(n−1)+L(n+1) cannot be :A30B75C18D122Solve the following questions on the basis of the following questions on the basis of following functions
(a) P(n+1)=P(n)−P(n−1),P is the term of the sequence and P(0)=0,P(1)=1
(b) Q(n+1)=Q(n)+Q(n+1),Q is the term of the sequence and Q(0)=0,Q(1)=1
Which of the following can be a positive value of Qn+P(n+1)?A55B234C146Dnone of theseSolve the following questions on the basis of the following questions on the basis of following functions
(a) P(n+1)=P(n)−P(n−1),P is the term of the sequence and P(0)=0,P(1)=1
(b) Q(n+1)=Q(n)+Q(n+1),Q is the term of the sequence and Q(0)=0,Q(1)=1
The value of Qn+Pn cannot be :A2B22C55D88
Consider the following table
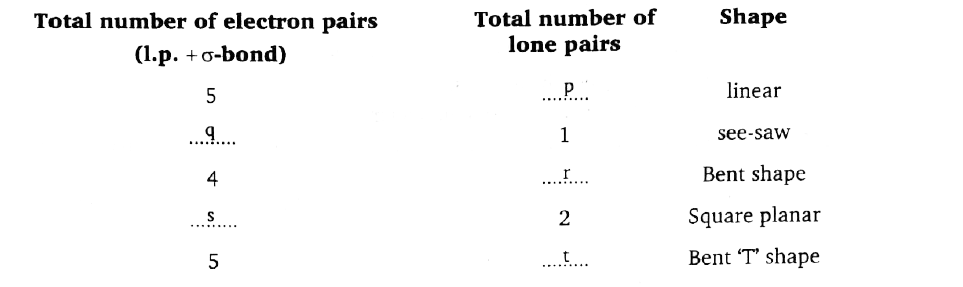
Then calculate value of "p+q+r-s-t".
View SolutionFind the value of :
12p2×4p2q×(−3p)
View SolutionSolve the following questions on the basis of the following questions on the basis of following functions
(a) P(n+1)=P(n)−P(n−1),P is the term of the sequence and P(0)=0,P(1)=1
(b) Q(n+1)=Q(n)+Q(n+1),Q is the term of the sequence and Q(0)=0,Q(1)=1
The value of Q[Q(n+1)] can be :
View SolutionSolve the following questions on the basis of the following questions on the basis of following functions
(a) P(n+1)=P(n)−P(n−1),P is the term of the sequence and P(0)=0,P(1)=1
(b) Q(n+1)=Q(n)+Q(n+1),Q is the term of the sequence and Q(0)=0,Q(1)=1
The value of Qn×Pn can be :
View SolutionSolve the following questions on the basis of the following questions on the basis of following functions
(a) P(n+1)=P(n)−P(n−1),P is the term of the sequence and P(0)=0,P(1)=1
(b) Q(n+1)=Q(n)+Q(n+1),Q is the term of the sequence and Q(0)=0,Q(1)=1
The value of P cannot be :
View Solution
