if value of spin quantum number(s)=−1/2,0,+1/2 then calculate number of groups in the new form of periodic table if all other rules of electronic configurations are remain same.
The correct Answer is:27
To solve the problem of determining the number of groups in the new form of the periodic table with the given spin quantum numbers, we will follow these steps:
Step 1: Understand the Spin Quantum Number
The spin quantum number (s) indicates the orientation of the electron's spin. In the standard model, electrons can have a spin of -1/2 or +1/2. However, in this case, we have three possible values: -1/2, 0, and +1/2.
Step 2: Determine the Electron Filling in Orbitals
In the new configuration, each orbital can accommodate three electrons due to the three possible spin states. This means that for each type of orbital (s, p, d), we will fill the electrons differently.
Step 3: Analyze Each Block of the Periodic Table
1. s-block:
- Normally, the s-block can hold 2 electrons (one for each spin state). With the new configuration, it can hold 3 electrons.
- Number of groups in s-block = 1 (since it can only accommodate one group of s orbitals).
2. p-block:
- The p-block can normally hold 6 electrons (2 per orbital for 3 orbitals). With the new configuration, each p orbital can hold 3 electrons.
- Number of groups in p-block = 3 (since there are 3 p orbitals).
3. d-block:
- The d-block can normally hold 10 electrons (2 per orbital for 5 orbitals). With the new configuration, each d orbital can hold 3 electrons.
- Number of groups in d-block = 5 (since there are 5 d orbitals).
Step 4: Calculate Total Number of Groups
Now, we can sum the number of groups from each block:
- s-block: 1 group
- p-block: 3 groups
- d-block: 5 groups
Total groups = 1 (s-block) + 3 (p-block) + 5 (d-block) = 9 groups.
Conclusion
The total number of groups in the new form of the periodic table, considering the spin quantum number values of -1/2, 0, and +1/2, is 9 groups.
---
The spin quantum number (s) indicates the orientation of the electron's spin. In the standard model, electrons can have a spin of -1/2 or +1/2. However, in this case, we have three possible values: -1/2, 0, and +1/2.
In the new configuration, each orbital can accommodate three electrons due to the three possible spin states. This means that for each type of orbital (s, p, d), we will fill the electrons differently.
1. s-block:
- Normally, the s-block can hold 2 electrons (one for each spin state). With the new configuration, it can hold 3 electrons.
- Number of groups in s-block = 1 (since it can only accommodate one group of s orbitals).
- The p-block can normally hold 6 electrons (2 per orbital for 3 orbitals). With the new configuration, each p orbital can hold 3 electrons.
- Number of groups in p-block = 3 (since there are 3 p orbitals).
- The d-block can normally hold 10 electrons (2 per orbital for 5 orbitals). With the new configuration, each d orbital can hold 3 electrons.
- Number of groups in d-block = 5 (since there are 5 d orbitals).
Now, we can sum the number of groups from each block:
- s-block: 1 group
- p-block: 3 groups
- d-block: 5 groups
The total number of groups in the new form of the periodic table, considering the spin quantum number values of -1/2, 0, and +1/2, is 9 groups.
Topper's Solved these Questions
- PERIODIC PROPERTIESBOOK - VK JAISWAL ENGLISHCHAPTER - PERIODIC PROPERTIESEXERCISE - Level 2
102 Videos
- PERIODIC PROPERTIESBOOK - VK JAISWAL ENGLISHCHAPTER - PERIODIC PROPERTIESEXERCISE - MATCH THE COLUMN
11 Videos
- PERIODIC PROPERTIESBOOK - VK JAISWAL ENGLISHCHAPTER - PERIODIC PROPERTIESEXERCISE - ASSERTION-REASON TYPE QUESTIONS
36 Videos
- p-BLOCK ELEMENTSBOOK - VK JAISWAL ENGLISHCHAPTER - p-BLOCK ELEMENTSEXERCISE - SUBJECTIVE PROBLEMS
35 Videos
- QUALITATIVE INORGANIC ANALYSISBOOK - VK JAISWAL ENGLISHCHAPTER - QUALITATIVE INORGANIC ANALYSISEXERCISE - SUBJECTIVE PROBLEMS
4 Videos
Similar Questions
A compound contains atom A, B and C . The oxidation number of A is +2, of B is +5 and C is -2. The possible formula of the compound is
View Solution
View SolutionAssertion: In CsF, salt, size of Cs+ is slight higher than size of F−.
Reason: Cs+ is largest monoatomic cation and F− is smallest anion.
View SolutionIn phosphorus acid, If X is number of non-bonding electron pairs. Y is number of σ-bonds and Z is number of π-bonds. Then calculate value of "YxZ-X".
View SolutionConsider the following reaction
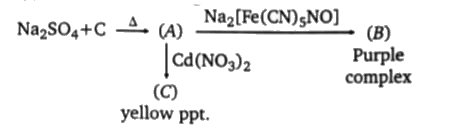
Then calculate value of ∣∣X2−Y2∣∣ (where X and Y are total number of electrons present t_(2g) and e_(g) orbitals respectively in d-block metal ion of compound B).
View SolutionWhat is the value of the spin quantum number of the last electron e^(9) configuration ?
View SolutionAn electron has spin quantum number (s) + 1//2 and magnetic quantum number is 1 it can be person in
View SolutionThe number of 2p electrons having spin quantum number s =- 1//2 are
View SolutionIF sping quantum number have the values +1/2,0 and -1/2 but all other quantum number have values as they have, then the maximum number of electrons in 5th orbit should be
View SolutionIf there are three possible values (-1//2,0,+1//2) for the spin quantum, then electronic configuration of K (19) will be:
View SolutionIf an electron has spin quantum number value is +12 and magnetic quantum number value is −1, then it cannot be present in
View SolutionThe maximum number of electrons with spin value +1/2 in the orbital with azimuthal quantum number value 1=2 and magnetic quantum number m=+1 is...
View SolutionThe number of 2p electrons having spin quantum number s = -1//2 are
View Solutionif value of spin quantum number (s)=-1//2,0,+1//2 then calculate number of groups in the new form of periodic table if all other rules of electronic configurations are remain same.
View SolutionIf spin quantum number S of an electron of an atom has three values -1,0,+1. Then 19K will be the element of which block of periodic table?
View Solution
