Let f(x)=2|x|−1x−3
Range of the values of 'k' for which f(x)=k has exactly two distinct solutions:
(−2,13)
(−2,1]
(0,23]
(−∞,−2)
The correct Answer is:A
To find the range of values of k for which the equation f(x)=k has exactly two distinct solutions, we start with the function defined as:
f(x)=2|x|−1x−3
Step 1: Analyze the function for different cases of x
Since f(x) involves the absolute value function, we need to consider two cases based on the sign of x.
Case 1: x≥0
For x≥0, we have |x|=x. Thus, the function simplifies to:
f(x)=2x−1x−3
Case 2: x<0
For x<0, we have |x|=−x. Thus, the function simplifies to:
f(x)=−2x−1x−3
Step 2: Set up the equations for both cases
We need to find the values of k such that f(x)=k has exactly two distinct solutions.
For Case 1: Setting f(x)=k:
2x−1x−3=k
Cross-multiplying gives:
2x−1=k(x−3)⟹2x−1=kx−3k
Rearranging this leads to:
(2−k)x=−3k+1⟹x=−3k+12−k
For Case 2: Setting f(x)=k:
−2x−1x−3=k
Cross-multiplying gives:
−2x−1=k(x−3)⟹−2x−1=kx−3k
Rearranging this leads to:
(−2−k)x=−3k+1⟹x=−3k+1−2−k
Step 3: Determine the conditions for distinct solutions
For f(x)=k to have exactly two distinct solutions, one solution must come from each case. This means we need to ensure that the two expressions for x yield valid solutions.
1. From Case 1: x=−3k+12−k must be non-negative.
2. From Case 2: x=−3k+1−2−k must be negative.
Step 4: Solve the inequalities
For Case 1: −3k+12−k≥0
This occurs when:
- The numerator and denominator are both positive, or both negative.
1. Numerator: −3k+1≥0⟹k≤13
2. Denominator: 2−k>0⟹k<2
For Case 2: −3k+1−2−k<0
This occurs when:
- The numerator is positive and the denominator is negative.
1. Numerator: −3k+1>0⟹k<13
2. Denominator: −2−k<0⟹k>−2
Step 5: Combine the results
From the inequalities, we have:
1. From Case 1: k≤13 and k<2
2. From Case 2: k<13 and k>−2
Combining these gives:
−2<k<13
Conclusion
The range of values of k for which f(x)=k has exactly two distinct solutions is:
(−2,13)
2. From Case 2: x=−3k+1−2−k must be negative.
2. Denominator: 2−k>0⟹k<2
2. Denominator: −2−k<0⟹k>−2
2. From Case 2: k<13 and k>−2
Topper's Solved these Questions
- FUNCTION BOOK - VK JAISWAL ENGLISHCHAPTER - FUNCTION EXERCISE - MATCHING TYPE PROBLEMS
6 Videos
- FUNCTION BOOK - VK JAISWAL ENGLISHCHAPTER - FUNCTION EXERCISE - SUBJECTIVE TYPE PROBLEMS
36 Videos
- FUNCTION BOOK - VK JAISWAL ENGLISHCHAPTER - FUNCTION EXERCISE - ONE OR MORE THAN ONE ANSWER IS/ARE CORRECT
23 Videos
- ELLIPSE BOOK - VK JAISWAL ENGLISHCHAPTER - ELLIPSE EXERCISE - Exercise-4 : Subjective Type Problems
2 Videos
- HYPERBOLABOOK - VK JAISWAL ENGLISHCHAPTER - HYPERBOLAEXERCISE - Exercise-4 : Subjective Type Problems
3 Videos
Similar Questions
Knowledge Check
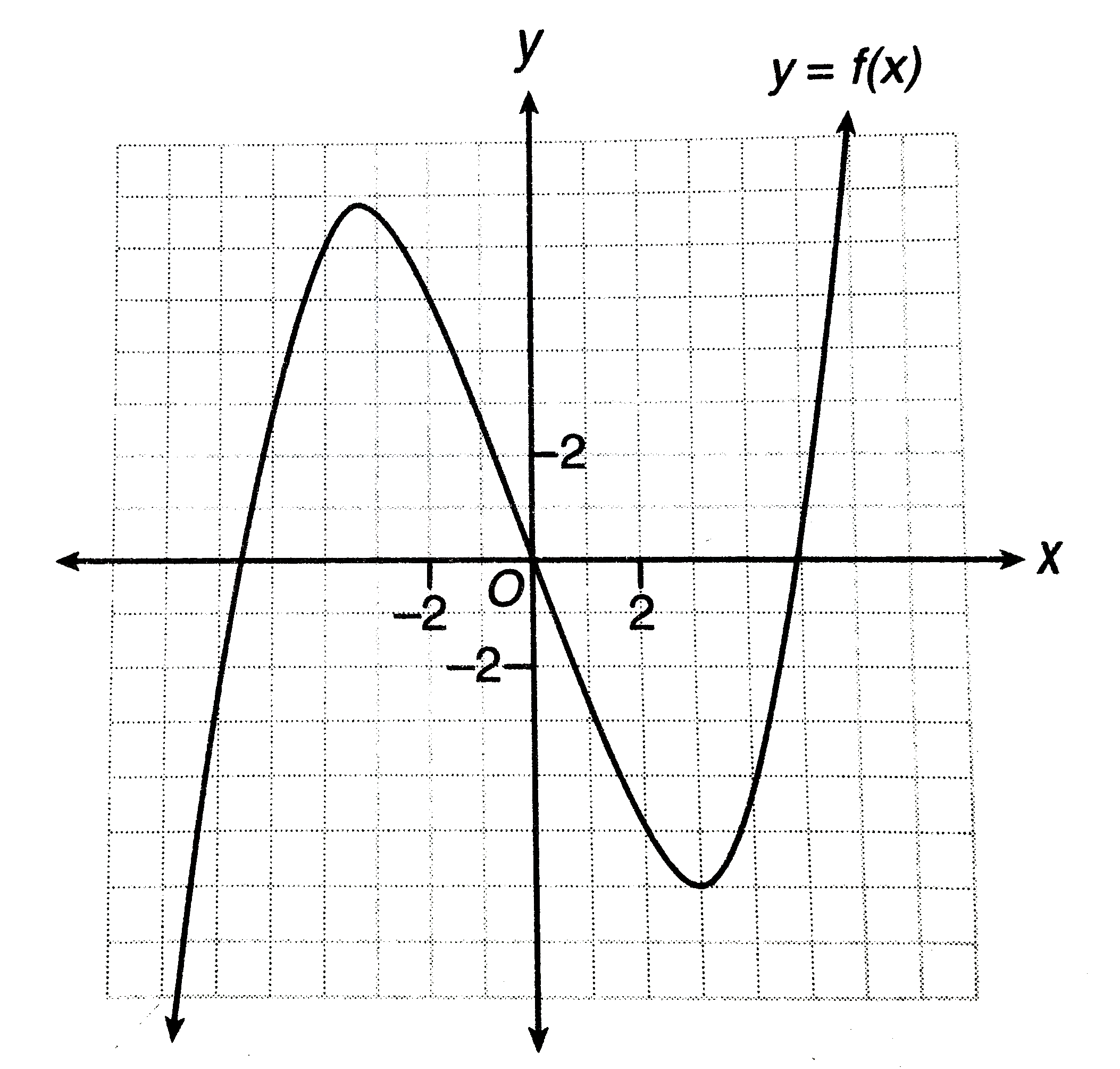
16-18 The graph of polynomial function f is shown above.
Q.What is the greatest integer value of k for which f(x)=k has exactly 3 real solutions?A−5B0C6D7
Let f(x)=sin−1x−cos−1 x, then the set of values of k for which of |f(x)|=k has exactly two distinct solutions is :
View SolutionMinimum integral value of k for which the equation ex=kx2 has exactly three real distinct solution,
View SolutionFind the minimum integral value of k for which the equation ex=kx2 has exactly three real distinct solutions.
View SolutionIf |x−1|+|x−2|+|x−3|=k,xεR then value of k for which given equation has exactly one solution.
View SolutionFind the values of k for which f(x)=kx3−9kx2+9x+3 is increasing on R .
View SolutionLet f(x)=x+2|x+1|+2|x−1|. Find the values of k if f(x)=k
(i) has exactly one real solution,
(ii) has two negative solutions,
(iii) has two solutions of opposite sign.
View SolutionLet f(x)=x+5√x2+1, then the smallest integral value of k for which f(x)≤k∀x∈Ris
View Solution
