The acceleration a in ms−2 of a particle is given by a=3t2+2t+2, where t is the time. If the particle starts out with a velocity v=2ms−1 at t=0, then find the velocity at the end of 2s.
To find the velocity of the particle at the end of 2 seconds given the acceleration function, we can follow these steps:
Step 1: Write down the acceleration function
The acceleration a is given by:
a=3t2+2t+2
Step 2: Relate acceleration to velocity
Acceleration is the rate of change of velocity with respect to time. Therefore, we can express this relationship as:
a=dvdt
This implies:
dv=adt
Step 3: Integrate the acceleration to find velocity
We need to integrate the acceleration function to find the velocity function v(t):
v(t)=∫adt=∫(3t2+2t+2)dt
Step 4: Perform the integration
Integrating term by term:
v(t)=∫3t2dt+∫2tdt+∫2dt
=t3+t2+2t+C
where C is the constant of integration.
Step 5: Use initial conditions to find the constant
We know that the initial velocity v(0)=2m/s at t=0:
v(0)=03+02+2⋅0+C=C
Thus, C=2. Therefore, the velocity function becomes:
v(t)=t3+t2+2t+2
Step 6: Calculate the velocity at t=2s
Now, we substitute t=2 into the velocity function:
v(2)=(2)3+(2)2+2⋅2+2
=8+4+4+2
=18m/s
Final Answer
The velocity of the particle at the end of 2 seconds is:
18m/s
The acceleration a is given by:
a=3t2+2t+2
Acceleration is the rate of change of velocity with respect to time. Therefore, we can express this relationship as:
a=dvdt
This implies:
dv=adt
We need to integrate the acceleration function to find the velocity function v(t):
v(t)=∫adt=∫(3t2+2t+2)dt
Integrating term by term:
v(t)=∫3t2dt+∫2tdt+∫2dt
=t3+t2+2t+C
where C is the constant of integration.
We know that the initial velocity v(0)=2m/s at t=0:
v(0)=03+02+2⋅0+C=C
Thus, C=2. Therefore, the velocity function becomes:
v(t)=t3+t2+2t+2
Now, we substitute t=2 into the velocity function:
v(2)=(2)3+(2)2+2⋅2+2
=8+4+4+2
=18m/s
The velocity of the particle at the end of 2 seconds is:
18m/s
Topper's Solved these Questions
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.5
2 Videos
- ARCHIVES 2 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - Integer
4 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
Similar Questions
The acceleration a of a particle in ms2 is give by a=18t2+36t+120. If the particle starts from rest, then velocity at end of 1 s is
View SolutionThe velocity of a particle is given by v=12+3(t+7t2). What is the acceleration of the particle?
View SolutionIf acceleration of a particle at any time is given by a=2t+5
Calculate the velocity after 5 s, if it starts from rest
View SolutionThe velocity of a particle is given by v=(2t2−4t+3)m/s where t is time in seconds. Find its acceleration at t=2 second.
View SolutionThe velocity of a particle is given by v=(4t2−4t+3)m/s where t is time in seconds. Find its acceleration at t=1 second.
View SolutionThe acceleration of a particle is given by a=t3−3t2+5, where a is in ms−2 and t is in second. At t=1s, the displacement and velocity are 8.30m and 6.25ms−1, respectively. Calculate the displacement and velocity at t=2s.
View SolutionAcceleration of a particle is given by
a=3t2+2t+1
Where t is time . Find
(i) its velocity at time t assuming velocity to be 10 m/s at t = 0.
(ii) its position at time t assuming that the particle is at origin at t = 0.
(iii) What is the average speed of the particle in the duration t = 0 to t = 1s?
(iv) What is the average acceleration of the particle in the duration t = 0 to t = 1s?
View SolutionVelocity of a particle is given as v=(2t2−3)m/s. The acceleration of particle at t = 3s will be :
View SolutionThe acceleration time graph of a particle is shown in the Fig. 2 (CF). 11. At time t=0s velocity of the particle is 8ms−1. Its velocity t=10s is.
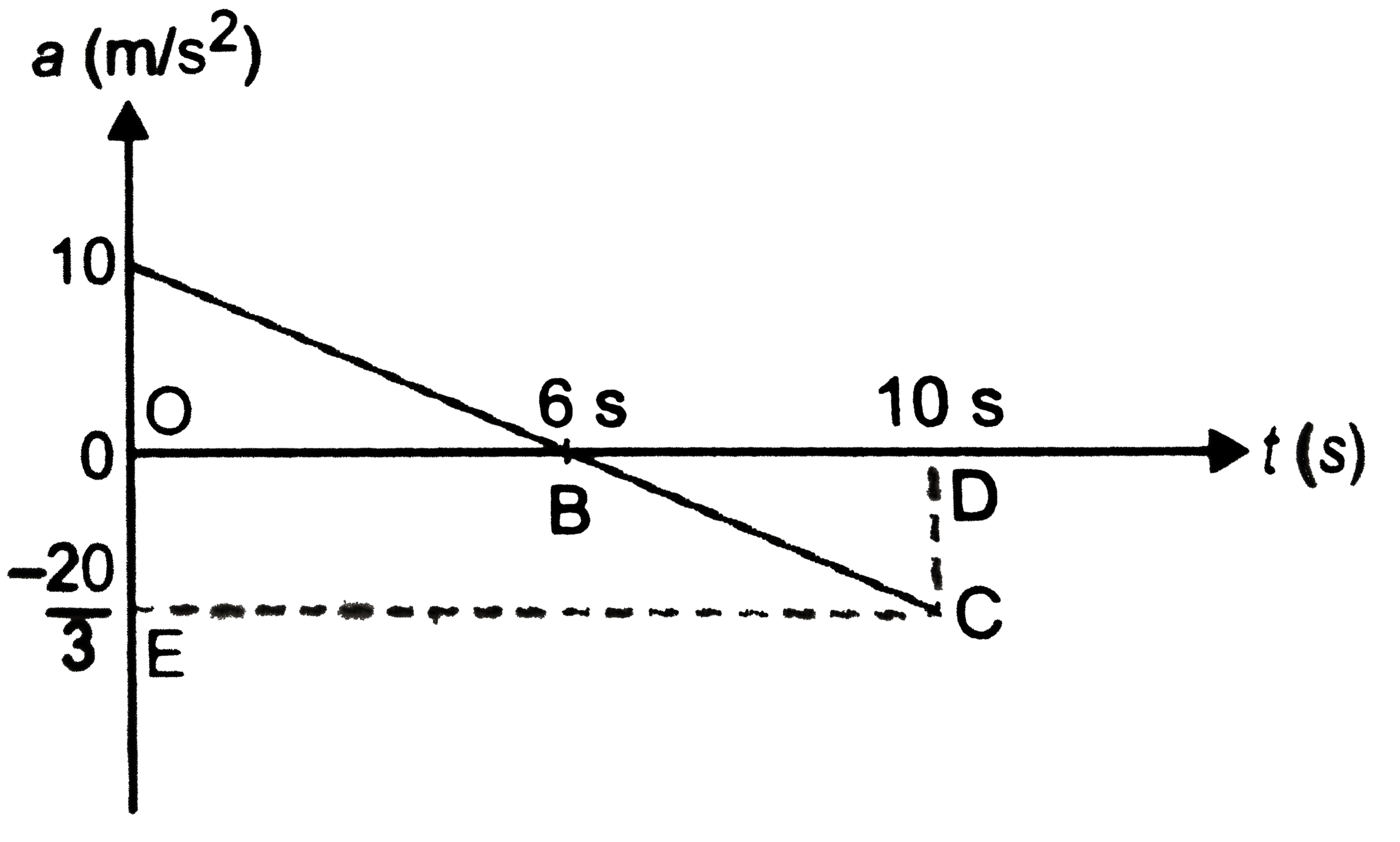 .
.
View SolutionAcceleration of a particle is given by
a=1/V
Where V is the velocity . Find
(i) Velocity of particle at time t, given that v = 10 m/s at t = 0.
(ii) Velocity of particle when its position is x, given that speed is 3 m/s at x = 0.
View Solution
