A ball (A) is thrown straight up from the edge of the roof of a building. Another ball (B) is dropped from the roof $1.00 s$ later. You may ignore air resistance . (a) If the height of the building is $20.0 m$ , what must the initial speed of ball A be if both are to hit the ground at the same time? (b) On the same graph sketch the position and velocity of each balls a function of time, measured from when the first ball is thrown and taken origin at ground.
.

Video Solution

Text Solution

Verified by Experts

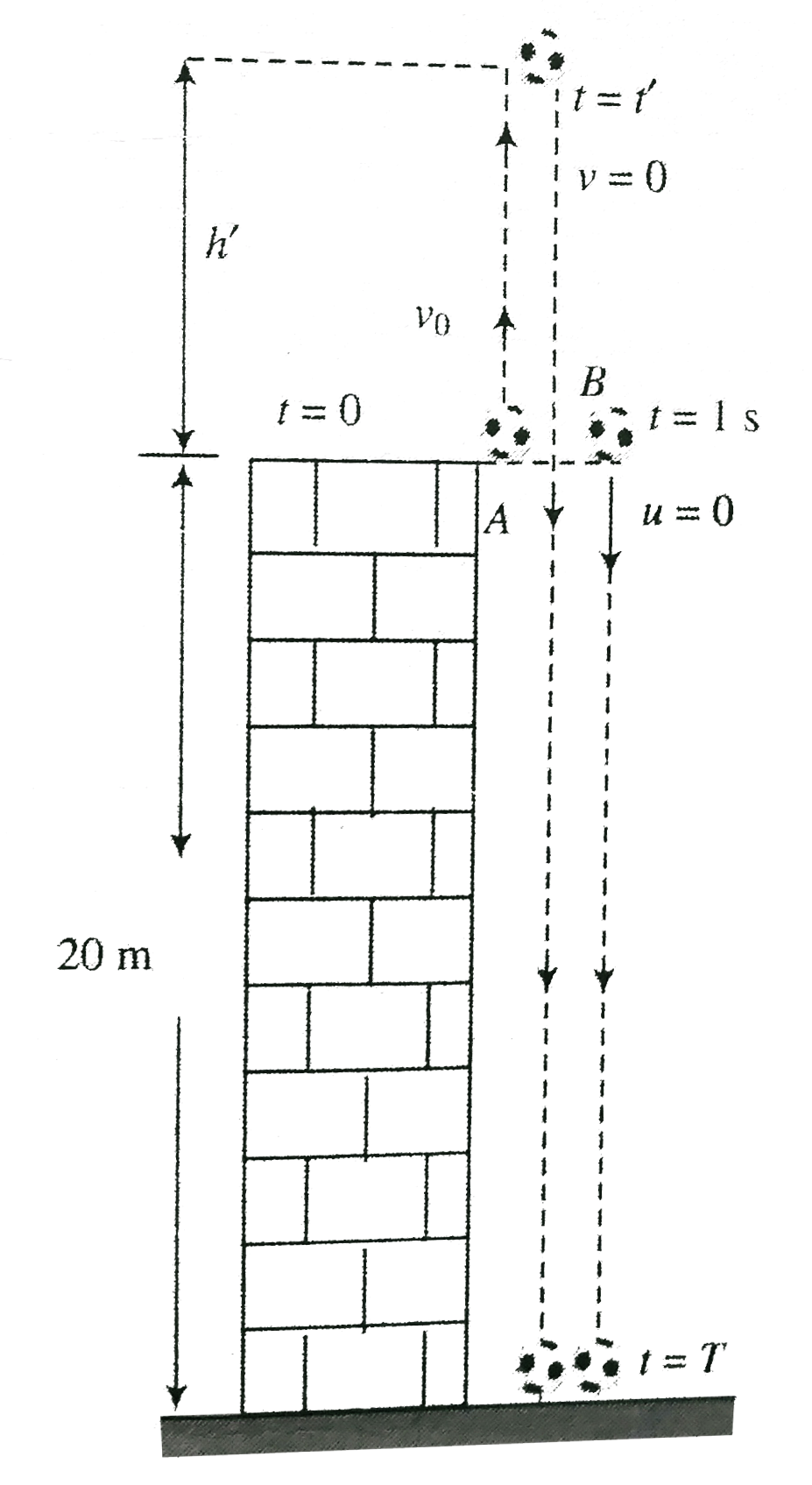For the purpose of doing all four farts with the least reperition of algebra, quantities will be dented symbolically. That is, let
$y_{1} = h + v_{0} t - \frac{1}{2} g t^{1}$ and $y_{2} = h - \frac{1}{2} {g (t - t_{0})}^{2}$ .
In this case, $t_{0} = 1.00 s$ . Setting $y_{1} = y_{2} = 0$ , expanding the binomial ${(t - t_{0})}^{2}$ , which can be solved for $t$ ,
$t = \frac{\frac{1}{2} g t_{0}^{2}}{g t_{0} - v_{0}} = \frac{t_{0}}{2} \frac{1}{(1 - \frac{v_{0}}{g t_{0}})}$ Substiuting this into the expressinon for $y_{1}$ and setting $y_{1} = 0$ and solving for $h$ as a function of $v_{0}$ yields after some algebra,
$h = \frac{1}{2} g t_{0}^{2} \frac{{(\frac{1}{2} g t_{0} - v_{0})}^{2}}{{(g t_{0} - v_{0})}^{2}}$
a. Using the given value, $t_{0} = 1.00 s$ and $g = 9.80 m s^{- 2}$ .
$h = 20.0 m = (4.9 m) (\frac{4.9 m s^{- 1} - v_{0}}{9.8 m s^{- 2} - v_{0}})$
This has two solutions, one of which is not physical [the first ball is still going up when the second is released, see part $C$ ]. The physical solution invlves taking the negative square root before solving for $v_{0}$ , and yields $8.2 m s^{- 1}$
.
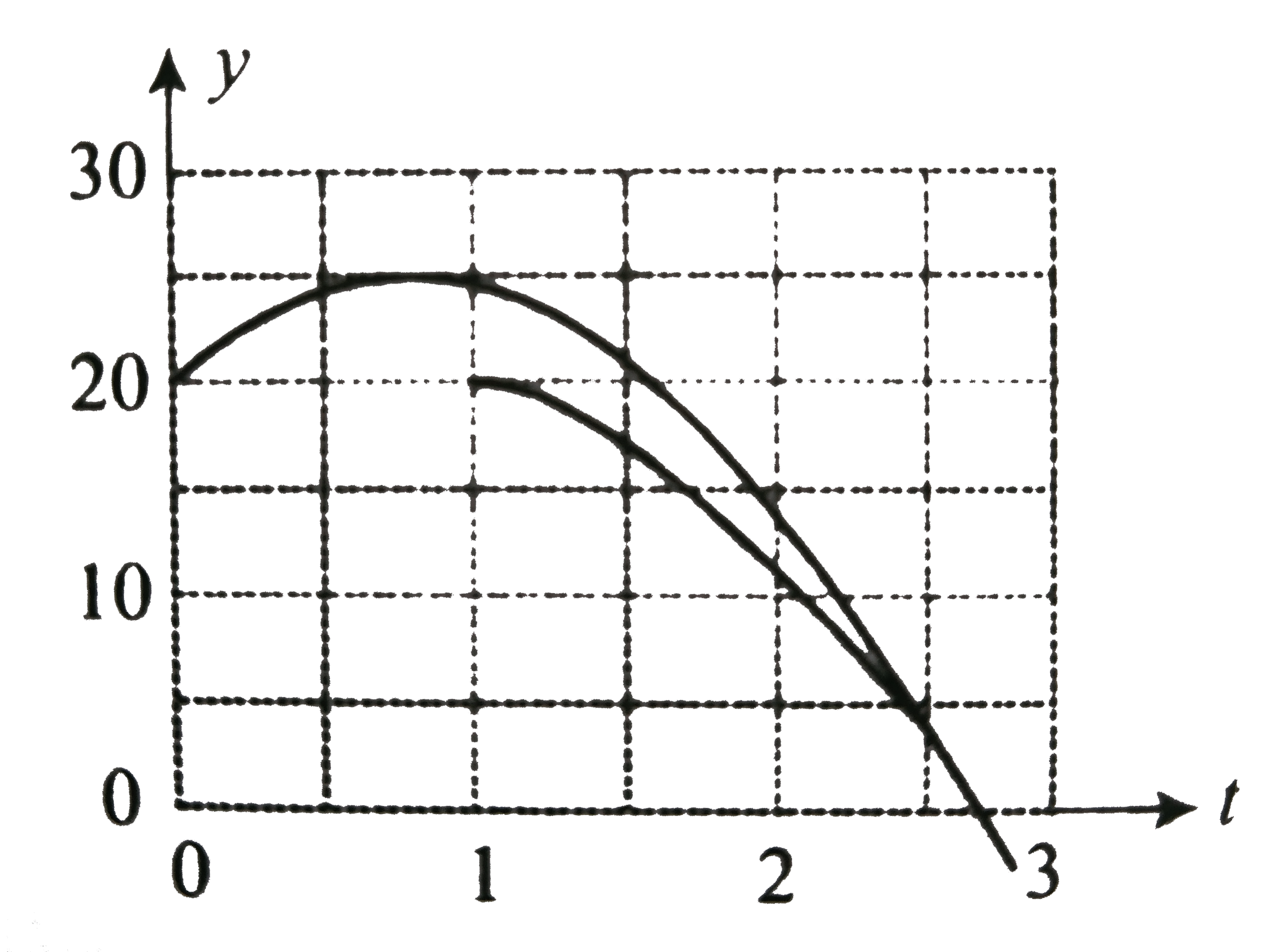b. The above expression gives
for (i) $0.411 m$ and for (ii) $1.15 k m$ .
C. As $v_{0}$ approaches $9.8 m s^{- 1}$ , the height $h$ becomes infinite corresponding to a relative velocity at the second ball is throun that approaches zero, If $v_{0} \leq 9.8 m s^{- 1}$ , the first ball can never catch the second ball.
d. As $v_{0}$ approaches $4.9 m s^{- 1}$ the height approaches zero. This corresponds to the first ball being closer and is released. If $v_{0} \leq 4.9 m s^{- 1}$ , the first ball will already have roof on the wau down nefore the second ball is released, and the second ball can catch up.

|

Updated on:21/07/2023

Class 11 PHYSICS RECTILINEAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A ball $A$ is thrown up vertically with a speed $u$ and at the same instant another ball $B$ is released from a height $h$ . At time $t$ , the speed $A$ relative to $B$ is
Au
Bu - 2gt
C $\sqrt{u^{2} - 2 g h}$
Du - gt

CENGAGE PHYSICS ENGLISH-KINEMATICS-1-Exercise 4.2

Home
Ask Doubt
Profile